1*Dep.
«Hydraulics and Water Supply», Dnipro National University of
Railway Transport named
after Academician V. Lazaryan, Lazaryana St.,
2, Dnipro, Ukraine, 49010, tel. +38 (056) 273 15 09,
e-mail
water.supply.treatment@gmail.com,ORCID
0000-0002-1531-7882
2*Dep.
«Hydraulics and Water Supply», Dnipro National University of
Railway Transport named
after Academician V. Lazaryan, Lazaryana St.,
2, Dnipro, Ukraine, 49010, tel. +38 (056) 273 15 09,
e-mail
water.supply.treatment@gmail.com, ORCID
0000-0002-1230-8040
3*Dep.
«Fluid Dynamics, Energy and Mass Transfer», Oles Honchar Dnipro
National University,
Haharina Av., 72, Dnipro, Ukraine, 49000, tel.
+38 (056) 374 98 22,
e-mail water.supply.treatment@gmail.com,
ORCID 0000-0003-2399-3124
4*Dep
«Hydraulics
and Water Supply», Dnipro National University of Railway Transport
named after Academician V. Lazaryan, Lazaryana St., 2, Dnipro,
Ukraine, 49010,
tel. +38 (056) 273 15 09, e-mail
gidro_eko@ukr.net, ORCID 0000-0003-3057-9204
5*Dep.
«Physics», Ukrainian State University of Chemical Technology,
Haharina Av., 8, Dnipro,
Ukraine, 49005,tel. +38 (056) 753 56 38,
e-mail physics@udhtu.edu.ua, ORCID 0000-0002-9893-3479
COMPUTER SIMULATION OF BIOLOGICAL WASTEWATER
TREATMENT PROCESSES IN AEROTanks WITH plates
Purpose. Efficiency determination of the aeration
tank at the stage of design or reconstruction of bioreactors in which
biological wastewater treatment is carried out requires the use of
special mathematical models and calculation methods. The main purpose
of the article is to develop CFD models for evaluating the operation
efficiency of aeration tanks. Methodology. A numerical model
has been developed for the computer calculation of the biological
wastewater treatment process in aerotanks, taking into account
hydrodynamics. The model is based on two-level mass conservation
equations for the substrate and activated sludge and the velocity
potential equation. The process of biological transformation of the
substrate is calculated based on the Monod model. For the numerical
integration of the mass transfer equations of activated sludge and
substrate, the alternating-triangular
difference splitting scheme is used. In this case, the basic
equations are divided into two equations of a more simplified form.
For the numerical integration of the equations for the velocity
potential, it is split into two one-dimensional equations. Further,
each equation is solved according to explicit scheme. For the
numerical integration of equations that describe the process of
substrate transformation based on the Monod model, the Euler method
is used. Findings. The software implementation of the
constructed numerical model has been carried out. The results of a
computational experiment on the study of the wastewater treatment
process in an aeration tank with plates are presented. This leads to
the conclusion that the quality control of wastewater treatment
in aeration tanks is possible with the help of plates. Originality.
A multivariate CFD model has been developed, which makes it possible
to quickly assess the efficiency of the aeration tank. A feature of
the model is the ability to evaluate the operation of the aeration
tank, taking into account its geometric shape and location of
additional plates in the construction. Practical value. The
constructed numerical model can be used during calculations in the
case of designing aeration tanks, or in determining the efficiency of
wastewater treatment under new operating conditions.
Keywords:
water purification; numerical modeling;
aerotank; model Mono; CFD models; biological cleaning; wastewater;
activated sludge; concentration of pollutants
Introduction
Biological treatment is one of
the most effective methods of wastewater treatment [3, 4, 6].
Efficiency determination of this treatment at the stage of design or
reconstruction of bioreactors, in which this method of wastewater
treatment is carried out, requires the use of special mathematical
models and calculation methods. Moreover, these theoretical
calculation methods are the main toolkit, since a physical
experiment in the field of biological treatment always requires a
long time and expensive equipment. To date, a significant number of
mathematical models have been developed that allow, with different
approximation degrees, determining the bioreactor efficiency. But
the existing mathematical models (empirical, balance, analytical)
[1–5, 7–17] do not take into account a number of important
parameters affecting the efficiency of bioreactors (their geometric
shapes and design features, movement hydrodynamics activated sludge
and substrate in them, the presence of additional elements, various
modes of operation), or require significant time when implemented on
computers (CFD-models). Therefore, the development of mathematical
models for assessing the efficiency of biological reactors, which
allow taking into account these important factors and quickly
determining the values of the parameters necessary for the designer,
is an important scientific task.
Purpose
This work
provides for the development of a numerical model to assess the
efficiency of wastewater treatment in aerotanks. The task is to
create a multifactorial computer model that makes it possible to
quickly calculate the process of
biological wastewater treatment, taking into account the geometric
shape of the bioreactor.
Methodology
When building a model, we will
take into account the following factors:
-
geometric shapes of aerotank;
– the process of changing the
substrate concentration in aerotanks over time;
– the process of changing the
of activated sludge concentration in aerotanks over time;
– the presence of additional
elements in aero-tanks.
The material balance equations
for the substrate and activated sludge in the reactor based on the
Monod model has the following form:

where
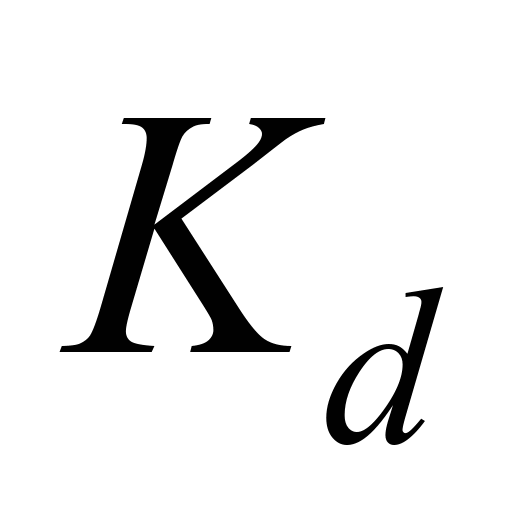 –
activated sludge
measurement coefficient;
–
activated sludge
measurement coefficient;
 – time;
– time;
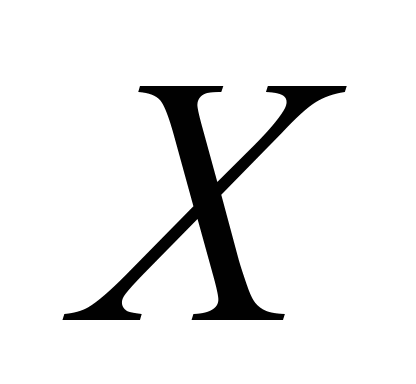 –
averaged concentration
of activated
sludge in the
bioreactor ;
–
averaged concentration
of activated
sludge in the
bioreactor ;
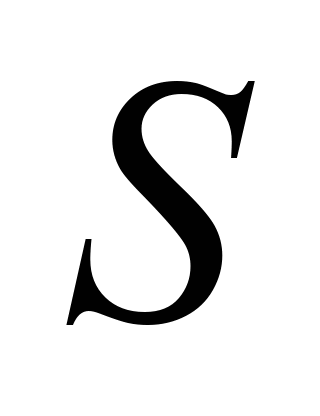 – averaged concentration
of substrate in
the bioreactor;
– averaged concentration
of substrate in
the bioreactor;
 – diffusion coefficient; Y –
parameter in the Monod model;
– diffusion coefficient; Y –
parameter in the Monod model; 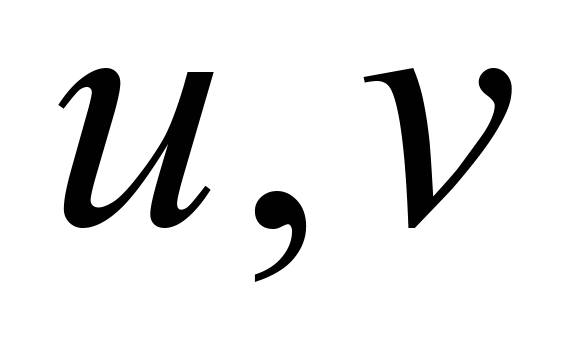 –
components of the water flow velocity in the bioreactor in the
direction of the x, y axes, respectively;
–
components of the water flow velocity in the bioreactor in the
direction of the x, y axes, respectively;
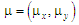 – components of the diffusion coefficients at the considered plane
point in the x, y
direction.
– components of the diffusion coefficients at the considered plane
point in the x, y
direction.
The averaged
concentration of activated sludge and substrate over the width of
the bioreactor is determined as follows:
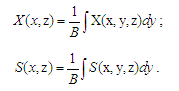
Equations
(1) and (2) describe the change in the concentration of activated
sludge and substrate over time in the aeration tank due to movement
and diffusion. Equations (3) – (5) describe the process of
substrate consumption by activated sludge.
The limiting conditions for
modeling equations are as follows:
at the inlet, the boundary
condition is:
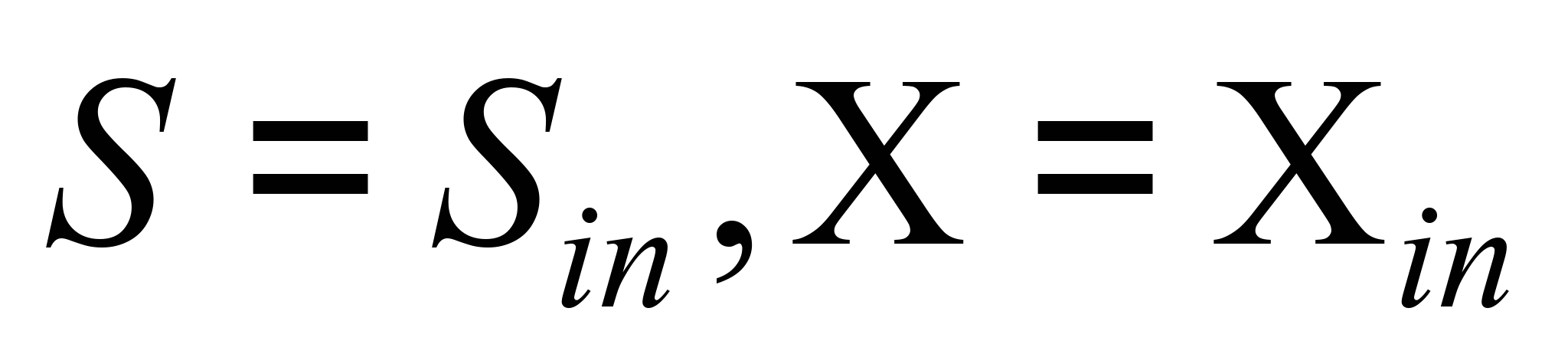 ,
,
where
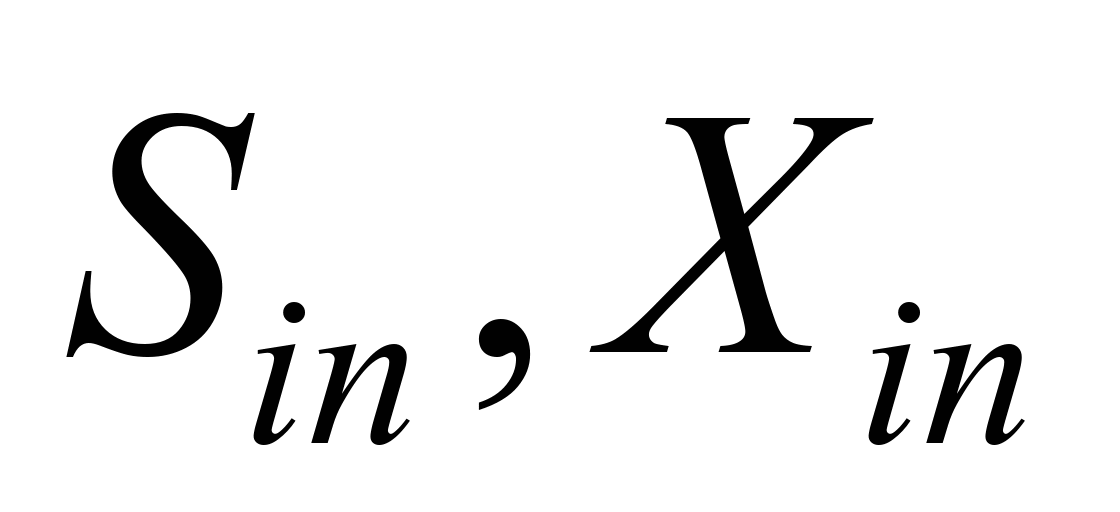 – known concentrations of substrate and sludge, respectively;
– known concentrations of substrate and sludge, respectively;
2) boundary
conditions at the exit from the bio-reactor:
 ;
;
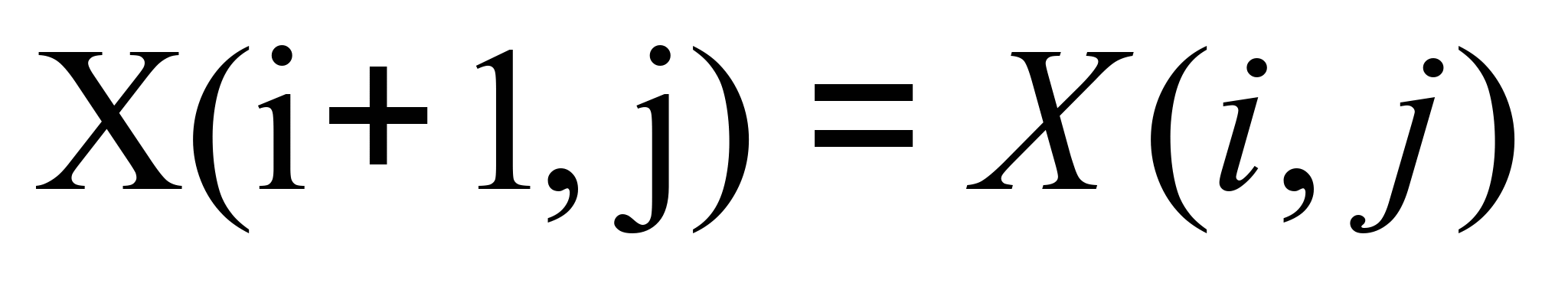 ,
,
where
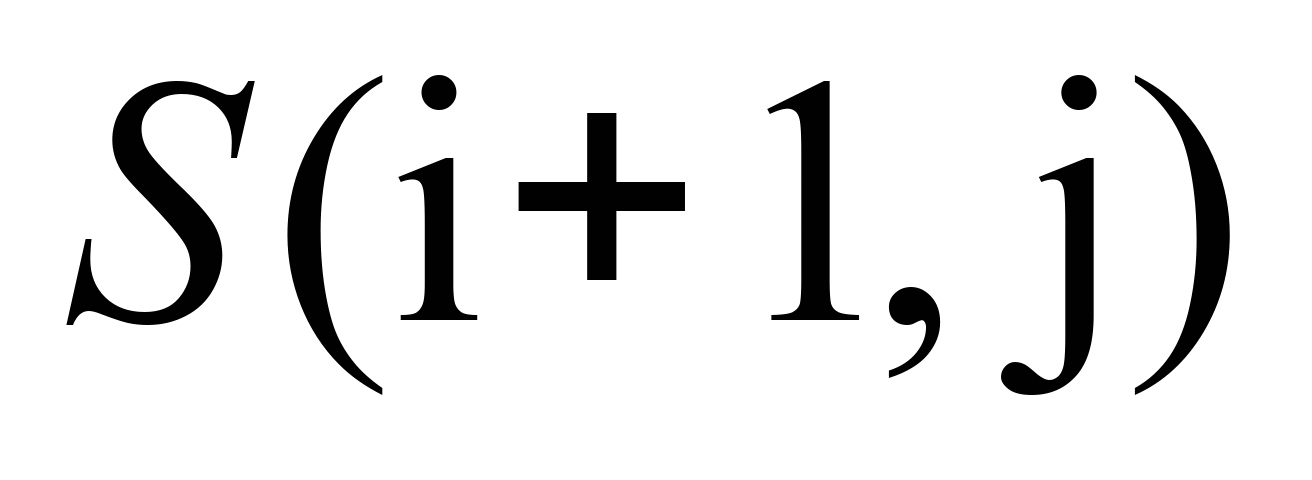 ,
, – concentrations in the last computational cell;
– concentrations in the last computational cell;

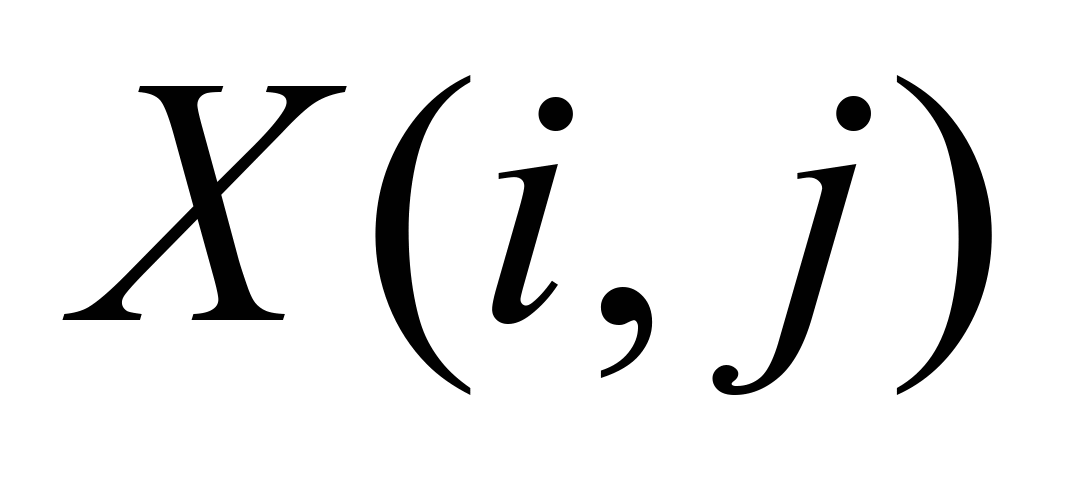 – concentrations in the previous computation cell;
– concentrations in the previous computation cell;
3) on rigid
surface:
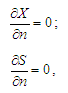
where n
– unit normal to the surface.
The initial
conditions are as follows: at t=0
X=X0,
S=S0.
To solve the hydrodynamics
problem – determining the components field of the flow velocity
vector in the aerotank – a model of potential motion was used [8,
29]:
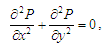 (6)
(6)
where Р
– velocity potential.
Knowing the potential field,
the values of the components of the flow velocity vector in the
bioreactor are determined by the formulas [8, 9, 14]:
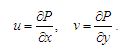
Let us consider the difference
in dependencies, with the help of which the numerical integration of
the modeling equations is carried out. Thus, to calculate the
substrate concentration in the bioreactor, an alternating-triangular
two-step splitting scheme is used [2]. At the first stage of
splitting, the calculated dependence has the form:
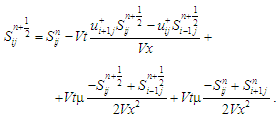 (7)
(7)
At the
second step of splitting, the calculated dependence is as follows:
 (8)
(8)
where
 ,
,
 .
.
For the
second equation, the difference schemes have the form:
– calculated
dependence at the first step:
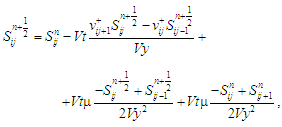 (9)
(9)
– calculated
dependence at the second step:
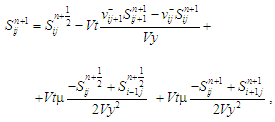 (10)
(10)
where
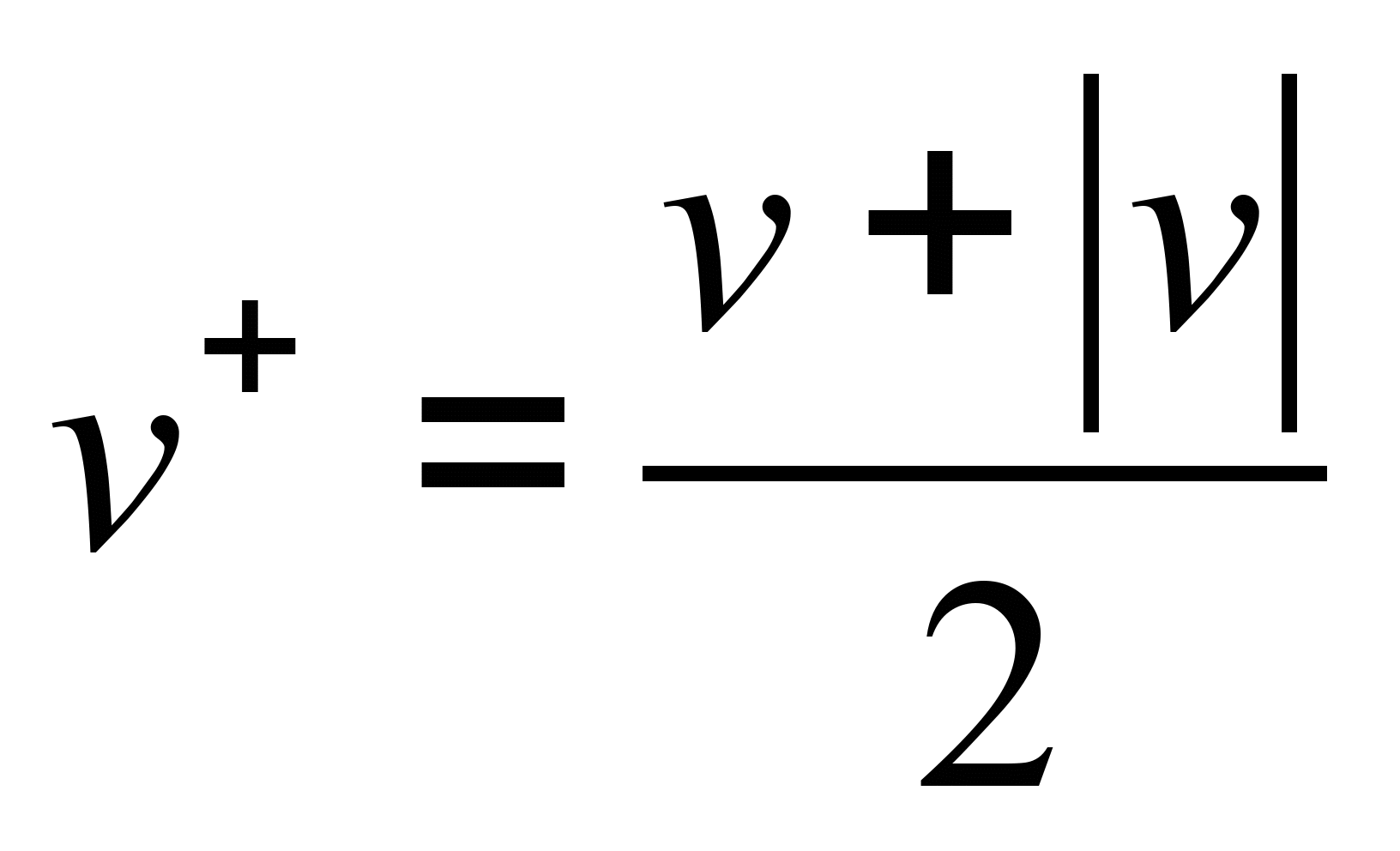 ,
,
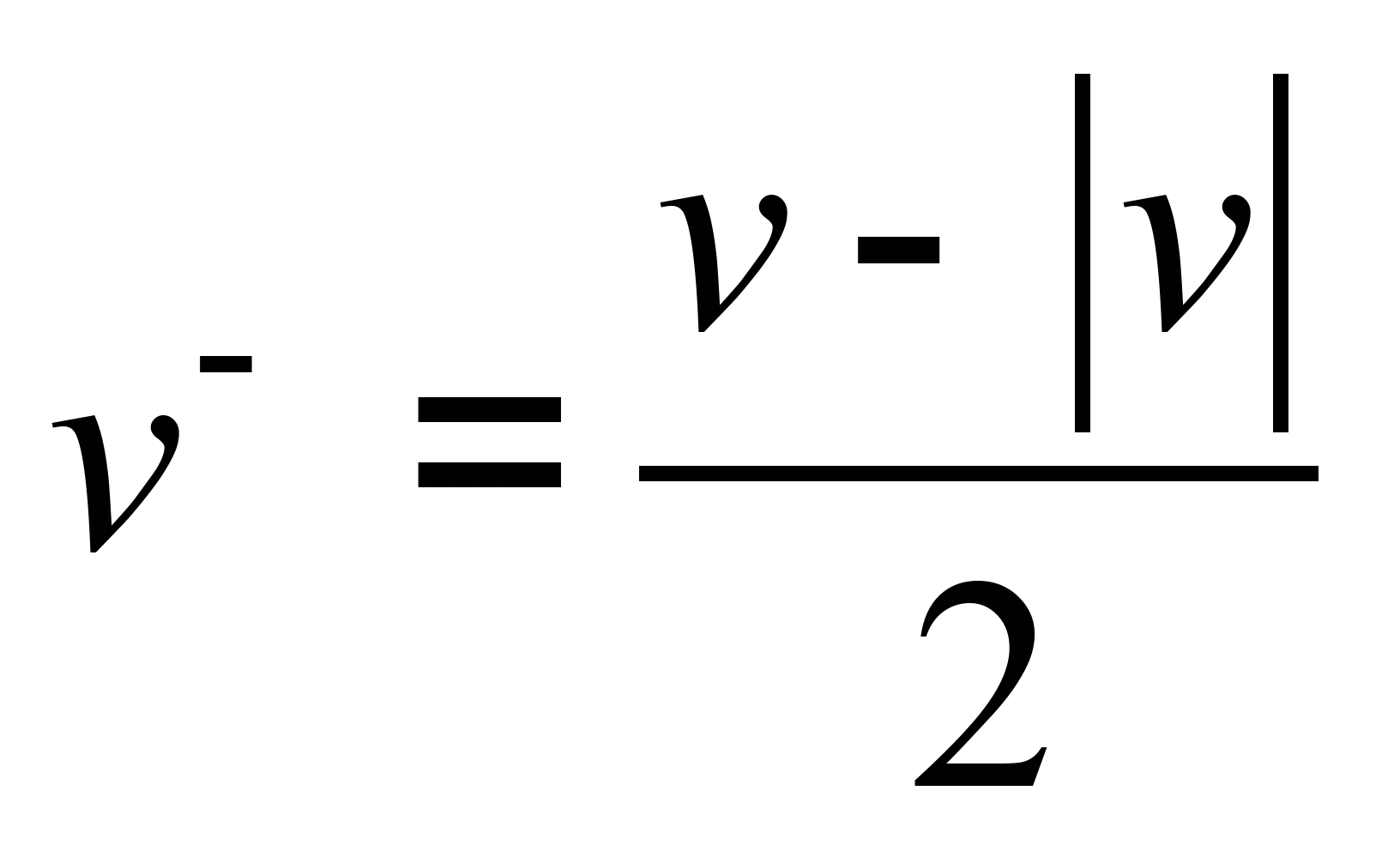 .
.
To construct
a local one-dimensional scheme for solving equation (6), we perform
the following transformations. First, let us represent this equation
in the form:
 (11)
(11)
where t
– fictitious time.
Further, we
divide equation (11) as follows:
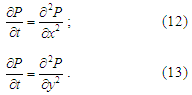
Equation
(12) describes the change in the value of Р
in the direction of the X
axis, and equation (13) describes the change in the Y
direction.
The
calculated dependencies (Richardson's method) for determining the
unknown value of Р based on
equation (12) have the form:
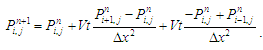 (14)
(14)
Accordingly,
the calculated dependencies (Richardson's method) for determining
the unknown value of Р based on
equation (13):
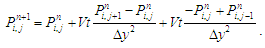 (15)
(15)
Since we solve the evolutionary
equation, the calculation by dependencies (14)–(15) ends when the
following condition is met:
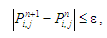
where ε
– small number; n
– iteration number.
We calculate the flow velocity
as follows:
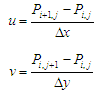
For numerical integration of
equations (3)–(4), the Euler method is used.
The algorithm for solving this
problem includes two main stages.
At the first stage, the
following steps are performed:
1) the
velocity potential field P
(x,
y) in the aerotank is calculated;
2) the flow
velocity field u
(x,
y), v
in the aerotank is determined.
The second
stage (calculation at the time step dt)
contains the following steps:
1) the change in the
concentration of activated sludge in the aerotank due to flow
movement and diffusion is calculated;
2) the change in the
concentration of the substrate in the aerotank due to the flow
movement and diffusion is calculated;
3) the change in the
concentration of activated sludge and substrate in each difference
cell based on the Monod model is calculated;
4) the calculation is repeated
at a new time step, starting from item 1.
Based on the constructed
numerical model, the BIO-2K computer program was developed.
Programming is carried out in the FORTRAN algorithmic language.
Findings
Let us
present the results of solving the problem of assessing the
efficiency of the aeration tank using the developed CFD model. The
following scenarios were considered:
scenario no.
1: the aerotank works without additional elements inside the
structure;
scenario no.
2: aerotank has one plate inside the structure;
scenario no.
3: aerotank has two plates inside the structure;
scenario no.
4: aerotank works as a reservoir for the substrate destruction, but
there is no entry and exit of the substrate and activated sludge.
That is, in this scenario, the aerotank is a tank filled with
activated sludge and substrate, and the process of changing their
concentration was studied using the Monod model.
Calculations
were performed with the following initial data:
Sin
= 360 mg/l – the concentration of
the substrate (Biological oxygen demand (complete), which enters the
structure;
bioreactor dimensions 15x5 m;
 =
3 495 m3/day
– waste water consumption;
=
3 495 m3/day
– waste water consumption;

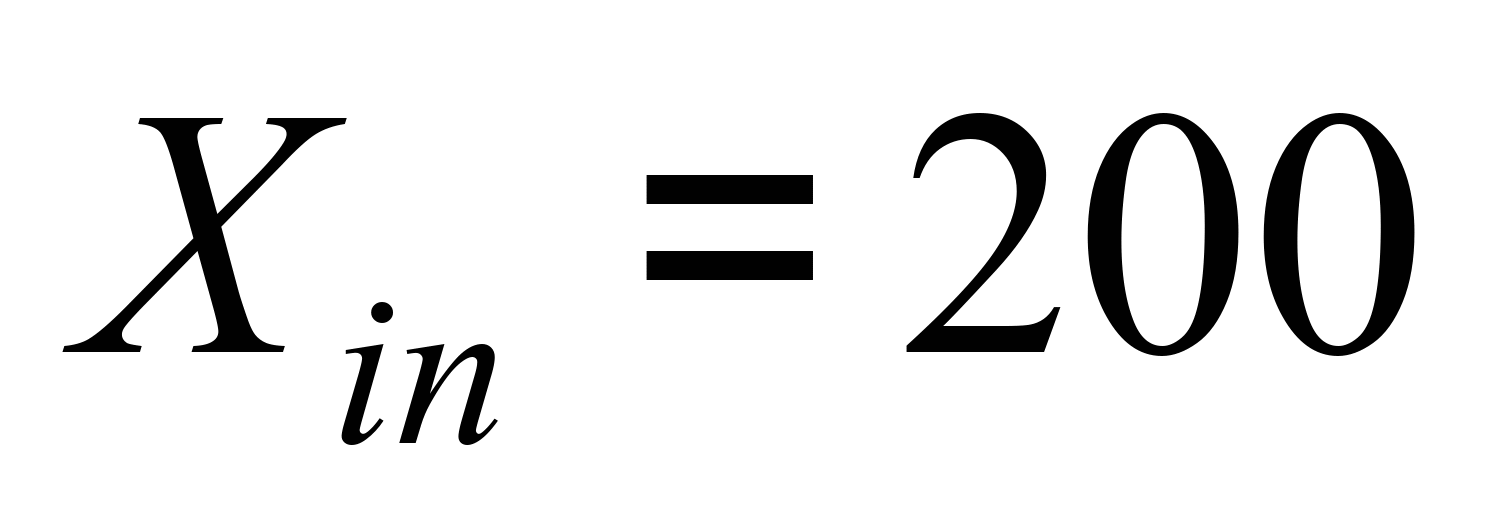 mg/l –
concentration of activated sludge that enters the reactor;
mg/l –
concentration of activated sludge that enters the reactor;
μmax
= 1.04 – reproducibility index parameter;
Ks
= 100 mg/l – sludge index
parameter;
Kd
= 0.055 1/day – theb coefficient that takes into account the death
of microorganisms;
Y = 0.55 – parameter
in the Monod model.
Initial
condition: Sin
= 360 mg/l, Xin
= 200 mg/l, is the concentration of the substrate and activated
sludge in the reactor for the time moment t
= 0.
Figures 1–4
show how the substrate concentration at the reactor outlet changes
for the scenarios under consideration. The concentration is averaged
over the aerotank width for the time moment t
= 1.5 (dimensionless time). Each number in the Figures shows the
substrate concentration as a percentage of the maximum concentration
(this is the concentration at the entrance to the reactor Sin
= 360 mg/l).

Fig.
1. Substrate concentration in the bioreactor
(no plates,
scenario no. 1)

Fig.
2. Substrate concentration
in the
bioreactor
(one
plate, scenario
no. 2)

Fig.
3. Substrate concentration in the bioreactor
(two plates,
scenario no. 3)

Fig.
4. Substrate concentration
in the
bioreactor, t
= 1.5 (no flow
in the reactor,
scenario no. 4)
As can be seen from the above
figures, the field of substrate concentration inside the reactor can
be divided into two zones. The first zone corresponds to the
concentration range from 99 to 10% and occupies approximately the
first half of the reactor. The second zone corresponds to the
substrate concentration in the range of 10–3% (output from the
reactor). The border between the zones looks like a «slanting»
line. The second zone even has a «sparse» view. A significant
concentration of the substrate in the first zone is caused by its
constant ingress into the structure through the inlet.
Fig. 5 shows
the field of activated sludge concentration in the structure (each
number is the activated sludge concentration in percent of the
maximum concentration value at a given time, for this time – Xmax
= 620.76 mg/l). This concentration gradually decreases from the
inlet (where the active sludge enters the reactor) to the outlet.
Thus, Table
1 shows the average value of the substrate concentration at the
outlet from the reactor for the time t
=1.5 for each scenario.
As you can see from Table 1,
the plates in the strcture affect the efficiency of water
purification in the bioreactor. The most active process of water
purification takes place if there is no movement in the reactor
(scenario no. 4), i.e., if there is no new portions of the substrate
entering the reactor. Thus, it can be concluded that the quality
control of wastewater treatment in biological reactors is possible
through the use of additional elements in the structure – plates.
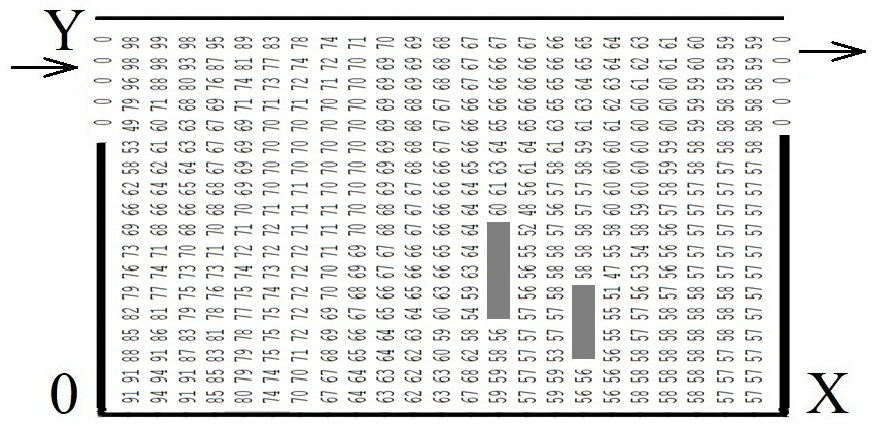
Fig.
5. Activated sludge concentration in the bioreactor, t
= 1.5 (two plates, scenario no. 3)
Table 1
Average
value of substrate concentration
at the bioreactor outlet
|
Scenario
|
Scenario
no. 1
|
Scenario
no. 2
|
Scenario
no. № 3
|
Scenario
no. 4
|
|
Concentration
|
19.95
mg/l
|
17.37
mg/l
|
14.71
mg/l
|
1.63
mg/l
|
It is also important to compare
the dynamics of the water purification rate in the reactor if there
is no movement (scenario no. 4) and when there is movement (scenario
no. 1). Biological reactors in scenarios no. 1 and no. 4 have the
same geometry, so this comparison is logical. Table 2 shows the
results of calculations for these reactors.
Table 2
Average
value of substrate concentration
at the bioreactor outlet
|
Time
(dimensionless)
|
Scenario
no. 4
|
Scenario
no. 1
|
|
0.6
|
148.32
mg/l
|
133.81
mg/l
|
|
0.96
|
44.71
mg/l
|
55.38
mg/l
|
|
1.2
|
12.25
mg/l
|
32.76
mg/l
|
|
1.4
|
2.89
mg/l
|
22.66
mg/l
|
|
1.5
|
1.63
mg/l
|
19.95
mg/l
|
Data
analysis of Table 2 shows that approximately from the moment of time
t = 0.96,
the deceleration of the water purification process starts in the
reactor, where the movement takes place, (scenario no. 1). By the
time moment t
= 1.5, the substrate concentration at the outlet from the reactor,
where there is movement, is significantly different from the
concentration for the reactor, where there is no movement.
Note that the time for
calculating each scenario was 5 s.
Originality
and practical value
A new numerical 2D model is
proposed to assess the operation efficiency of the aerotank. A
feature of the model is the ability to assess the operation of the
aerotank, taking into account its geometric shape and location of
additional plates in the structure. The simulated equations reflect
the fundamental law of continuum mechanics – the law of mass
conservation.
The developed numerical model
makes it possible to determine the concentration field of the
substrate and activated sludge in the bioreactor. The model can be
useful when performing calculations in the case of designing
biological treatment facilities or when reconstructing existing
bioreactors.
Conclusions
In the article, a new numerical
model has been developed that allows one to determine the aerotank
operation efficiency, taking into account its geometric shape. The
results of computational experiments show that the use of additional
elements in the aerotank improves the efficiency of water
purification.
In the future, this scientific
direction should be developed in the field of development of
numerical models for evaluating the aerotank operation efficiency
based on the Navier-Stokes equation.
list of reference links
Беляев
Н. Н., Нагорная Е. К. Математическое
моделирование массопереноса в
отстойниках систем водоотведения
: монография.
Днепропетровск : Новая идеология, 2012.
112 с.
Беляев
Н. Н., Козачина В. А.
Математическое моделирование
массопереноса в горизонтальных
отстойниках :
монография. Днепропетровск : Акцент
ПП, 2015. 115 с.
Василенко
О. А., Грабовський П. О., Ларкіна Г. М.,
Поліщук О. В., Прогульний В. Й. Реконструкція
і інтенсифікація споруд водопостачання
та водовідведення :
навч. посіб. Київ : ІВНВКП «Укргеліотек»,
2010. 272 с.
Карелин
Я. А., Жуков Д. Д., Журов В. Н., Репин Б. Н.
Очистка производственных
сточных вод в
аеротенках. Москва :
Стройиздат, 1973, 223 с.
Ласков
Ю. М., Воронов Ю. В., Калицун В. И. Примеры
расчетов канализационных сооружений.
Москва : Высшая
школа, 1981. 237 с.
Олійник О. Я, Айрапетян
Т. С. Моделювання очисних стічних вод
від органічних забруднень в
біореакторах-аеротенках зі зваженим
(вільно плаваючим) і закріпленим
біоценозом. Доповідь
НАН України. 2015. № 5.
С. 55–60. DOI: 10/15407/dopovidi2015.05.055
Alharbi
A. O. M. The biological treatment of wastewater : mathematical
models. Bulletin of the Australian
Mathematical Society.
2016. Vol. 94. Іss. 2. P. 347–348.
DOI: https://doi.org/10.1017/S0004972716000411
Amaral A., Gillot S.,
Garrido-Baserba M., Filali A., Karpinska A. M., Plósz B. G., …
Rosso D. Modelling gas-liquid mass transfer in wastewater treatment
: when current knowledge needs to encounter engineering practice
and vice-versa. Water Science &
Technology.
2019. Vol. 80.
Iss. 4. P. 607–619. DOI: 10.2166/wst.2019.253
Babaei A.,
Azadi R., Jaafarzadeh N., Alavi N. Application and Kinetic
Evaluation of upflow Anaerobic biofilm Reactor for Nitrogen Removal
from Wastewater. Iranian Journal of
Environmental Health Science and Engineering.
2013. Vol. 10. Iss. 1. P. 1–8. DOI:
10.1186/1735-2746-10-20
Bomba A., Klymiuk Y.,
Prysiazhniuk I., Prysiazhniuk O., Safonyk A.
Mathematical modeling of wastewater treatment from multicomponent
pollution by through microporous filling.
AIP Conference Proceedings.
2016. Vol. 1773.
P. 040003-1–040003-11. DOI: 10.1063/1.4964966
Dapelo D.,
Bridgeman G. A CFD strategy to retrofit an anaerobic digester
to improve mixing performance in wastewater treatment. Water
Science & Technology. 2020. Vol.
81. Iss. 8. P. 1646–1657.
DOI: 10.2166/wst.2020.086
Gao
H., Stenstrom M. K. Development and applications in CFD modeling
for secondary settling tanks over the last three decades : A
review. Water Environment Research.
2019. Vol. 92. Iss. 6. P. 796–820.
DOI: 10.1002/wer.1279
Gao
H., Stenstrom M. K. Influence of Model Parameters and Inlet
Turbulence Boundary Specification Methods in Secondary Settling
Tanks : Computational Fluid Dynamics Study. Journal
of Environmental Engineering.
2020. Vol. 146. Iss. 5. P. 04020028-1–04020028-12. DOI:
10.1061/(ASCE)EE.1943-7870.0001689
Gao
H., Stenstrom M. K. Computational Fluid Dynamics Analysis for
Improving Secondary Settling Tank Performance. Conference
: World Environmental and Water Resources Congress 2020.
2020. P. 212–224. DOI: 10.1061/9780784482988.021
Griborio
A. Secondary Clarifier Modeling : A
Multi-Process Approach. Dissertation
and Theses. University of New Orleans
: USA, 2004. 440 p.
Mocanu
C. R., Mihaillescu R. Numerical Simulation Wastewater Treatment
Aeration Processes. U. P. B. Sci.
Bull., Series D. 2012. Vol. 74. Iss.
2. P. 191–198.
Pereda
M., Zamarreno J. M. Agent – based modeling of an activated sludge
process in batch reactor. 19th
Mediterrian Conference on Control and Automation Aquis.
2011. P. 1128–1133. DOI: 10.1109/med.2011.5983027
М. М. БІЛЯЄВ1*,
М. В. ЛЕМЕШ2*,
В. В. БІЛЯЄВА3*,
П. Б. МАШИХІНА4*,
З. М. Якубовська5*
1*Каф.
«Гідравліка і водопостачання»,
Дніпровський національний університет
залізничного транспорту імені академіка
В. Лазаряна, вул. Лазаряна, 2,
Дніпро,
Україна, 49010, тел. +38 (056) 273 15 09,
ел. пошта
water.supply.treatment@gmail.com, ORCID 0000-0002-1230-8040
2*Каф.
«Гідравліка і водопостачання»,
Дніпровський національний
університет
залізничного транспорту імені академіка
В. Лазаряна, вул. Лазаряна, 2, Дніпро,
Україна, 49010, тел. +38 (056) 273 15 09, ел. пошта
water.supply.treatment@gmail.com,
ORCID 0000-0002-1531-7882
3*Каф.
«Аерогідромеханіка та енергомасоперенос»,
Дніпровський національний університет
імені Олеся Гончара,
пр. Гагаріна, 72, Дніпро, Україна,
49000, тел. +38 (056) 374
98 22,
ел. пошта water.supply.treatment@gmail.com,
ORCID 0000-0003-2399-3124
4*Каф.
«Гідравліка і водопостачання»,
Дніпровський національний університет
залізничного
транспорту імені академіка
В. Лазаряна, вул. Лазаряна, 2, Дніпро,
Україна, 49010,
тел. +38 (056) 273 15 09, ел. пошта
gidro_eko@ukr.net, ORCID 0000-0003-3057-9204
5*Каф.
«Фізика», Український
державний хіміко-технологічний
університет, пр. Гагаріна, 8,
Дніпро,
Україна, 49005, тел. +38 (056) 753 56 38, ел. пошта
physics@udhtu.edu.ua,
ORCID 0000-0002-9893-3479
КОМП’ЮТЕРНЕ
МОДЕЛЮВАННЯ ПРОЦЕСІВ
БІОЛОГІЧНОГО
ОЧИЩЕННЯ СТІЧНИХ ВОД
В
АЕРОТЕНКАХ ІЗ ПЛАСТИНАМИ
Мета.
Визначення ефективності роботи
аеротенків на етапі проєктування або
реконструкції біореакторів, у яких
здійснюється біологічне очищення
стічних вод, вимагає використання
спеціальних математичних моделей і
методів розрахунку. Основною метою
статті є розробка CFD-моделі для оцінки
ефективності роботи аеротенка. Методика.
Для комп’ютерного
розрахунку процесу біологічного
очищення стічних вод в аеротенку, з
урахуванням гідродинаміки, розроблена
чисельна модель. В основу моделі
покладено двовимірні рівняння збереження
маси для субстрату та активного мулу
та рівняння для потенціалу швидкості.
Процес біологічного перетворення
субстрату розраховано на базі моделі
Monod. Для чисельного інтегрування рівнянь
масопереносу активного мулу та субстрату
використано поперемінно-трикутну
різницеву схему розщеплення. При цьому
базові рівняння розщеплено на два
рівняння більш спрощеного вигляду.
Для чисельного інтегрування рівняння
для потенціалу швидкості здійснено
його розщеплення на два одновимірних
рівняння. Далі кожне рівняння розв’язано
за явною схемою. Для чисельного
інтегрування рівнянь, що описують
процес трансформації субстрату на базі
моделі Monod, використано метод Ейлера.
Результати.
Здійснено програмну реалізацію
побудованої чисельної моделі. Наведено
результати обчислювального експерименту
з дослідження процесу очищення стічних
вод в аеротенку за рахунок використання
додаткових елементів у споруді–пластин.
Це дозволяє зробити висновки, що
управління якістю очищення стічних
вод в аеротенках можливе за допомогою
застосування пластин. Наукова
новизна.
Розроблено багатофакторну CFD-модель,
що дозволяє швидко оцінити ефективність
роботи аеротенка. Особливістю моделі
є можливість оцінки роботи аеротенка
з урахуванням його геометричної форми
та розташування в споруді додаткових
пластин. Практична
значимість. Побудована
чисельна модель може
бути використана під час проведення
розрахунків у випадку проєктування
споруд аеротенків або під час визначення
ефективності очищення стічних вод за
нових умов експлуатації.
Ключові слова: очищення
води; чисельне моделювання; аеротенк;
модель Monod; CFD-моделі; біологічне очищення;
стічні води; активний мул; концентрація
забруднювальних речовин
Н. Н. БЕЛЯеВ1*,
М. В. ЛЕМЕШ2*,
В. В. БЕЛЯеВА3*,
П. Б. МАШИХиНА4*,
З. Н. ЯкубовскаЯ5*
1*Каф.
«Гидравлика и водоснабжение», Днипровский
национальный университет
железнодорожного
транспорта имени академика В. Лазаряна,
ул. Лазаряна, 2,
Днипро, Украина, 49010, тел.
+38 (056) 273 15 09,
эл. почта
water.supply.treatment@gmail.com, ORCID 0000-0002-1230-8040
2*Каф.
«Гидравлика и водоснабжение», Днипровский
национальный университет
железнодорожного
транспорта имени академика В. Лазаряна,
ул. Лазаряна, 2,
Днипро, Украина, 49010, тел.
+38 (056) 273 15 09,
эл. почта
water.supply.treatment@gmail.com, ORCID 0000-0002-1531-7882
3*Каф.
«Аэрогидромеханика и энергомассоперенос»,
Днипровский национальный
университет имени Олеся Гончара,
пр. Гагарина, 72, Днипро,
Украина, 49000,
тел. +38 (056) 374
98 22, эл. почта
water.supply.treatment@gmail.com,
ORCID 0000-0003-2399-3124
4*Каф.
«Гидравлика и водоснабжение», Днипровский
национальный университет
железнодорожного
транспорта имени академика В. Лазаряна,
ул. Лазаряна, 2,
Днипро, Украина, 49010, тел.
+38 (056) 273 15 09, эл. почта gidro_eko@ukr.net,
ORCID
0000-0003-3057-9204
5*Каф.
«Физика»,
Украинский государственный
химико-технологический
университет,
пр. Гагарина, 8, Днипро, Украина, 49005,
тел. +38 (056) 753 56 38,
эл. почта physics@udhtu.edu.ua,
ORCID 0000-0002-9893-3479
КОМПЬЮТЕРНОЕ МОДЕЛИРОВАНИЕ
ПРОЦЕССОВ
БИОЛОГИЧЕСКОЙ ОЧИСТКИ СТОЧНЫХ
ВОД
В АЭРОТЕНКАХ С ПЛАСТИНАМИ
Цель. Определение
эффективности работы аэротенка на
этапе проектирования или реконструкции
биореакторов, в которых осуществляется
биологическая очистка сточных вод,
требует использования специальных
математических моделей и методов
расчета. Основной целью статьи является
разработка CFD-модели для оценки
эффективности работы аэротенков.
Методика. Для
компьютерного расчета процесса
биологической очистки сточных вод в
аэротенках, с учётом гидродинамики,
разработана численная модель. В основу
модели заложены двухуровневые уравнения
сохранения массы для субстрата и
активного ила и уравнение для потенциала
скорости. Процесс биологического
преобразования субстрата рассчитан
на базе модели Monod. Для численного
интегрирования уравнений массопереноса
активного ила и субстрата использована
попеременно–треугольная разностная
схема расщепления. При этом базовые
уравнения разделены на два уравнения
более упрощенного вида. Для численного
интегрирования уравнения для потенциала
скорости осуществлено его расщепление
на два одномерных уравнения. Далее
каждое уравнение решено по явной схеме.
Для численного интегрирования уравнений,
описывающих процесс трансформации
субстрата на базе модели Monod, использован
метод Эйлера. Результаты.
Осуществлена программная реализация
построенной численной модели. Приведены
результаты вычислительного эксперимента
по исследованию процесса очистки
сточных вод в аэротенке за счет
использования дополнительных элементов
в сооружении – пластин. Это проводит
к выводу, что управление качеством
очистки сточных вод в аэротенках
возможно с помощью применения пластин.
Научная новизна.
Разработана многофакторная CFD-модель,
позволяющая быстро оценить эффективность
работы аэротенка. Особенностью модели
является возможность оценки работы
аэротенка с учетом его геометрической
формы и расположения в сооружении
дополнительных пластин. Практическая
значимость. Построенная
численная модель может быть использована
при проведении расчетов в случае
проектирования сооружений аэротенков
или при определении эффективности
очистки сточных вод в новых условиях
эксплуатации.
Ключевые слова: очистка
воды; численное моделирование; аэротенк;
модель Monod; CFD-модели; биологическая
очистка; сточные воды; активный ил;
концентрация загрязняющих веществ
REFERENCES
Biliaiev,
N. N., & Nagornaya, E. K. (2012). Matematicheskoye
modelirovaniye massoperenosa v otstoynikakh sistem vodootvedeniya:
monografiya. Dnepropetrovsk: Novaya ideologiya. (in Russian)
Biliaiev,
N. N., & Kozachina, V. A. (2015). Modelirovaniye
massoperenosa v gorizontalnykh otstoynikakh:
monografiya. Dnepropetrovsk: Aktsent PP. (in Russian)
Vasylenko,
O. A., Hrabovskyi, P. O., Larkina, H. M., Polishchuk, O. V., &
Prohulnyi, V. Y. (2010). Rekonstruktsiia
i intensyfikatsiia sporud vodopostachannia ta vodovidvedennia:
navchalnyi posibnyk.
Kyiv: IVNVKP «Ukrheliotek». (in Ukrainian)
Karelin,
Ya. A., Zhukov,
D. D., Zhurov,
V. N., & Repin,
B. N. (1973). Ochistka
proizvodstvennyh
stochnyh
vod
v
aerotenkah.
Moscow:
Stroiyzdat. (in
Russian)
Laskov,
Yu. M., Voronov, Yu. V., & Kalicun, V. I. (1981). Primery
raschetov kanalizacionnyh sooruzhenij.
Moscow: Vysshaya Shkola.
(in Russian)
Oleynik,
A. Y., & Airapetyan, T. S. (2015). The modeling of the
clearance of waste waters from organic pollutions in
bioreactors-aerotanks with suspended (free flow) and fixed
biocenoses. Reports of the National
Academy of Sciences of Ukraine, 5,
55-60. DOI: 10.15407/dopovidi2015.05.055 (in
Ukrainian)
Alharbi, A. O. M. (2016). The
biological treatment of wastewater: mathematical models. Bulletin
of the Australian Mathematical Society, 94(2),
347-348. DOI: 10.1017/s0004972716000411 (in
English)
Amaral,
A., Gillot, S., Garrido-Baserba, M., Filali, A., Karpinska, A. M.,
Plósz, B. G., … & Rosso, D. (2019). Modelling gas-liquid
mass transfer in wastewater treatment: when current knowledge needs
to encounter engineering practice and vice-versa. Water
Science & Technology,
80(4),
607-619. DOI: 10.2166/wst.2019.253
(in English)
Babaei,
A., Azadi, R., Jaafarzadeh, N., & Alavi, N. (2013). Application
and Kinetic Evaluation of upflow Anaerobic biofilm Reactor for
Nitrogen Removal from Wastewater. Iranian
Journal of Environmental Health Science and Engineering, 10(1),
1-8. DOI:
10.1186/1735-2746-10-20 (in English)
Bomba,
A., Klymiuk, Y., Prysiazhniuk, I., Prysiazhniuk, O., & Safonyk,
A. (2016). Mathematical modeling of wastewater treatment from
multicomponent pollution by through microporous filling. AIP
Conference Proceedings, 1773,
040003-1-040003-11. DOI: 10.1063/1.4964966 (in
English)
Dapelo, D.,
& Bridgeman, G. (2020). A CFD strategy to retrofit an
anaerobic digester to improve mixing performance in wastewater
treatment. Water Science &
Technology, 81(8), 1646-1657.
DOI: 10.2166/wst.2020.086
(in English)
Gao,
H., & Stenstrom, M. K. (2019). Development and applications in
CFD modeling for secondary settling tanks over the last three
decades: A review. Water Environment
Research, 92(6),
796-820
DOI: 10.1002/wer.1279
(in English)
Gao,
H., & Stenstrom, M. K. (2020). Influence of Model Parameters
and Inlet Turbulence Boundary Specification Methods in Secondary
Settling Tanks: Computational Fluid Dynamics Study. Journal
of Environmental Engineering,
146(5), 04020028-1-04020028-12.
DOI: 10.1061/(ASCE)EE.1943-7870.0001689
(in English)
Gao, H.,
& Stenstrom, M. K. (2020). Computational Fluid Dynamics
Analysis for Improving Secondary Settling Tank Performance.
Conference: World Environmental and
Water Resources Congress 2020,
212-224.
DOI: 10.1061/9780784482988.021
(in English)
Griborio,
A. (2004). Secondary Clarifier
Modeling: A Multi-Process Approach. Dissertation and Theses.
USA, University of New Orleans Publ. (in English)
Mocanu,
C. R., & Mihaillescu, R. (2012). Numerical Simulation
Wastewater Treatment Aeration Processes.U.
P. B. Sci. Bull., Series D, 74(2),
191-198. (in English)
Pereda,
M., & Zamarreno, J. M. (2011). Agent–based modeling of an
activated sludge process in batch reactor. 19th
Mediterrian Conference on Control and Automation Aquis, 1128-1133.
DOI: 10.1109/med.2011.5983027
(in English)
Received:
05.06.2020
Accepted:
07.10.2020
–
activated sludge
measurement coefficient;
– time;
–
averaged concentration
of activated
sludge in the
bioreactor ;
– averaged concentration
of substrate in
the bioreactor;
– diffusion coefficient; Y –
parameter in the Monod model;
–
components of the water flow velocity in the bioreactor in the
direction of the x, y axes, respectively;
– components of the diffusion coefficients at the considered plane
point in the x, y
direction.
,
– known concentrations of substrate and sludge, respectively;
;
,
,
– concentrations in the last computational cell;
– concentrations in the previous computation cell;
(6)
(7)
(8)
,
.
(9)
(10)
,
.
(11)
(14)
(15)
=
3 495 m3/day
– waste water consumption;
mg/l –
concentration of activated sludge that enters the reactor;