ISSN 2307–3489 (Print), ІSSN 2307–6666 (Online)

Наука та прогрес транспорту. Вісник Дніпропетровського
національного університету залізничного транспорту, 2020, № 3 (87)

Introduction

Particularly dangerous are industrial sites, where emergencies are possible with the sudden formation of intense, diverse impact factors (shock wave, heat wave, emission of toxic substances). In this case, it is extremely important to predict the risk of damage to personnel in the workplace during emergencies.

Analytical and numerical forecasting methods are used to determine the risk of personnel damage in case of emergencies [3, 6–15]. These methods are focused on assessing the risk of toxic damage to humans during accidental releases of toxic substances. At the same time, the task of predicting the risk of thermal damage to people during emergencies is also relevant. This is especially important in cases where there is a fire inside industrial shops with a significant number of workers. The risk of thermal damage may occur, for example, in the event of emergency ignition of solid rocket propellant in the shops. The temperature of the combustion products of solid rocket propellants is very high. Due to the high emission of heated propellant combustion products, the area of thermal contamination spreads across the shop and there is a risk of thermal damage to personnel in the work areas. To assess the risk of thermal damage to personnel, it is necessary to have mathematical models.

Purpose

Fig. 1. Solid propellant engine of Thunder-2 rocket
in the working room
(https://allkharkov.ua/news/state/grom-grianet-v-etom-gody.html)

The paper envisages the development of a numerical model for assessing the risk of thermal damage to people in the workplace in the event of emergency burning of solid rocket propellant (SRP) for Thunder-2 rocket (Fig. 1).

Methodology

To assess the risk of thermal damage to people in the workplace in the event of emergency burning of solid rocket propellant, we will use the equation of convective heat transfer (two-dimensional, planned model, Boussinesq approximation) [4, 7]:

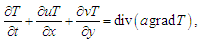(1)

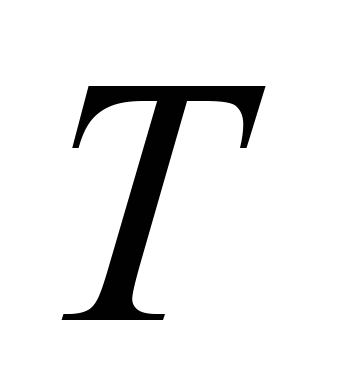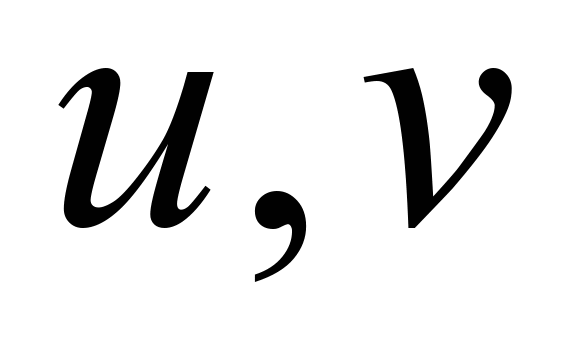where – is the air temperature; – components of the air velocity vector in the building; 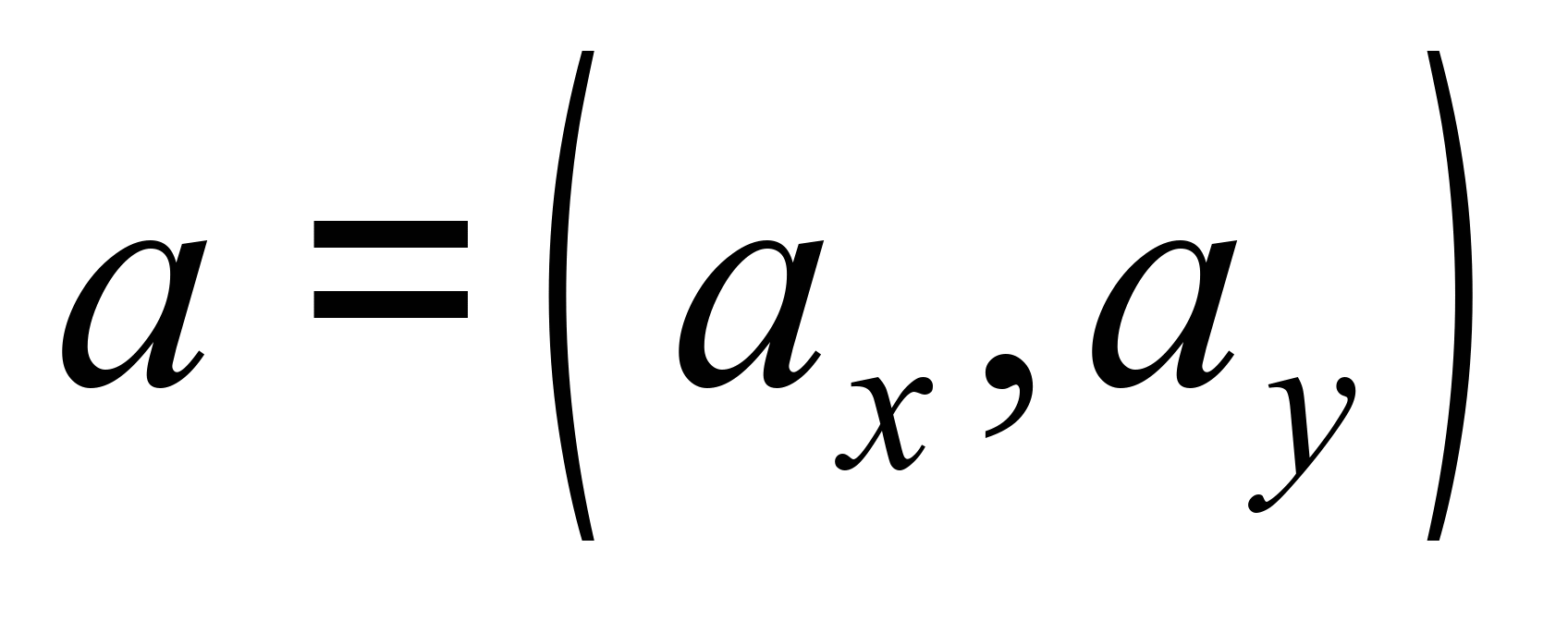– temperature conductivity coefficients; 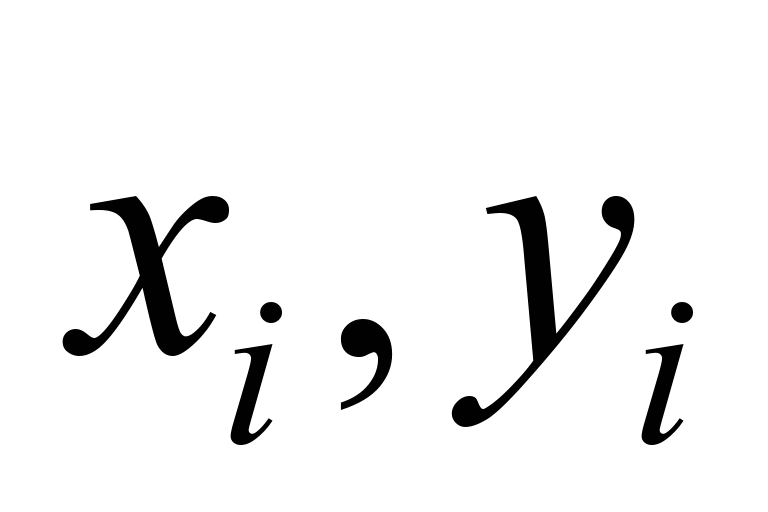– Cartesian coordinates;  – time.

Statement of boundary conditions for energy equation (1) is as follows [7]:

1. At the entrance to the calculation area:

,

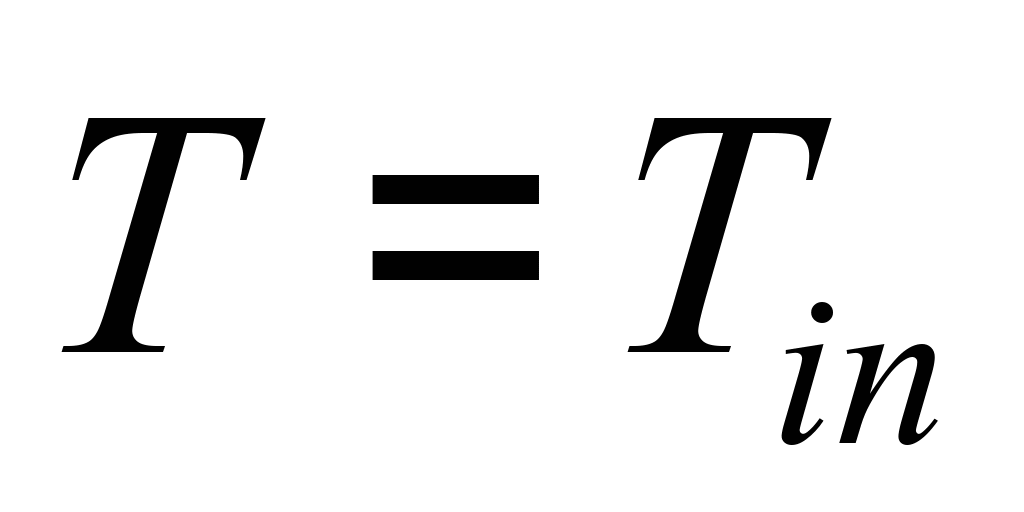where 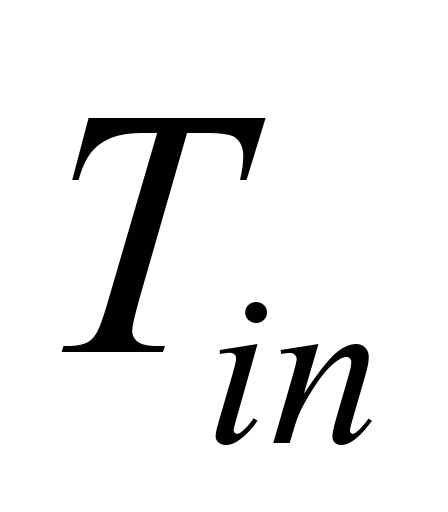– known air temperature (for example, ).

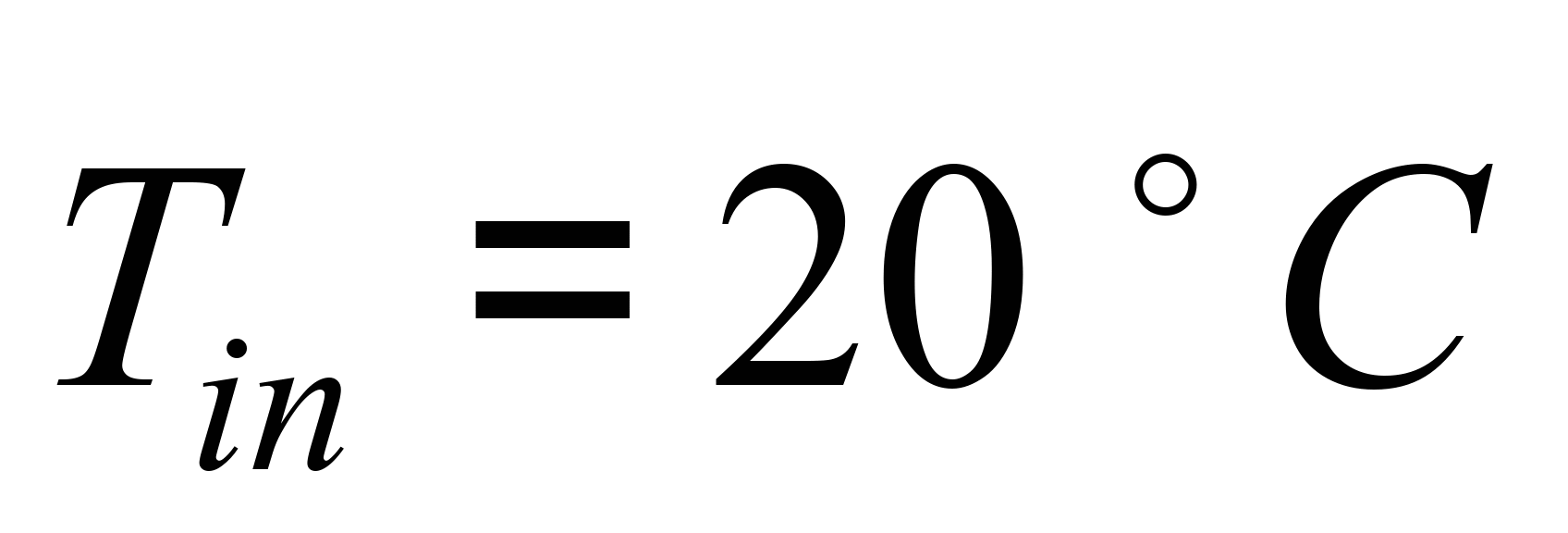2. At the boundary of the outflow of the calculation area:

,

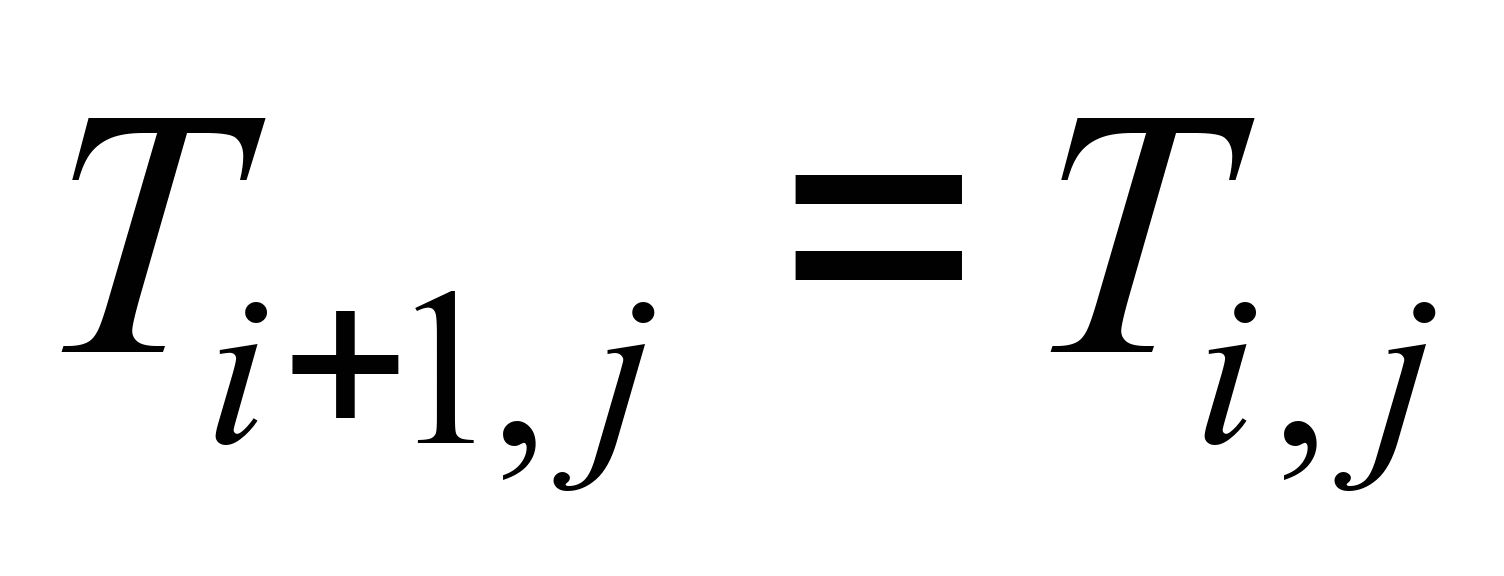where 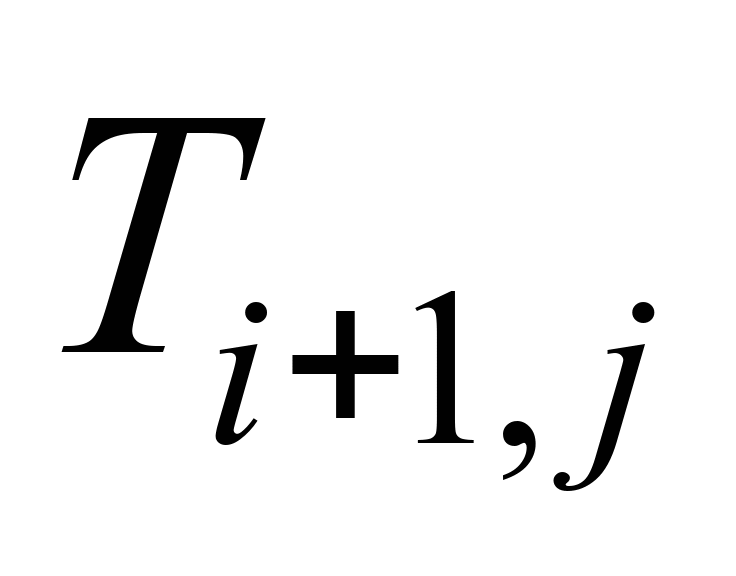– temperature in the last difference cell; 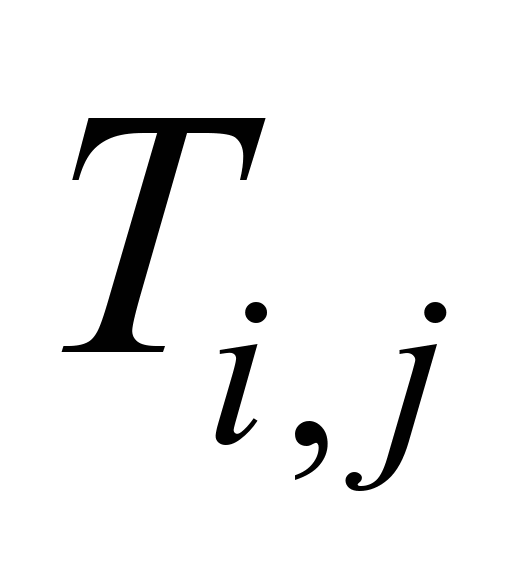– the temperature in the previous cell.

3. On the hard boundaries

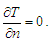
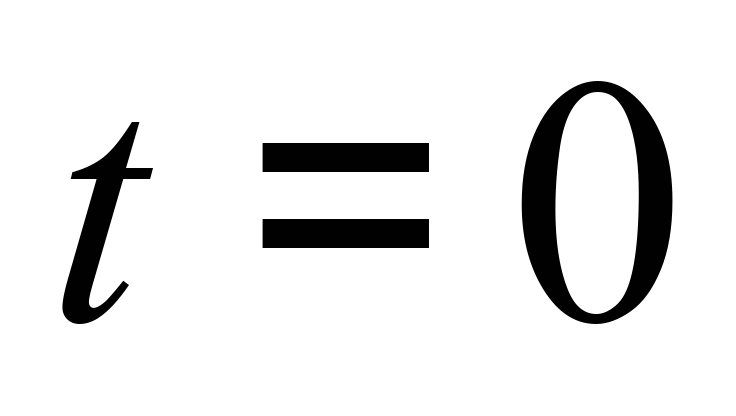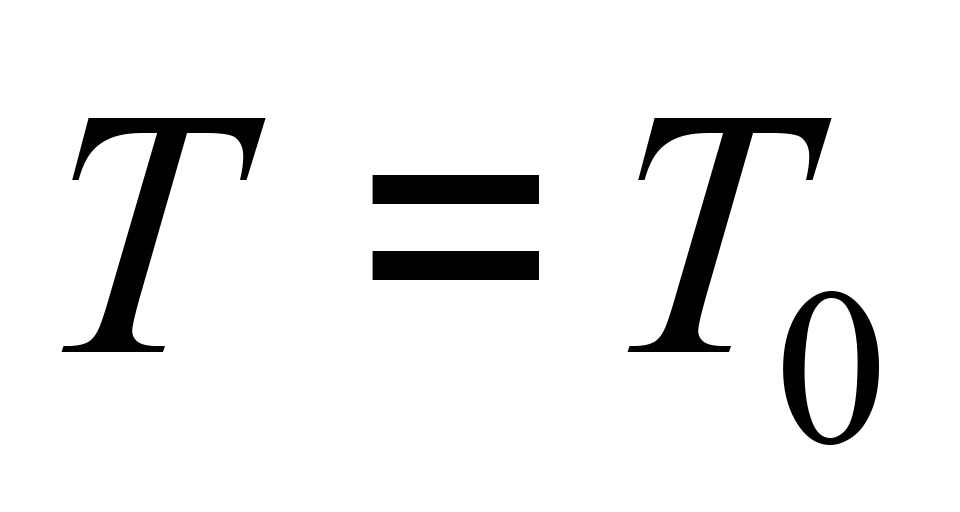For the moment of time , i.e. at the beginning of the calculation, we set the condition , where 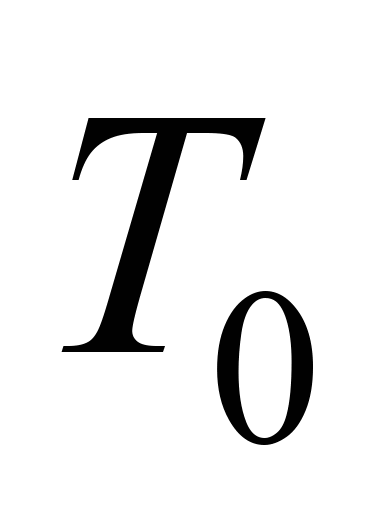is the known air temperature in the calculation area, for example . The temperature of burning products is set at the place of solid propellant combustion [7].

By solving equation (1) we can determine the temperature distribution in the working areas in the shop. The important thing is that one can obtain a non-steady solution to the problem – the data of changes in the temperature distribution in the shop over time. The risk of thermal damage [7] is determined from the following condition: if the air temperature in the working area is more than 100 ºC, at which there is a complete protein denaturation, then at this point in the working area it is assumed that the risk of damage is 100 %.

To numerically solve equation (1), we split the energy equation at the differential level into a sequence of such equations [5, 7]:

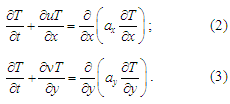
Next, we use a non-explicit difference scheme to numerically integrate one-dimensional energy equations [5, 7]. Let us perform the following transformations:

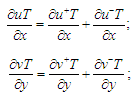
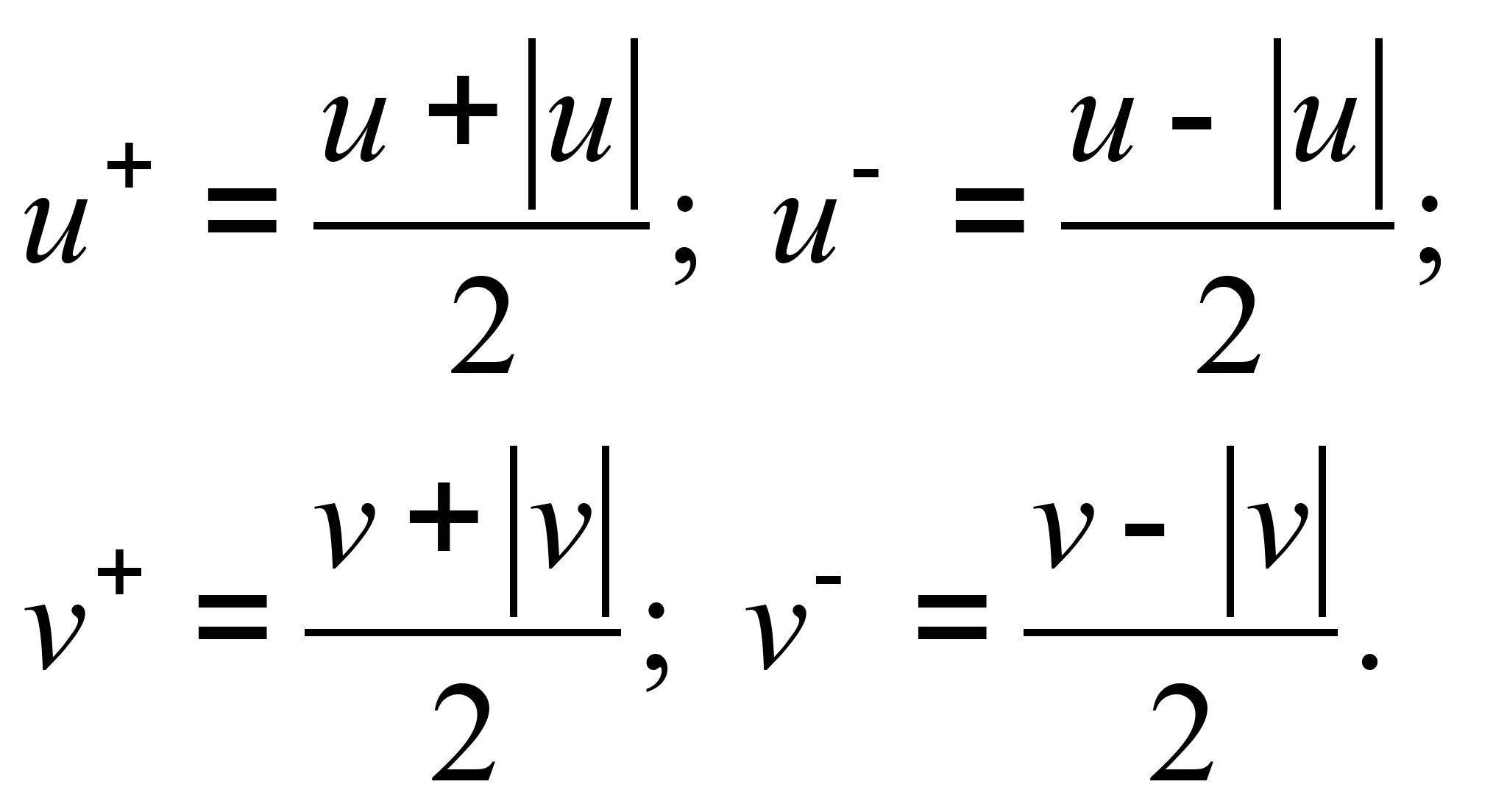
Let us perform further approximation of derivatives for equations from system (2), (3) [7]:

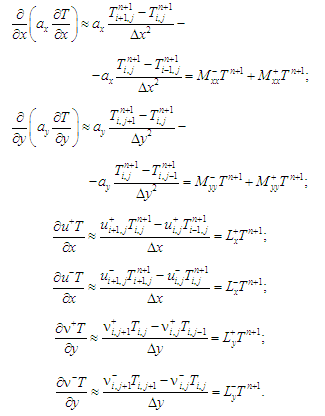
The splitting scheme for equation (2) is written as follows [5, 7]:

– in the first step, the difference equation has the form:

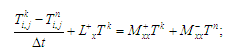(4)

– in the second step, the difference equation takes the form:

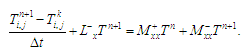(5)

The splitting scheme for numerical integration of equation (3) will be as follows:

– in the first step we obtain the difference equation:

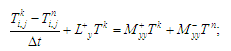(6)

– in the second step of splitting, the difference equation will have the form:

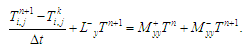(7)

The unknown value of temperature T at each splitting step (4) – (7) is calculated by the formula of the point-to-point computation.

The air velocity field u, v, in the presence of obstacles at the industrial site, is determined based on the model of the movement potential [2, 3, 7]:

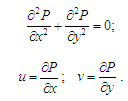(8)

The boundary conditions for equation (8) are as follows [2, 3, 7]:

1) 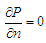 – on the hard boundaries;

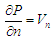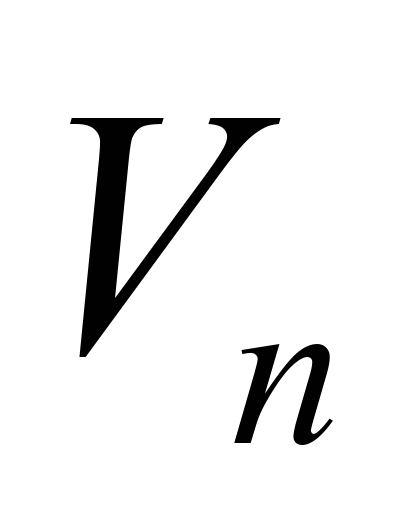2)  – at the boundary where the flow enters through the ventilation system, – is the known air velocity;

3) P = const – at the boundary of the outflow of the calculation area.

To numerically solve this equation, we will use the scheme of conditional approximation [5]. The difference splitting equations in this case are as follows [5]:

– in the first step of splitting:

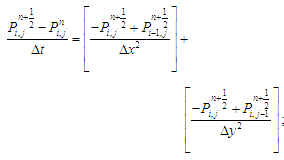(9)

– in the second step of splitting:

(10)

Unknown values of the velocity potential 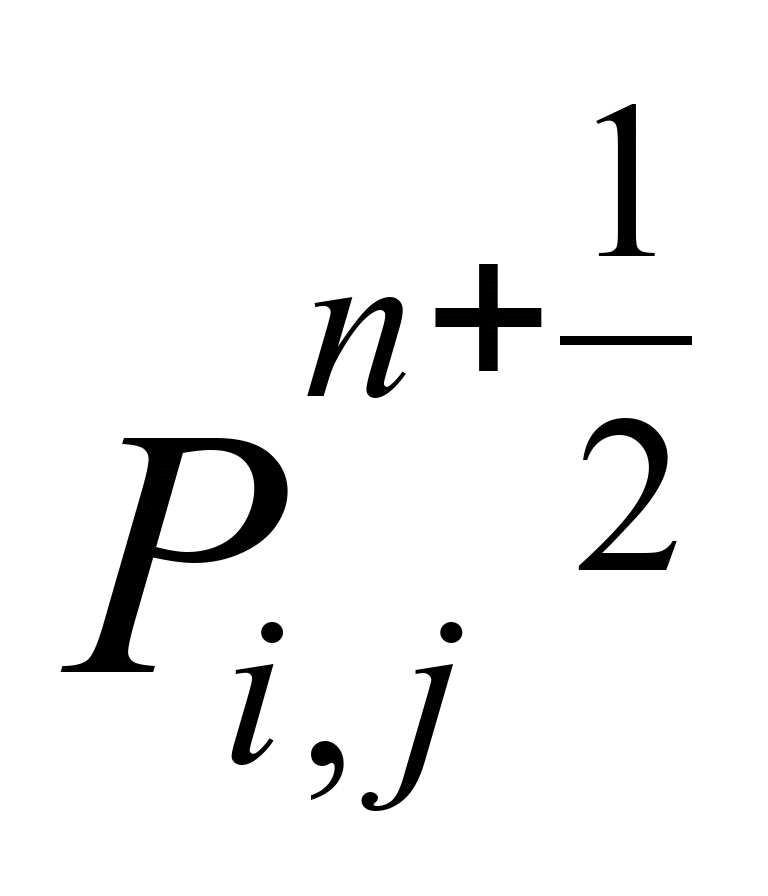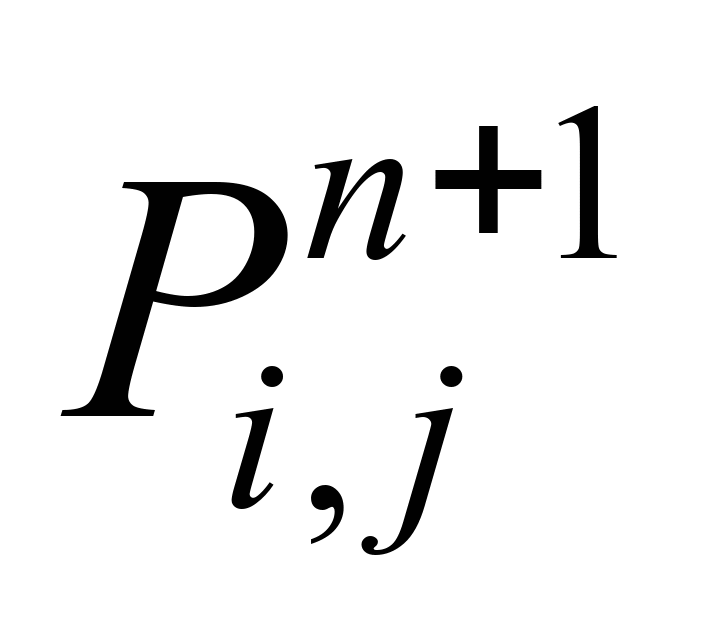_, in each splitting step (9), (10) are determined by the method of point-to-point computation.

The calculation according to dependences (9), (10) ends when [7]:

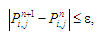
where 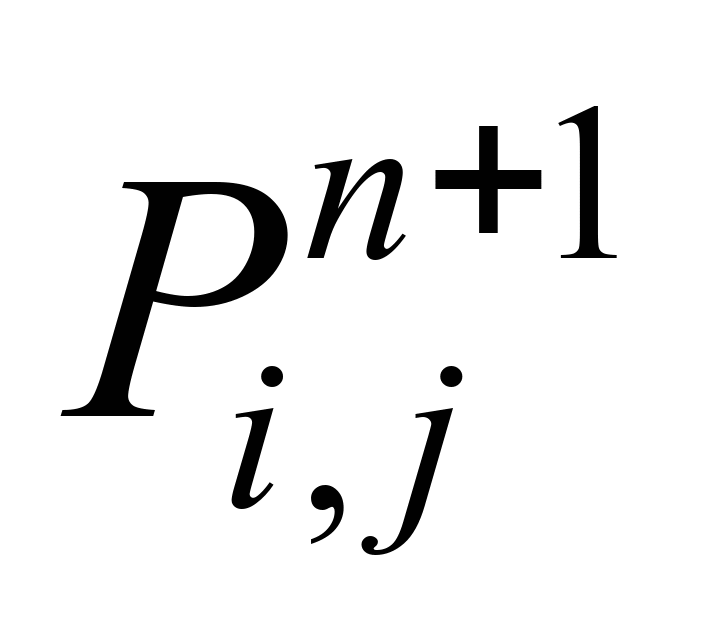– the value of the velocity potential on the new iteration; – the value of the velocity potential on the previous iteration; ε – small number.

To start the calculation it is necessary to set the «initial» value of the velocity potential in the calculation area, during the calculation we take the value.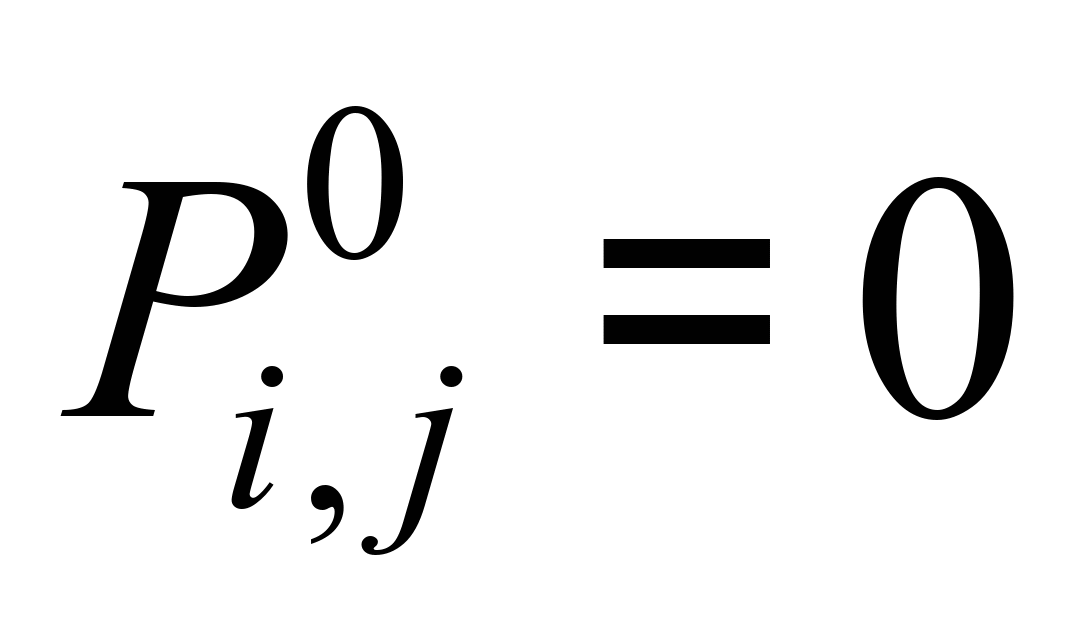

The components of the air velocity vector are calculated on the sides of the computational cell as follows [3, 7]:

After calculating the velocity component, we perform the solution of the energy equation where this field is used.

Let us consider the algorithm for solving the problem of determining the potential risk of thermal damage to personnel in the shop [1, 2, 7]. First, based on numerical integration of fundamental equations (energy equations and equations for velocity potential) we perform the calculation of the temperature distribution in the shop for different emergencies. When assessing the risk, we assume that the probability of each emergency is known. After calculating the temperature field for different accident scenarios in the shop, the computer program determines the areas where the value of air temperature is greater than the temperature of the damage.

Then we print the forecast results of the thermal damage risk for a certain time point. In this article the various probability of fire point of rocket propellant in shop is considered.

The numerical solution of all difference equations has been programmed. FORTRAN was used to create computer code to simulate the process of thermal air pollution.

Findings

The constructed numerical model was used to assess the potential risk of thermal damage to people in the shop where the solid rocket propellant of Thunder-2 rocket is located. In the event of emergency that leads to the burning of solid rocket propellant inside the shop, there may be a «domino» effect – the ignition of the propellant of a neighboring rocket engine.

The scheme of the calculation area is shown in Fig. 2. The arrow in the Figure shows the direction of air movement in the shop. During the calculations, the task was set to determine the level of temperature pollution in the shop over time and in the working area (position no. 2 in Fig. 2). The air exchange rate in the shop is equal to к_р = 15. The initial air temperature in the shop is 20 ºС. We assumed that the temperature of combustion products at the accident site is equal to 1 000 ºC [7]. For the calculations, the influence of the engine housings in the shop on the formation of temperature fields was taken into account. Below Fig. 3 and 4 show the changes dynamics in air temperature in the shop for different time periods after the emergency.

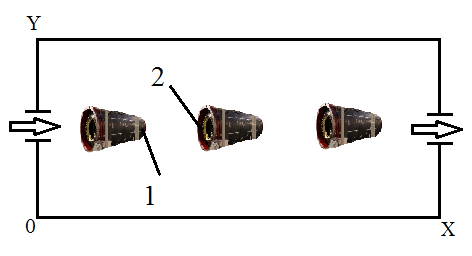
Fig. 2. Scheme of the calculation area:
1 – fire point of propellant in the shop;
2 – solid propellant engines of the Thunder-2 rocket

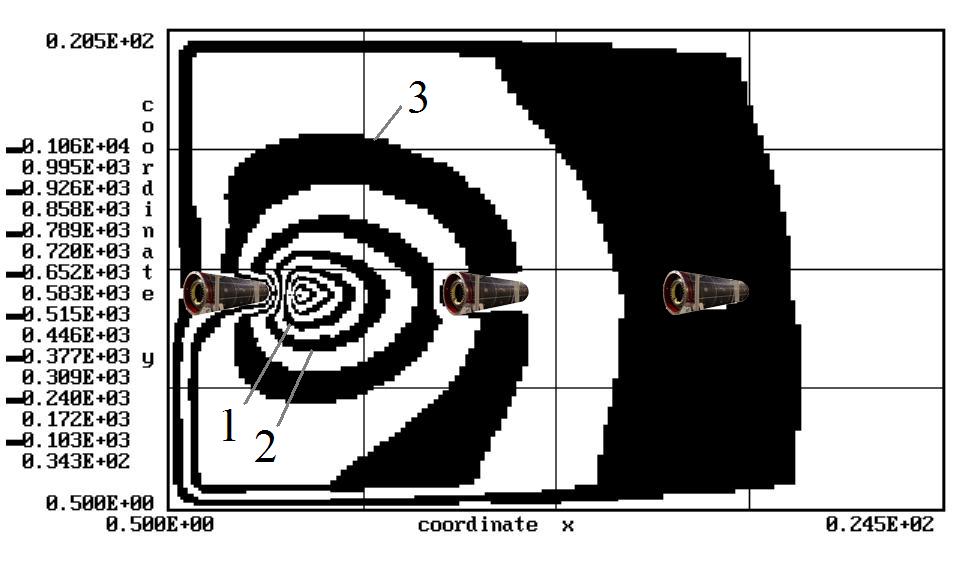
Fig. 3. Zone of thermal pollution (isotherms)
inside the shop, t = 11 sec:
1 – T = 789 ºС; 2 – T=625 ºС; 3 – T = 377 ºС

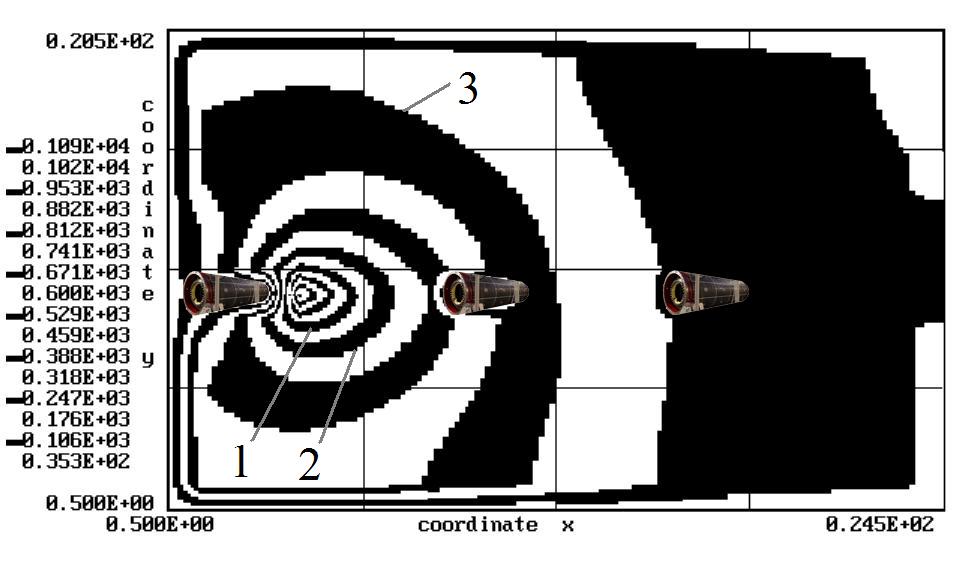
Fig. 4. Zone of thermal pollution (isotherms)
inside the shop, t = 15 sec:
1 – T = 812 ºС; 2 – T = 671 ºС; 3 – T = 388 ºС

The analysis of the given Figures shows that the thermal pollution zone is formed very quickly in the shop. A zone with a high temperature gradient is formed near the emission source.

Fig. 5 shows the temperature change over time in the working area.

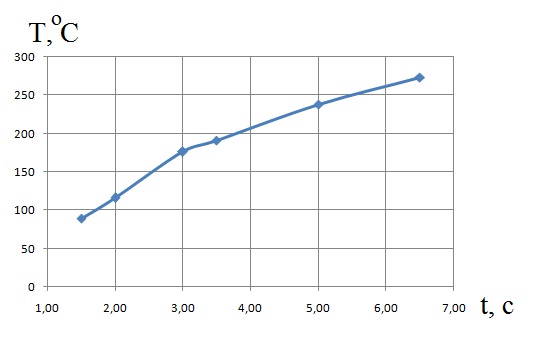
Fig. 5. Change in air temperature over time
in the working area

As we can see from the Figure, the air temperature rises very quickly in the working area. In 7 seconds it almost reaches the value of 270 ºC, i.e. there is a risk not only of thermal damage to personnel, but also of a «domino» effect – ignition of a neighboring rocket engine, which is located at a distance of 5 m from the engine where the fire started. Fig. 7, 8 show the results of solving another problem to assess the risk of thermal damage to personnel in the shop. The situation when several solid propellant engines are located in the shop is considered (Fig. 6), and the further probability of emergency – the probability of engine ignition in zone no. 1 is 25 %, and in zone no. 2 – 75 %.

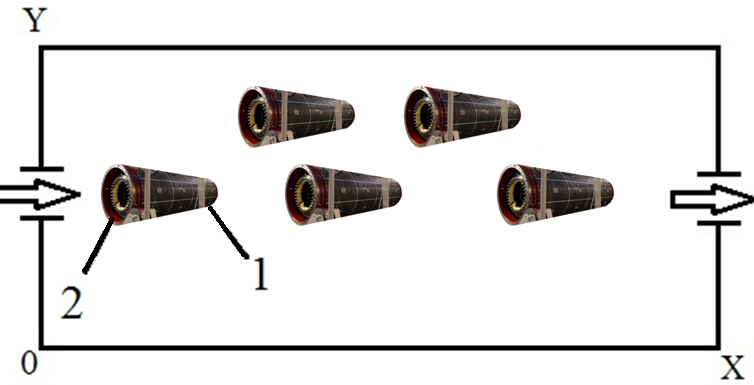
Fig. 6. Scheme of the calculation area (shop where solid propellant engines are located):
1 – fire point no. 1 (fire probability 25 %);
2 – fire point no. 2 (fire probability 75 %)

Fig. 7, 8 show the matrix of potential territorial risk of thermal damage to people in the shop for different points in time in the case of the realization of these probable situations.

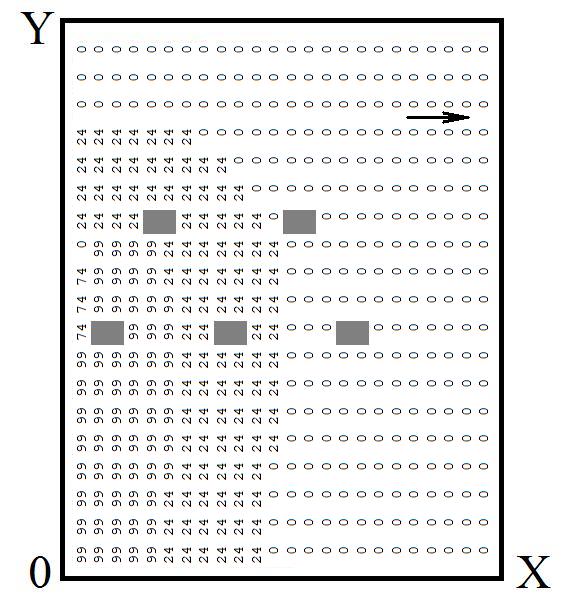
Fig. 7. The probability of thermal damage
to personnel in the shop at time t = 12 sec

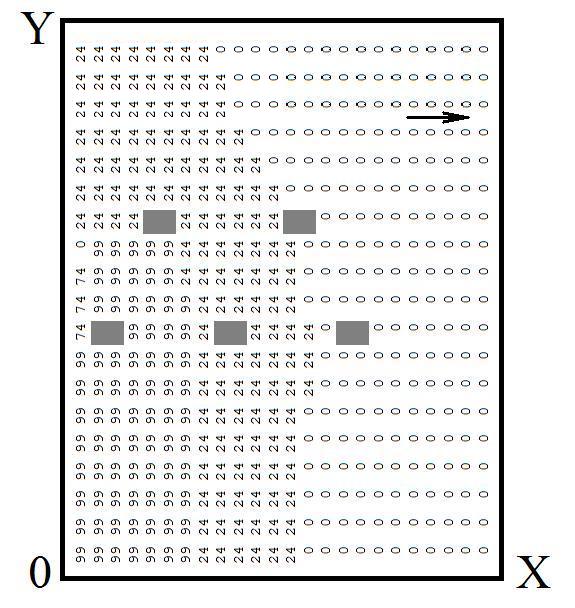
Fig. 8. The probability of thermal damage
to personnel in the shop at time t = 24 sec

As we can see from the given Figures, for the considered situations the risk of thermal damage to the personnel in the shop is extremely high, because the zone of thermal damage is formed very quickly.

It should be noted that it took about 5 seconds computer time to solve the problem.

Originality and practical value

A computer model has been built to quickly assess the potential risk of thermal damage to people in the shop in the event of emergency ignition of solid rocket propellant. A code has been developed that allows to quickly model the formation of temperature fields in the shop in case of emergency ignition of solid rocket propellant and to determine the areas of potential thermal damage to workers based on this information.

The developed computer program can be used to assess the risk of thermal damage in the chemical industry in the event of emergency.

Conclusions

1. A computer model has been proposed to predict the risk of thermal damage to shop workers in the event of emergency that results in the ignition of solid rocket propellant.

2. Based on computer simulation data, it can be concluded that in the event of emergency in the shop, there will be lethal thermal damage to workers.

3. The formation of thermal damage zones in the shop is very quickly, so it is necessary to develop measures to save the lives of workers.

LIST OF REFERENCE LINKS

1. Алымов В. Т., Тарасова Н. П. Техногенный риск. Анализ и оценка : учеб. пособие для вузов. Москва : Академкнига, 2004. 118 с.

2. Беляев Н. Н., Гунько Е. Ю., Кириченко П. С., Мунтян Л. Я. Оценка техногенного риска при эмиссии опасных веществ на железнодорожном транспорте. Кривой Рог : Р. А. Козлов, 2017. 127 с.

3. Згуровский М. З., Скопецкий В. В., Хрущ В. К., Беляев Н. Н. Численное моделирование распространения загрязнения в окружающей среде. Киев : Наук. думка, 1997. 368 с.

4. Марчук Г. И. Математическое моделирование в проблеме окружающей среды. Москва : Наука, 1982. 320 с.

5. Самарский А. А. Теория разностных схем. Москва : Наука, 1983. 616 с.

6. Berlov O. V. Atmosphere protection in case of emergency during transportation of dangerous cargo. Наука та прогрес транспорту. 2016. № 1 (61). С. 48–54. DOI: https://doi.org/10.15802/stp2016/60953

7. Biliaiev M. M., Berlov O. V., Kozachina V. A., Kalashnikov I. V., Shevchenko O. V. Risk assessment of thermal damage to people at industrial sites in case of emergency of burning solid propellant. Наука та прогрес транспорту. 2020. № 1 (85). С. 7–16. DOI: 10.15802/stp2020/200752

8. Biliaiev M. M., Kharytonov M. M. Numerical Simulation of Indoor Air Pollution and Atmosphere Pollution for Regions Having Complex Topography. NATO Science for Peace and Security. Series C : Environmental Security. Dordrecht, 2012. P. 87–91. DOI: 10.1007/978-94-007-1359-8_15

9. Boris J., Patnaik G., Obenschain K., Moses A., Obenschain M.-Y., Theodore Y., Delaney J., Donnelly J. Fast and accurate prediction of windborne contaminant plumes for civil defense in cities. The Fifth International Symposium on Computational Wind Engineering (CWE2010). 2010. P. 1–9.

10. Cao C., Li C., Yang Q., Zhang F. Multi-Objective Optimization Model of Emergency Organization Allocation for Sustainable Disaster Supply Chain. Sustainability. 2017. Vol. 9. Іss. 11. P. 1–22. DOI: 10.3390/su9112103

11. Ilić P., Ilić S., Stojanović Bjelić L. Hazard modelling of accidental release chlorine gas using modern tool-aloha software. Quality of Life (Banja Luka) - APEIRON. 2018. Vol. 16. Iss. 1–2. P. 38–45. DOI: 10.7251/QOL1801038I

12. Komatina D. Ilić, Galjak J., Belošević S. Simulation of chemical accidents with acetylene in «messer tehnogas» kraljevo plant by «aloha» software program. The University Thought - Publication in Natural Sciences. 2018. Vol. 8. Iss. 2. P. 19–26. DOI: 10.5937/univtho8-18014

13. Lacome J.-M., Truchot D., Duplantier S. Application of an innovative risk dedicated procedure for both conventional and 3D atmospheric dispersion models evaluation. 18 International Conference on Harmonisation within Atmospheric Dispersion Modelling for Regulatory Purposes (HARMO 18) (Bologne, Oct 2017). Bologne, 2017. P. 821–825.

14. Lee H., Sohn J.-R., Byeon S.-H., Yoon S., Moon K. Alternative Risk Assessment for Dangerous Chemicals in South Korea Regulation : Comparing Three Modeling Programs. Int. J. Environ. Res. Public Health. 2018. Vol. 15. Iss. 8. Р. 1–12. DOI: 10.3390/ijerph15081600

15. Sideris G. M., Christolis M. N., Markatos. N. C. Numerical simulation of pollutants dispersion around an electric-arc-furnace in case of an accidental release. Biodiversity International Journal. 2020. Vol. 4. Iss. 1. P. 50‒57. DOI: 10.15406/bij.2020.04.00164

М. М. БІЛЯЄВ^1*, о. в. БЕРЛОВ^2*, В. В. БІЛЯЄВА^3*, в. А. козачина^4*, І. В. калашніков^5*

^1*Каф. «Гідравліка та водопостачання», Дніпровський національний університет залізничного транспорту імені академі-ка В. Лазаряна, вул. Лазаряна, 2, Дніпро, Україна, 49010, тел. +38 (056) 273 15 09, ел. пошта water.supply.treatment@gmail.com, ORCID 0000-0002-1531-7882
^2*Каф. «Безпека життєдіяльності», ДВНЗ Придніпровська державна академія будівництва та архітектури, вул. Чернишевського, 24а, Дніпро, Україна, 49600, тел. +38 (056) 756 34 57, ел. пошта berlov.oleksandr@pgasa.dp.ua, ORCID 0000-0002-7442-0548
^3*Каф. «Аерогідромеханіка та тепломасоперенос», Дніпровський національний університет імені Олеся Гончара, просп. Гагаріна, 72, Дніпро, Україна, 49000, тел. +38 (056) 374 98 22, ел. пошта water.supply.treatment@gmail.com, ORCID 0000-0003-2399-3124
^4*Каф. «Гідравліка та водопостачання», Дніпровський національний університет залізничного транспорту імені академіка В. Лазаряна, вул. Лазаряна, 2, Дніпро, Україна, 49010, тел. +38 (056) 273 15 09, ел. пошта v.kozachyna@gmail.com, ORCID 0000-0002-6894-5532
^5*Харківське відділення філії «Проєктно-вишукувальний інститут залізничного транспорту» АТ «Українська залізниця», вул. Котляра, 7, Харків, Україна, 61052, тел. +38 (057) 724 41 25, ел. пошта uzp38@ukr.net, ORCID 0000-0002-2814-380X

аварійнЕ горіння твердого ракетного

палива: ОЦІНКА РИЗИКУ УРАЖЕННЯ

ЛЮДЕЙ В РОБОЧОМУ ПРИМІЩЕННІ

Мета. Ця робота передбачає розробку комп’ютерної моделі для розрахунку ризику термічного ураження людей у цеху в разі аварійного горіння твердого ракетного палива. Методика. Для розрахунку поля температури в цеху, з метою визначення зон термічного ураження працівників у приміщенні, використано рівняння, що виражає закон збереження енергії. На базі цього моделювального рівняння розраховано поле температур у цеху за наявності джерела емісії тепла – твердого ракетного палива, що горить. Розрахунок поля швидкості повітряного потоку в цеху, з урахуванням розташування перешкод на шляху розповсюдження теплової хвилі, проведено на базі моделі безвихрового руху повітря – рівняння потенціалу швидкості. Чисельне розв’язання рівняння для потенціалу швидкості виконано за допомогою двокрокової скінченнорізницевої схеми умовної апроксимації. Для чисельного розв’язання рівняння енергії використано різницеву схему розщеплення. На першому етапі побудови різницевої схеми виконано розщеплення двовимірного рівняння енергії на систему одновимірних рівнянь. Кожне одновимірне рівняння дозволяє розрахувати зміну температури в одному координатному напрямку. Для визначення температури використано схему біжучого рахунку. Під час проведення обчислювального експерименту враховано повітрообмін у приміщенні. Оцінку ризику термічного ураження персоналу в приміщенні виконано для різних вірогідностей місця аварійного горіння твердого ракетного палива. Результати. За допомогою розробленої чисельної моделі виконано прогнозування зон потенціального ризику термічного ураження персоналу в цеху для різних аварійний ситуацій. Наукова новизна. Побудовано комп’ютерну модель для експрес-оцінки потенціального ризику термічного ураження людей в цеху в разі аварійного горіння твердого ракетного палива. Практична значимість. Розроблено код, що дозволяє швидко моделювати формування температурних полів у цеху у випадку аварійного горіння твердого ракетного палива, та на базі цієї інформації визначати зони потенціального термічного ураження працівників. Розроблена комп’ютерна програма може бути використана для оцінки ризику термічного ураження на підприємствах хімічної промисловості в разі виникнення аварійних ситуацій.

Ключові слова: чисельне моделювання; ризик ураження; аварійне горіння твердого ракетного палива; теплове забруднення повітря

Н. Н. БЕЛЯЕВ^1*, А. В. БЕРЛОВ^2*, В. в. беляева^3*, В. А. козачина^4*, И. В. калашников^5*

^1*Каф. «Гидравлика и водоснабжение», Днипровский национальный университет железнодорожного транспорта имени академика В. Лазаряна, ул. Лазаряна, 2, Днипро, Украина, 49010, тел. +38 (056) 273 15 09, эл. почта water.supply.treatment@gmail.com, ORCID 0000-0002-1531-7882
^2*Каф. «Безопасность жизнедеятельности», ГВУЗ Приднепровская государственная академия строительства и архитектуры, ул. Чернышевского, 24а, Днипро, Украина, 49600, тел. +38 (056) 756 34 57, эл. почта berlov@pgasa.dp.ua, ORCID 0000-0002-7442-0548
^3*Каф. «Аэрогидромеханика и энергомасоперенос», Днипровский национальный университет имени Олеся Гончара, просп. Гагарина, 72, Днипро, Украина, 49000, тел. +38 (056) 374 98 22, эл. почта water.supply.treatment@gmail.com, ORCID 0000-0003-2399-3124
^4*Каф. «Гидравлика и водоснабжение», Днипровский национальный университет железнодорожного транспорта имени академика В. Лазаряна, ул. Лазаряна, 2, Днипро, Украина, 49010, тел. +38 (056) 273 15 09, эл. почта v.kozachyna@gmail.com, ORCID 0000-0002-6894-5532
^5*Харьковское отделение филиала «Проектно-изыскательный институт железнодорожного транспорта» АО «Українська залізниця», ул. Котляра, 7, Харьков, Украина, 61052, тел. +38 (057) 724 41 25, эл. почта uzp38@ukr.net, ORCID 0000-0002-2814-380X

АВАРИЙНОЕ ГОРЕНИе твердого ракетного

топлива: ОЦЕНКА РИСКА ПОРАЖЕНИЯ

ЛЮДЕЙ В РАБОЧЕМ ПОМЕЩЕНИИ

Цель. Эта работа предусматривает разработку компьютерной модели для расчета риска термического поражения людей в цехе при аварийном горении твердого ракетного топлива. Методика. Для расчета поля температуры в цехе, с целью определения зон термического поражения работников в помещении, использовано уравнение, выражающее закон сохранения энергии. На базе этого моделирующего уравнения рассчитано поле температур в цехе при наличии источника эмиссии тепла – горящего твердого ракетного топлива. Расчет поля скорости воздушного потока в цехе, с учетом расположения препятствий на пути распространения тепловой волны, проведен на базе модели безвихревого движения воздуха – уравнения потенциала скорости. Численное решение уравнения для потенциала скорости выполнено с помощью двухшаговой конечноразностной схемы условной аппроксимации. Для численного решения уравнения энергии использована разностная схема расщепления. На первом этапе построения разностной схемы выполнено расщепление двумерного уравнения энергии на систему одномерных уравнений. Каждое одномерное уравнение позволяет рассчитать изменение температуры в одном координатном направлении. Для определения температуры использована схема бегущего счета. При проведении вычислительного эксперимента учтен воздухообмен в помещении. Оценку риска термического поражения персонала в помещении выполнено для различных вероятностей места аварийного горения твердого ракетного топлива. Результаты. С помощью разработанной численной модели выполнено прогнозирование зон потенциального риска термического поражения персонала в цехе для различных аварийный ситуаций. Научная новизна. Построена компьютерная модель для экспресс-оценки потенциального риска термического поражения людей в цехе в случае аварийного горения твердого ракетного топлива. Практическая значимость. Разработан код, позволяющий быстро моделировать формирования температурных полей в цехе при аварийном горении твердого ракетного топлива, и на базе этой информации определять зоны потенциального термического поражения работников. Разработанная компьютерная программа может быть использована для оценки риска термического поражения на предприятиях химической промышленности в случае возникновения аварийных ситуаций.

Ключевые слова: численное моделирование; риск поражения; аварийное горение твердого ракетного топлива; тепловое загрязнение воздуха

References

1. Alymov, V. T., & Tarasova, N. P. (2004). Tekhnogennyy risk. Analiz i otsenka: uchebebnoe posobie dlya vuzov. Moscow: Akademkniga. (in Russian)

2. Belyaev, N. N., Gunko, Y. Y., Kirichenko, P. S., & Muntyan, L. Y. (2017). Otsenka tekhnogennogo riska pri emissii opasnykh veshchestv na zheleznodorozhnom transporte. Krivoi Rog: Kozlov R. A. (in Russian)

3. Zgurovskiy, M. Z., Skopetskiy, V. V., Khrushch, V. K., & Belyaev, N. N. (1997). Chislennoe modelirovanie rasprostraneniya zagryazneniya v okruzhayushchey srede. Kуiv: Naukova dumka. (in Russian)

4. Marchuk, G. I. (1982). Matematicheskoye modelirovaniye v probleme okruzhayushchey sredy. Moscow: Nauka. (in Russian)

5. Samarskij, A. A. (1983). Teoriya raznostnyh skhem. Moscow: Nauka. (in Russian)

6. Berlov, O. V. (2016). Atmosphere protection in case of emergency during transportation of dangerous cargo. Sciance and Transport Progress, 1(61), 48-54. DOI: 10.15802/stp2016/60953 (in English)

7. Biliaiev, M. M., Berlov, O. V., Kozachina, V. A., Kalashnikov, I. V. & Shevchenko, O. V. (2020). Risk assessment of thermal damage to people at industrial sites in case of emergency of burning solid propellant. Sciance and Transport Progress, 1(85), 7-16. DOI: 10.15802/stp2020/200752 (in English)

8. Biliaiev, M. M., & Kharytonov, M. M. (2012). Numerical Simulation of Indoor Air Pollution and Atmosphere Pollution for Regions Having Complex Topography. NATO Science for Peace and Security. Series C: Environmental Security, 87-91. DOI: 10.1007/978-94-007-1359-8_15 (in English)

9. Boris, J., Patnaik, G., Obenschain, K., Moses, A., Obenschain, M.-Y., Theodore, Y., Delaney, J. & Donnelly, J. (2010). Fast and accurate prediction of windborne contaminant plumes for civil defense in cities. The Fifth International Symposium on Computational Wind Engineering (CWE2010), 1-9. (in English)

10. Cao, C., Li, C., Yang, Q., & Zhang, F. (2017). Multi-Objective Optimization Model of Emergency Organization Allocation for Sustainable Disaster Supply Chain. Sustainability, 9(11), 1-22. DOI: 10.3390/su9112103 (in English)

11. Ilić, P., Ilić, S., & Stojanović Bjelić, L. (2018). Hazard Modelling of Accidental Release Chlorine Gas Using Modern Tool-Aloha Software. Quality of Life (Banja Luka)-APEIRON, 16(1-2), 38-45. DOI: 10.7251/QOL1801038I (in English)

12. Komatina, D. Ilić, Galjak, J., Belošević, S. (2018). Simulation of chemical accidents with acetylene in «messer tehnogas» kraljevo plant by «aloha» software program. The University Thought-Publication in Natural Sciences, 8(2), 19-26. DOI: 10.5937/univtho8-18014 (in English)

13. Lacome, J. M., Truchot, D. & Duplantier S. (2017). Application of an innovative risk dedicated procedure for both conventional and 3D atmospheric dispersion models evaluation. 8 International Conference on Harmonisation within Atmospheric Dispersion Modelling for Regulatory Purposes (HARMO 18) (pp. 821-825). Bologne, Italy. (in English)

14. Lee, H., Sohn, J.-R., Byeon, S.-H., Yoon, S., & Moon, K. (2018). Alternative Risk Assessment for Dangerous Chemicals in South Korea Regulation: Comparing Three Modeling Programs. International Journal of Environmental Research and Public Health, 15(8), 1-12. DOI: 10.3390/ijerph15081600 (in English)

15. Sideris, G. M., Christolis, M. N., Markatos, N. C. (2020). Numerical simulation of pollutants dispersion around an electric-arc-furnace in case of an accidental release. Biodiversity International Journal, 4(1), 50-57. DOI: 10.15406/bij.2020.04.00164 (in English)

Received: January 30, 2020

Accepted: June 02, 2020

Creative Commons Attribution 4.0 International © M. M. Biliaiev, O. V. Berlov, V. V. Biliaieva,

doi: 10.15802/stp2020/208097 V. A. Kozachyna, I. V. Kalashnikov, 2020