ISSN
2307–3489 (Print), ІSSN
2307–6666
(Online)
Наука та прогрес
транспорту. Вісник
Дніпропетровського
національного університету залізничного
транспорту, 2019,
№ 4
(82)
ЗАЛІЗНИЧНА
КОЛІЯ ТА АВТОМОБІЛЬНІ ДОРОГИ
ЗАЛІЗНИЧНА
КОЛІЯ та автомобільні дороги
UDC
629.4.015:625.1.032
I. O.
BONDARENKO1*,
L. О. NЕDUZha2*
1*Dep.
«Track and Track Facilities», Dnipro
National University
named after
Academician V.
Lazaryan, Lazaryan St.,
2, Dnipro,
Ukraine,
49010, tel.
+38 (063) 802 21 80,
e-mail
irina_bondarenko@ua.fm, ORCID 0000-0003-4717-3032
2*Dep.
«Theoretical and
Structural Mechanics»,
Dnipro
National
University named after Academician V. Lazaryan,
Lazaryan St., 2,
Dnipro, Ukraine, 49010,
tel. +38 (067) 810 51 65, e-mail
nlorhen@i.ua,
ORCID
0000-0002-7038-3006
INVESTIGATION OF THE influence
OF THE ROLLING STOCK DYNAMICS
ON
THE INTENSITY OF USING
THE RAILWAY TRACK ELEMENTS
Purpose. The
main purpose of this work is to research deformability parameters of
the railway track under various operating conditions as the initial
data for the formation of forced oscillations of rolling stock and
the trackform use intensity. Methodology.
For the research, an original model of the trackform,
described with using the basic concepts of the theory of elasticity
and the propagation of elastic waves was developed. Findings.
It has been established that the ratio between the intensity of
work of elements and the trackform, as criteria for deformability,
can be used as the estimated parameters of the functionally safe
operation of the track. It can be the basis for the classification of
critical track states under which it had to perform work to restore
its working capacity in order to ensure the required level of
efficiency of use construction during the service life.
Originality. Trackform
and parameters of the elements of the track superstructure and
substructure can be described using the railway tracks deformability
behaviour. In the future this conclusion will help to increase the
efficiency of the dynamic properties of rolling stock when designing
and maintain in readiness the infrastructure while in operation.
Practical value. Changes in the regulatory framework of
rail transport in recent years provide for the observance of its
functional safety, therefore, the question of the need to assess the
impact of rolling stock on the way to be regarded as a dynamic
process with consideration to the deformability resistance track.
This research provides a basis for record of the stiffness of
deformation that allows creating the regulatory framework for the
functional safety of the railway track in Ukraine.
Keywords: trackform;
deformability parameters; oscillation;
deformability of the railway track
Introduction
The issue of providing the
reliable and safe operation of the railway track and rolling stock
during their interaction has existed since the introduction of
railway transport. The requirements for the said objects of
interaction were forming gradually and depended on the level of
knowledge and technologies. Thus, before introduction of rails of
Р50, UIC60, Р65 types and reinforced concrete sleepers in the
trackform, the main requirements were the provision of railway track
element strength and the track panel stability under the influence
of increasing loads and speeds of the rolling stock. The use of the
aforesaid track structure components completely provided the
superstructure strength under the weight of a rolling stock.
From 70s of the last century, the new requirements were forming, and
it has resulted in approving the international standards such as IEC
62278:2002, EN 50126-1-2017, EN 50126-2-2017 and IEC 60300-3-3:2017.
The modern
requirements for transport system maintenance strategy are directed
for minimizing the lifecycle cost, and for maximizing the
reliability, availability, maintainability and safety (RAMS) of the
transport systems. All the issues related to defining the
functionally safe operation of transport systems imply the study and
evaluation of dynamic processes resulting from the interaction in
time between the railway track and rolling stock. The influence of
dynamic processes in time becomes decisive while assessing the
functionally safe operation of modern speed projects such as «Aero
Train» (Yasuaki
Kohama), «Hyperloop»
(Elon Musk). In order to ensure the functionally safe operation of
any transport system, it is required to know which changes occur in
its elements in time and how the functionally safe status of each
element as well as their aggregate affect the system’s operation.
Indeed, in order to predict the operation of subgrade under actual
climate and service conditions, it is required to consider in time
the process of permanent deformation accumulation in it. In other
words, it is required to figure out the following issues: a) which
changes occur in the subgrade as a result of multiple force impact
by a rolling stock with respect to the change in temperature and
soil moisture; b) how the process of change in temperature and
moisture in the subgrade occurs under the influence of force,
electromagnetic and gravitational fields.
The influence of force fields
in the hard texture elements is researched insufficiently. The study
of stress-strain state of the railway track elements results in
identifying the peak stresses and deformations while using the full
force value. The deformability of the railway trackform as the
aggregate of deformations and shifts is presented as an influence
line (field, when the finite element method is used). It is
considered that the influence line shifts together with the force
motion. In all the mathematical models of railway track calculation,
it is taken that the influence of force field spreads evenly in all
directions, i.e. the principle of force field transfer is used due
to longitudinal waves how it occurs in liquid and gaseous media. The
influence of longitudinal waves is not taken into account in the
models. It may cause the error during calculation because it does
not allow reflecting the physical process of transferring the force
fields in the solid bodies and between them.
While
running the test on the rolling stock’s influence on the railway
track, a huge number of statistical data on strain-stress state of
the track have been obtained. They describe different deformability
of the same length of the track under the influence of the same
rolling stock. The different statistical data are conditioned by the
process of mutual influence of the rolling stock and the railway
track. We consider next the issue of analyzing the parameters of
railway track deformability under various operating conditions as
the initial data for forming the forced oscillations of the railway
rolling stock and the intensity of using the trackform elements.
Purpose
The main purpose of this work
is to study deformability parameters of the railway track under
various operating conditions as the initial data for the formation
of forced oscillations of rolling stock and the intensity in the use
of elements of the trackform. The object of the study is the process
of mutual influence of the railway rolling stock and the track. The
subject of the study is the deformability of the railway track under
various operating conditions as a factor in the formation of dynamic
irregularities.
Methodology of investigation
The main
macro indicators of the operation of the railway transport
infrastructure are the availability and economic efficiency
coefficients. Currently, many studies have been devoted to the
problems of studying the mathematical description of perturbations
acting on the track and the crew during their interaction [2-3,
5-23]. Each article adds an important part of the overall process of
the track and
rolling stock interaction. Articles
[2, 5-6, 10,
12, 13, 15-17,
20, 21-23]
focus on analysing
the simulation results delivered with implementation of the
mathematical models of the rolling stock: for transport carriages
[2, 5, 22], for real locomotives [6, 13, 16, 20,
21], for locomotive-roller rig coupled
system [9], for general rail vehicle [10, 12, 15, 17, 23]. Paper [3]
describes the mechanism of transferring force from the rolling stock
to the trackform. The simplified method to determine a vertical
impact force of the wheel with flat and rail interaction is
presented in this article. This article demonstrates the influence
of the wheel with flat geometrical parameters, speed of a vehicle to
maximum contact force and its distribution in the contact zone.
Articles [7, 11,
14, 16, 18, and 19] are dedicated to studying the track and the
rolling stock interaction.
The numerical model [7] is
subsequently used to determine the track vertical and lateral
stiffness. The work [11] proposes a computational methodology to
study how the varying vehicle component characteristics, on normal
and degraded conditions, impact on the vehicle/track interaction
loads and on the track damage. The paper [14] proposes an approach
for the validation of railway vehicle models based on on-track
measurements. The tasks that are addressed in the research [18]: to
perform tests at track irregularities of symmetrical sinusoidal
shape running both along straight and curved tracks, as well as
driving over the junctions along the straight and curved tracks, and
compare the results. A novel wheel–rail contact model [19] is
proposed to be implemented for multi-body dynamics simulation, in
order to facilitate accurate online calculation of damage phenomena
such as wear and rolling contact fatigue.
The above
articles studies are very important issues, but they apply some of
the approaches that restrict their research.
Modern theoretical models, which study the
track and rolling stock interaction, means and methods of recording
oscillations used in experiments, while studying the track and
rolling stock interaction, or assessing geometrical track states,
have no mechanism for determining the source of vibration and the
nature of the process of transferring these vibrations to the
«rolling
stock-track system».
Consequently, the oscillograms of the railway track contain the
superposition of both the impact of the rolling stock on the track
and the response of the track, taking into account its
deformability. The oscillograms on the rolling stock contain a
superposition of both the impact of the rolling stock on the track
and the other way round. These perturbations are not classified
according to physic-mechanical and geometric characteristics of
railway track construction elements that determine its
deformability. Thus, changes in the conditions of rails and wheels
contact with their tortuous motion remain unconsidered. Therefore,
firstly, it has become necessary to study the mechanism of
transferring force from the rolling stock to the trackform and vice
versa in time. Secondly, there was a need to study the physics of
the oscillation process and to differentiate the vibrations from the
physical-mechanical and geometric characteristics of the elements of
the construction of the railway track, which determine its
deformability.
Based on the
experience gained, a model was developed for determining the
processes of deformative operation of the railroad track [1, 4,
8].
The original model was created
for investigating the dynamic problem of railway track deformability
under the influence of the rolling stock. It applies the basic
provisions of the theories of elasticity and propagation of elastic
waves.
In the
model, it is taken that the track and rolling
stock interaction occurs by means of impulses. The impulses are
transmitted via bond pads conditioned by the position of a wheel set
along the track. The impulse duration is characterized by the time
of transferring the load from the rolling stock to the rail and
depends on the rolling stock speed. Any dynamic force is
characterized by the impulse transmission frequency on a particular
bond pad and by the impulse repetition frequency in particular
section [1, 4, 8].
The impulses are characterized by the law of force change and the
law of force intensity change in a contact time. A local system of
force coordinates for each
propagation of oscillations is always positioned in such a way so
that the x axis
coincides with the direction of force motion and the
 axis – direction of force action. An explanation to the directions
and angles is given in Fig. 1.
axis – direction of force action. An explanation to the directions
and angles is given in Fig. 1.
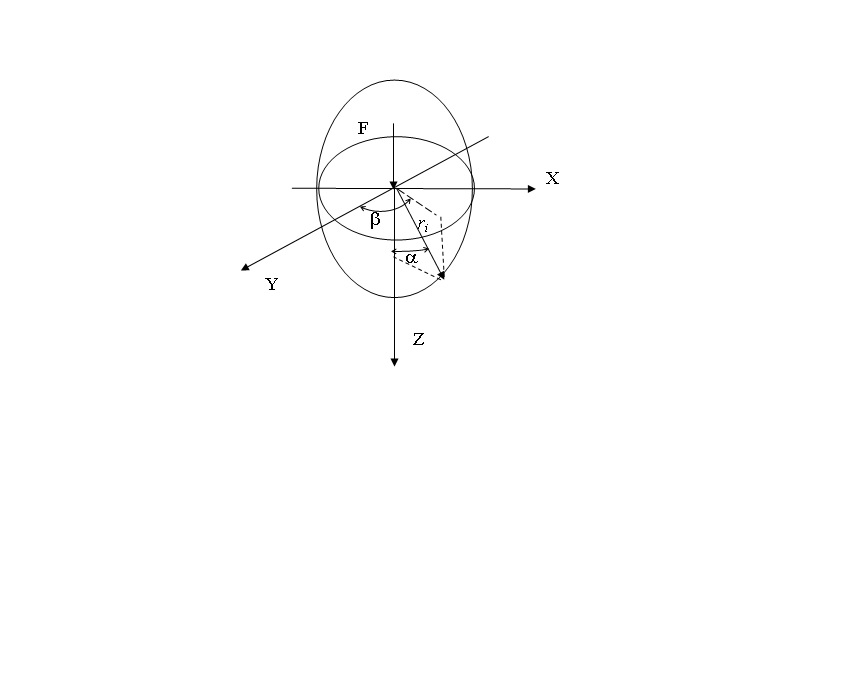
Fig. 1. Determining the directions and angles of
oscillations propagation
Components of the wave vector
along the axes of coordinates that define the plane of equal phases
and amplitudes of oscillatory motion depend on the position of a
local coordinate system of the force relative to the basic
coordinate system of the structure. Thus, the position of a local
coordinate system of the force relative to the basic coordinate
system of the structure defines both the directions of propagation
of longitudinal and transverse waves and polarization of these
waves.
The impulses are transmitted by
the force waves. The process of force wave propagation is described
by using the propagation properties of transverse and longitudinal
waves. All oscillations generated by the contacting surfaces, which,
up to the given point, have not touched or have renewed their
contact after a break, propagate by spherical waves. They
characterize the main direction of propagation of the wave process
from a new or renewed contact between surfaces and account for the
contact and local concentrations of stresses and deformations.
All oscillations generated by
the contacting surfaces, which, up to the given point, have touched
and established contacts, propagate by quasi spherical waves. They
characterize the basic direction of propagation of the wave process
from the contact point of the surfaces and account for the
non-uniformity of oscillating. But one spherical wave of incidence
that carries the longitudinal and transverse mode causes four quasi
spherical refracted waves: two longitudinal and two transverse ones.
Each of them is heterogeneous, as it has vivid dependence of the
change in characteristics on its direction and carries the
consequences from the neighboring refracted waves in other
directions.
Since in the
process of propagation there is the superposition of waves, it
characterizes the non-uniformity of the whole process of
oscillating. Thus, in every point of the structure in a certain
period of action, one will observe either homogeneous spherical or
non-uniform quasi spherical waves. Their availability and velocity
of propagation are conditioned by stress-strain properties of
element materials. Each wave affects the particle in different
directions with respect to the direction of wave propagation. Each
wave, while transiting from one element to the other, changes its
properties. So, the calculation in the model is done with respect to
the directions of force wave front propagation
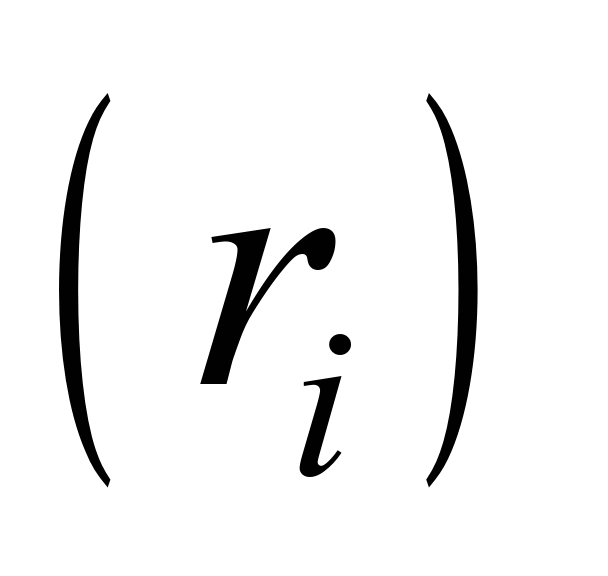 .
The main dependences of motion-direction are shown in [1, 4]. As a
result, the shift of structural particles in time has been obtained
.
The main dependences of motion-direction are shown in [1, 4]. As a
result, the shift of structural particles in time has been obtained
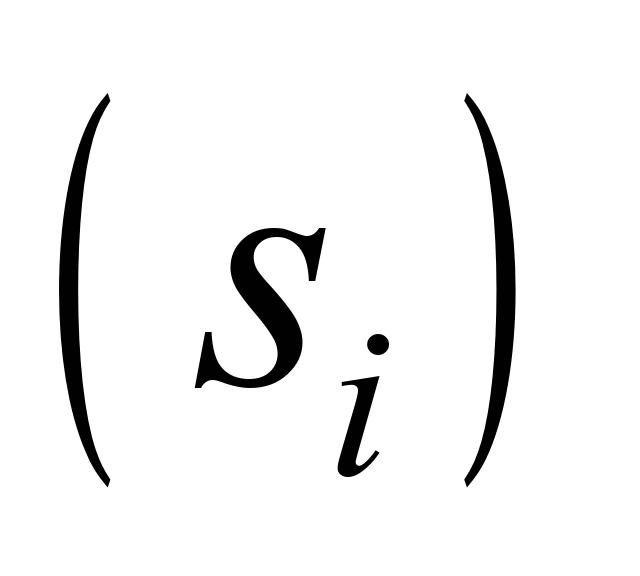 (see Fig. 2-5).
(see Fig. 2-5).
We compared
the values of the stresses at the rail base edge and head and the
rail deflections caused by running of ChS8 locomotive at a speed of
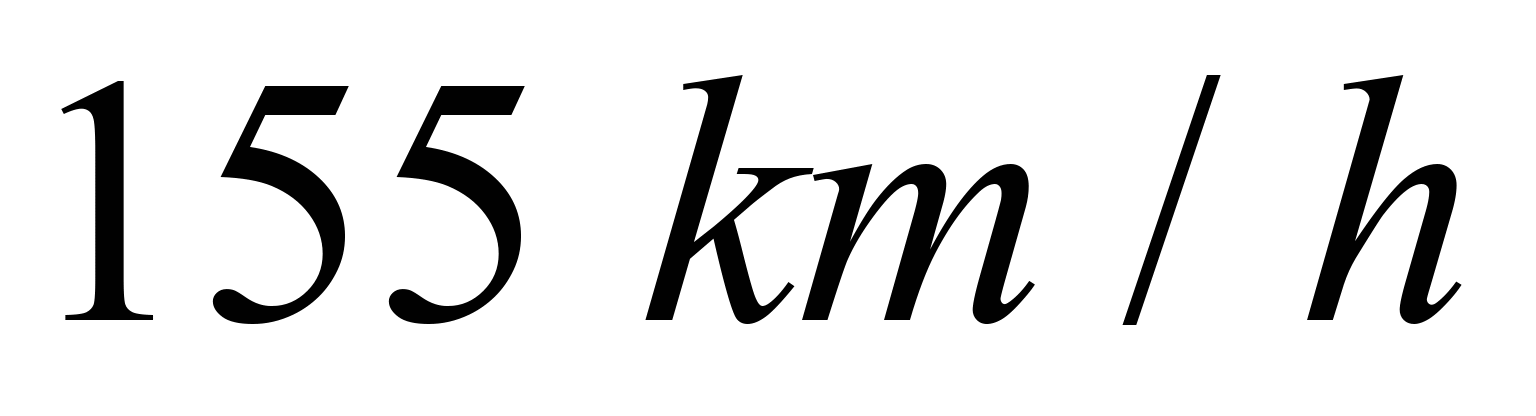 .
The experiment was carried out on a single-track section. The
trackform elements: UIC60 rail track, reinforced concrete sleepers
with KPP-5 type fastening,
.
The experiment was carried out on a single-track section. The
trackform elements: UIC60 rail track, reinforced concrete sleepers
with KPP-5 type fastening,
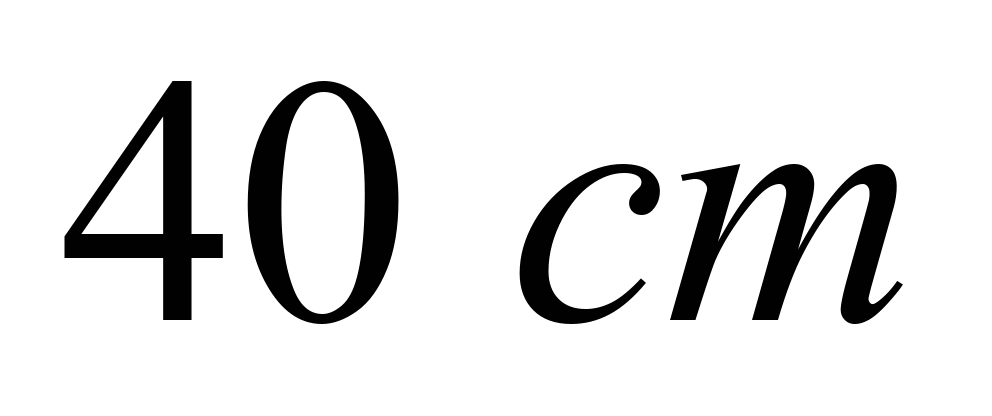 ballast depth, the roadbed is a sandy loam subgrade. The modulus of
the track elasticity in the vertical plane for calculation by the
existing method was taken from the experimental data and made
ballast depth, the roadbed is a sandy loam subgrade. The modulus of
the track elasticity in the vertical plane for calculation by the
existing method was taken from the experimental data and made
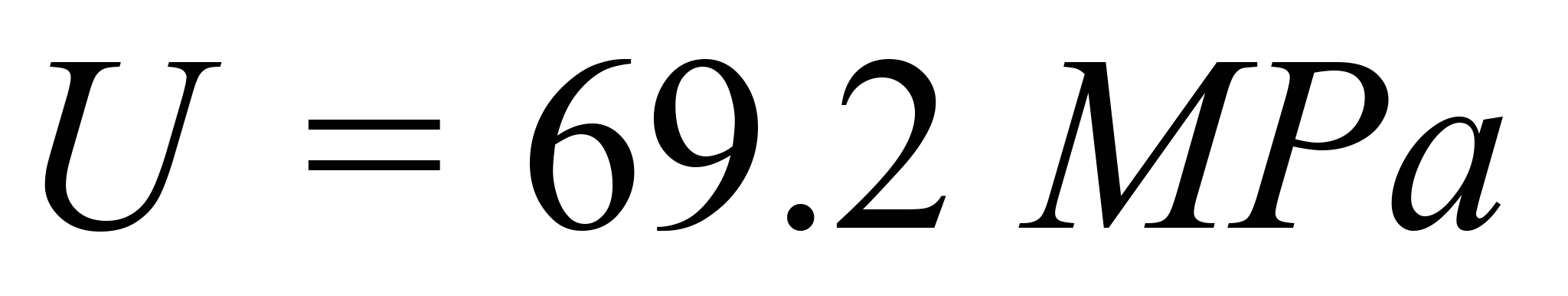 .
Table 1 shows the data calculated by the existing method «Track
strength calculation»,
the proposed method and the experiment obtained ones.
.
Table 1 shows the data calculated by the existing method «Track
strength calculation»,
the proposed method and the experiment obtained ones.
Table
1
Data
of the track stress-strain state
obtained using various methods
and experimentally
|
Method
|
Stresses
(MPa) in rail
|
Rail
deflection, mm
|
|
Base edge
|
Head
|
|
The existing method
|
115.7
|
120.69
|
1.56
|
|
The
proposed method
|
159.5
|
145.9
|
2.81
|
|
Experiment
|
163.3
|
146.1
|
2.5
|
Based on the strain-stress
properties and geometrical characteristics of the elements, the use
of wave properties allows establishing: a) the reaction of elements
to the force impact with respect to the time of its propagation; b)
time ratio of impulse impact and its propagation along the length
and depth of the trackform; c) change in amount of energy in any
point of the structure in time. The aforementioned parameters allow
changing the deformation processes by altering the element geometry
and material properties.
Based on the
described mathematical model, it is proposed to take as a composite
index the deformable work of trackform elements
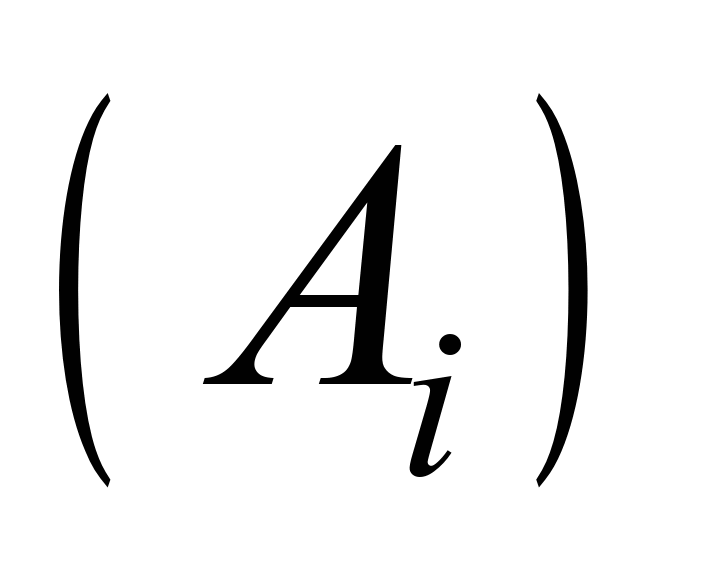 :
:
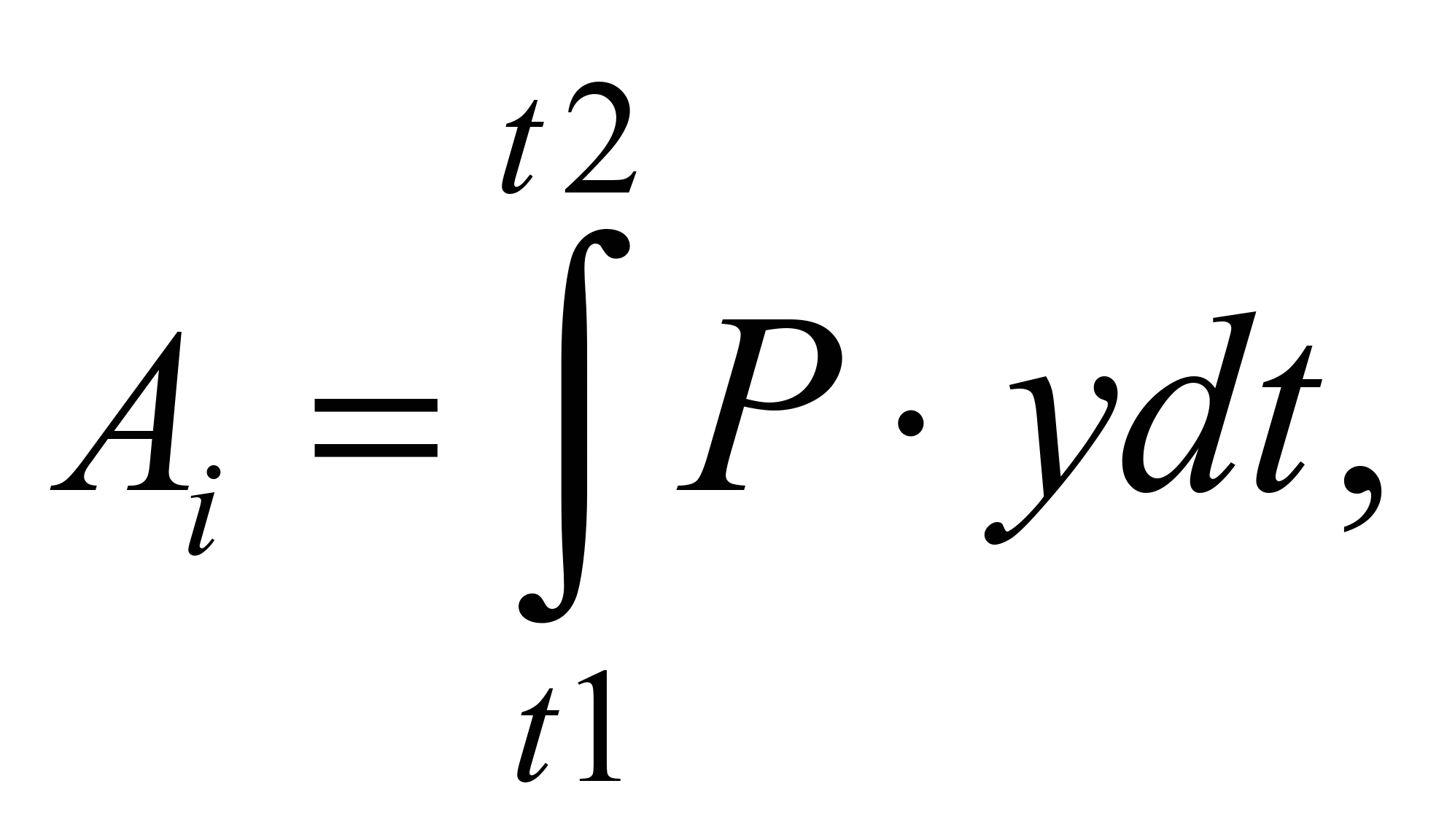 (1)
(1)
where
 – force that affects the element;
– force that affects the element;
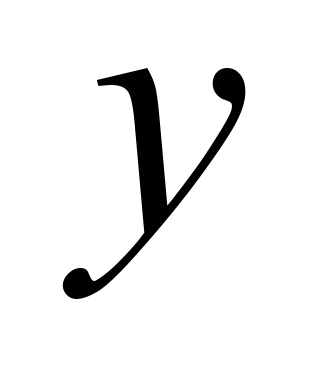 – shift caused by the force during the action [
– shift caused by the force during the action [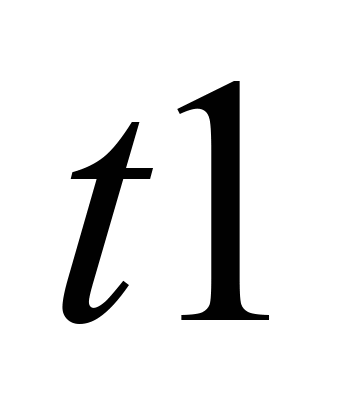 ,
,
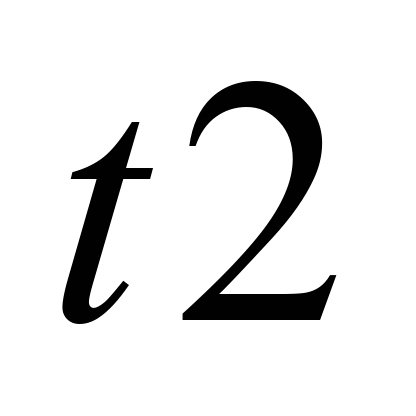 ].
].
The amounts
of shift have three-dimensional nature even for one section of the
trackform. So, it was proposed to define the deformable work of
trackform
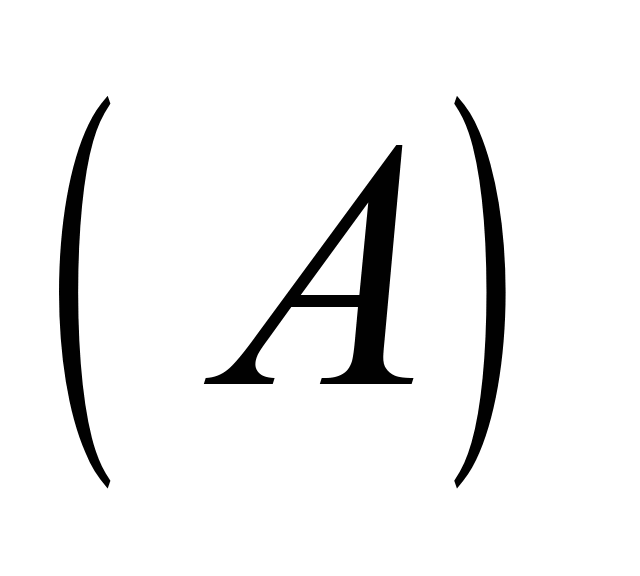 as the sum of works performed by all the track elements in one
section under the influence of the rolling stock with respect to the
time of perception and reaction of the elements:
as the sum of works performed by all the track elements in one
section under the influence of the rolling stock with respect to the
time of perception and reaction of the elements:
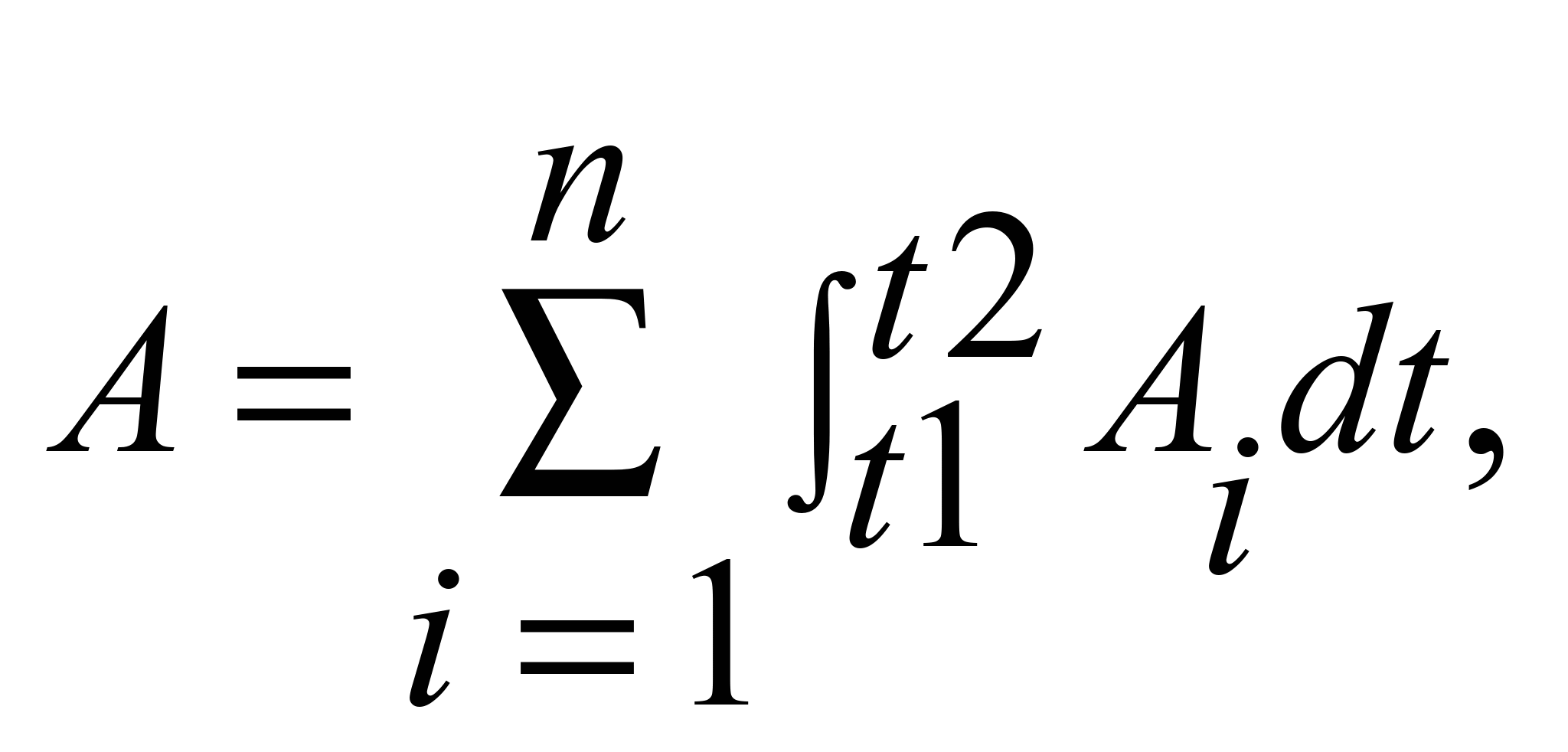 (2)
(2)
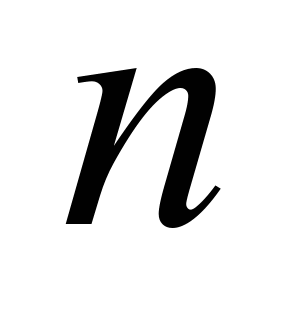 – a number
of elements getting a force impact.
– a number
of elements getting a force impact.
For evaluating and comparing
the use of trackform and elements under different operating
conditions, it was proposed to use I – the intensity of deformable
work of the elements or the structure (deformable work in the unit
of time).
The
investigation is carried out for the trackform model consisting of
the following elements such as rails of R65 type, concrete sleepers
of SB3 type, fastenings of KPP-5-К, ballast stone of
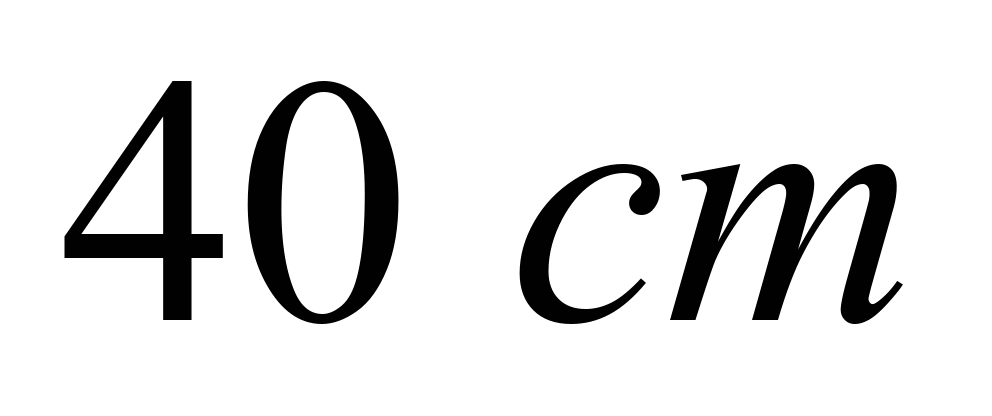 thick, subgrade. They are given in Table 2.
thick, subgrade. They are given in Table 2.
Table
2
The properties of trackform elements
|
Element
|
Density,
kg/m3
|
Poisson's coefficient
|
Young's module Е, МPa
|
Cl, m/s 1
|
Ct, m/s 2
|
|
rail
|
7 830
|
7 830
|
0.24
|
0.3
|
2.1х105
|
2.1х105
|
5 622
|
6 008
|
3 288
|
3 211
|
|
pad
|
918
|
935
|
0.3
|
0.485
|
100
|
200
|
382
|
1 572
|
204
|
268
|
|
sleeper
|
2 200
|
2 500
|
0.1
|
0.15
|
36 000
|
40 000
|
4 090
|
4 110
|
2 727
|
2 637
|
|
ballast
|
1 900
|
2 200
|
0.2
|
0.2
|
100
|
400
|
241
|
449
|
148
|
275
|
|
ground base
|
170
|
175
|
0.3
|
0.35
|
30
|
40
|
487
|
605
|
260
|
290
|
1
–
the speed of longitudinal; 2
–
the speed of transverse waves in the material
The rolling stock influence on
the track was considered with respect to the central position of the
wheel set. As a result of investigation, the following dependences
were obtained, which are shown in Fig. 2-3.
The upper
part of the diagram demonstrates the dependence of forces having an
impact on the track with respect to the time at various motion
speeds. The values of forces are given against the force value at
the speed of
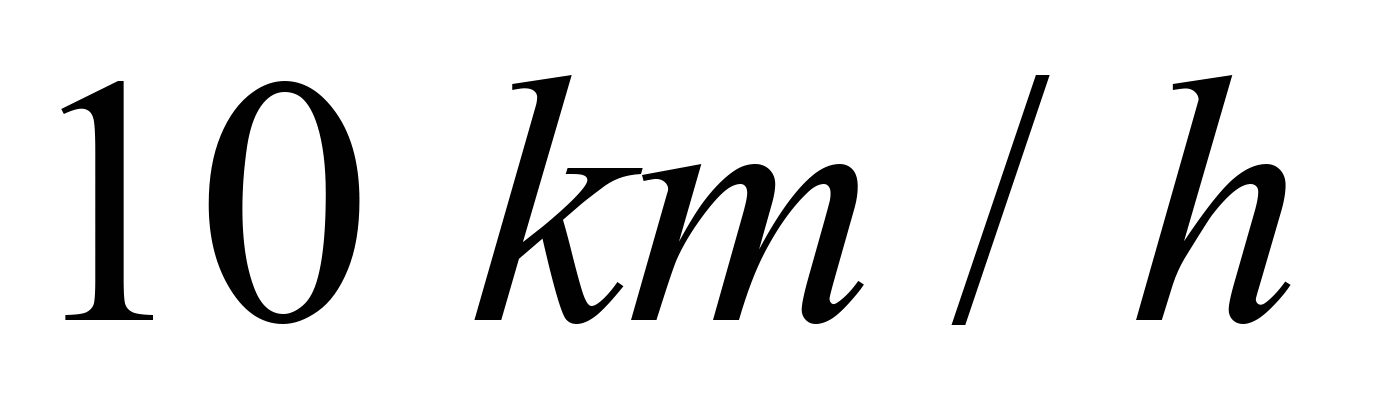 .
The lower part of the diagram demonstrates the dependence of
oscillation amplitudes of the trackform section in time with respect
to different motion speeds under the single force impact on the
section.
.
The lower part of the diagram demonstrates the dependence of
oscillation amplitudes of the trackform section in time with respect
to different motion speeds under the single force impact on the
section.
In the existing models, it is
assumed that the trackform deformability as a result of the rolling
stock influence occurs instantly. Figures 2 and 3 clearly
demonstrate the falsehood of such assumption, depending on the time
of impulse impact conditioned by the motion speed of the rolling
stock.
The time of occurrence of the
deformability process in the railway trackform due to the rolling
stock impact depends on two constituents. The first constituent is
the time of impulse impact that depends on the motion speed. Its
value decreases together with the increase in motion speed. The
second constituent is the time of propagation of force impact in the
trackform elements. It depends on stress-strain and geometrical
characteristics of trackform elements. For the same trackform, in
case of the same-type force impact (the equal direction of force and
place of its application), this value is constant. Therefore, in
case of increase in motion speed, the time of deformability process
running in the trackform approaches the value of second constituent.
With increase in motion speed, we may observe the increase in
temporary delay value between the time of impact on the trackform
and the time when deformability occurs.
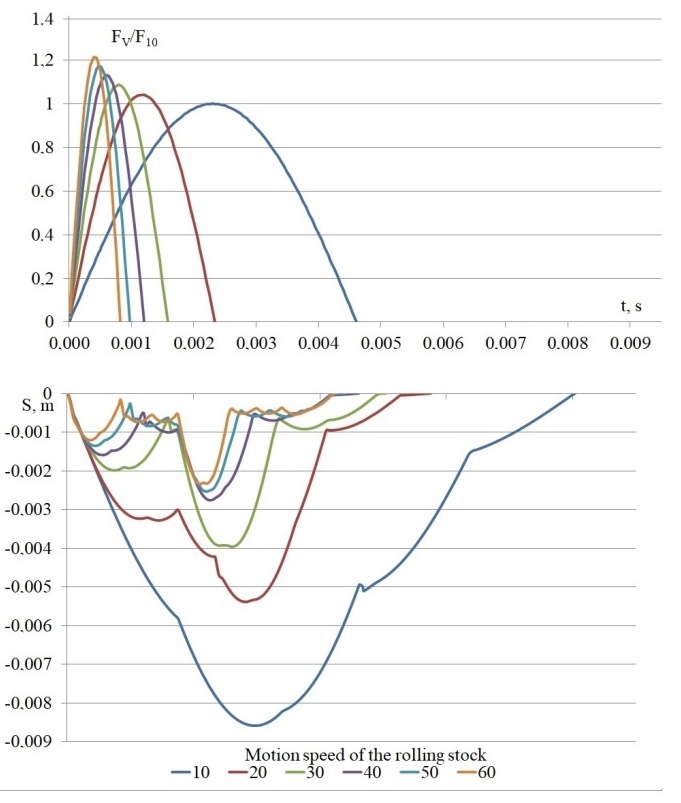
Fig. 2. The dependence of oscillation amplitudes
of
the trackform section in time with respect to different motion
speeds under the impact on the section
of single force

Fig. 3. The dependence of oscillation amplitudes
of
the trackform section in time with respect
to different motion
speeds under the impact
on the section of single force
The dependences of oscillation
amplitudes of trackform section in time for various motion speeds
under the single force impact on the section are shown in Fig. 4 and
5, which are similar to Fig. 2. The difference in diagrams is
caused by the difference in force values having an effect on the
track. With increase in action force, the direct variation of
dependences of oscillation amplitudes of trackform section in time
is not traced in diagrams. The form of oscillations changes together
with the change in oscillation amplitudes. The form of oscillation
towards the direction of force impact propagation changes in
propagation time and is connected with the correlation between the
force action time and the time of deformability process propagation
in the trackform elements.
The number
of energy transferred by the wave is proportional to the square of
oscillation amplitudes. The change in energy occurs due to the
following conditions: a) changes in oscillation characteristics
towards directions of wave propagation; b) changes in oscillation
characteristics with respect to the wave properties during their
transition from one element to the other; с) friction forces in the
very oscillation propagation medium; d) friction forces arising
during interaction of element contact pairs. For the same trackform
and with the same-type force action, the change in energy occurs due
to condition а; the rest of conditions change proportionally to the
change in force. This condition а preconditioned the change in the
depth of trackform required for taking up a load. Conditions
 are changing the form of dependences only in case of changing the
trackform. However, condition b
is decisive for choosing the number of elements and characteristics
of element materials in the trackform.
are changing the form of dependences only in case of changing the
trackform. However, condition b
is decisive for choosing the number of elements and characteristics
of element materials in the trackform.
The
aforesaid shows that the change in power loads and motion speeds of
the rolling stock directly affects the process of track
deformability. This process is one of the main factors for the
formation of dynamic track irregularities which serve as disturbance
effect for forming the three-dimensional vibrations of the rolling
stock. Each variety of trackform has its own time of force impact
propagation in the trackform elements. The change in number of
trackform elements, stress-strain properties, geometrical and
inertial characteristics of the trackform elements can cause the
change in time of propagation of the deformability process.
Therefore, we
can trace the interdependence of the rolling stock dynamics and the
process of track deformability.

Fig. 4. The dependence of oscillation amplitudes
of
the trackform section in time with respect
to different motion
speeds under the impact
on the section of single force


Fig. 5. The dependence of oscillation amplitudes
of
the trackform section in time with respect to different motion
speeds under the impact on the section
of single force
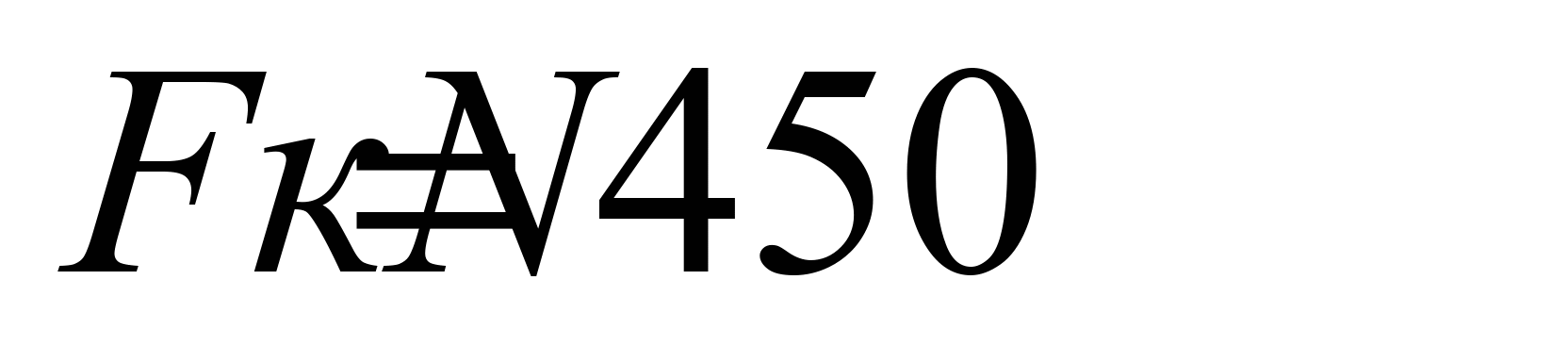
The track
deformability is accompanied by the trackform element work to take
up the force impacts from the rolling stock and to react to this
impact. Each element engages in the process of deformability from
the moment the force waves begin impacting on it. Each element has
its own time of propagation and transformation of force waves which
is conditioned by the stress-strain properties and geometrical
characteristics of the material. The trackform structure
characterizes the time of direct presence of force waves in the
trackform elements. Therefore, there exists the time when the
element directly takes and transforms force impacts, and the time
when the element becomes deformed due to deformations and shifts of
the other elements. In other words, the structure elements can work
«actively»
(by transforming the force impacts) and «passively».
For the purpose of evaluating
the operation of the above structure elements, we calculated the
dependences of oscillation amplitudes of the elements and trackform
section in time for various motion speeds during single force
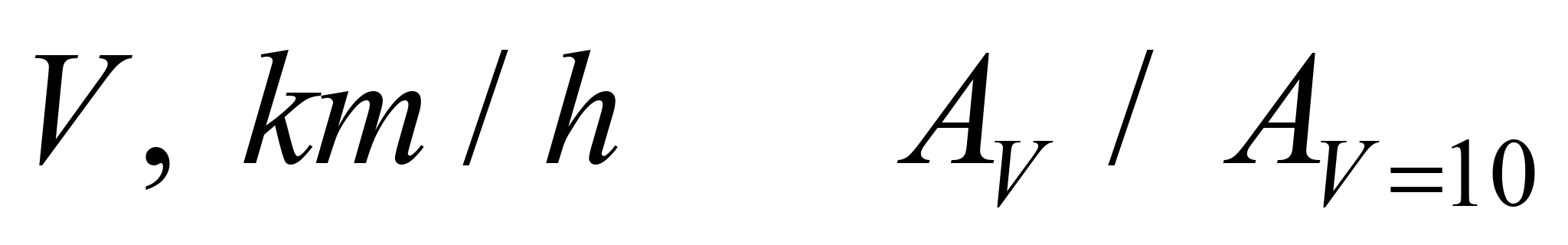
movement (wheel motion). Table 3 shows the relationship between the
values of the deformability behavior of the elements and the
trackform at various motion speeds.
Table
3
Deformability behavior ratios at various
motion
speeds
|
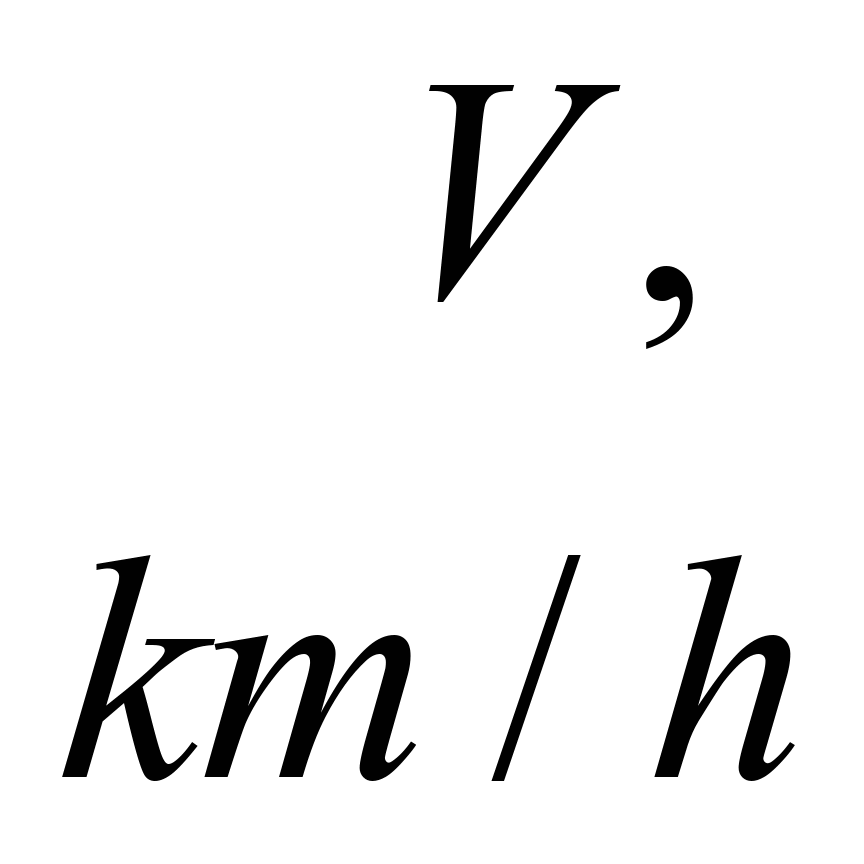
|
structure
|
pad
|
sleeper
|
ballast
|
ground base
|
|
|
|
10
|
1
|
1
|
1
|
1
|
1
|
|
30
|
0.17
|
0.18
|
0.09
|
0.469
|
0.134
|
|
60
|
0.03
|
0.08
|
0.03
|
0.121
|
0.044
|
|
90
|
0.02
|
0.07
|
0.02
|
0.055
|
0.025
|
|
120
|
0.01
|
0.06
|
0.01
|
0.044
|
0.018
|
|
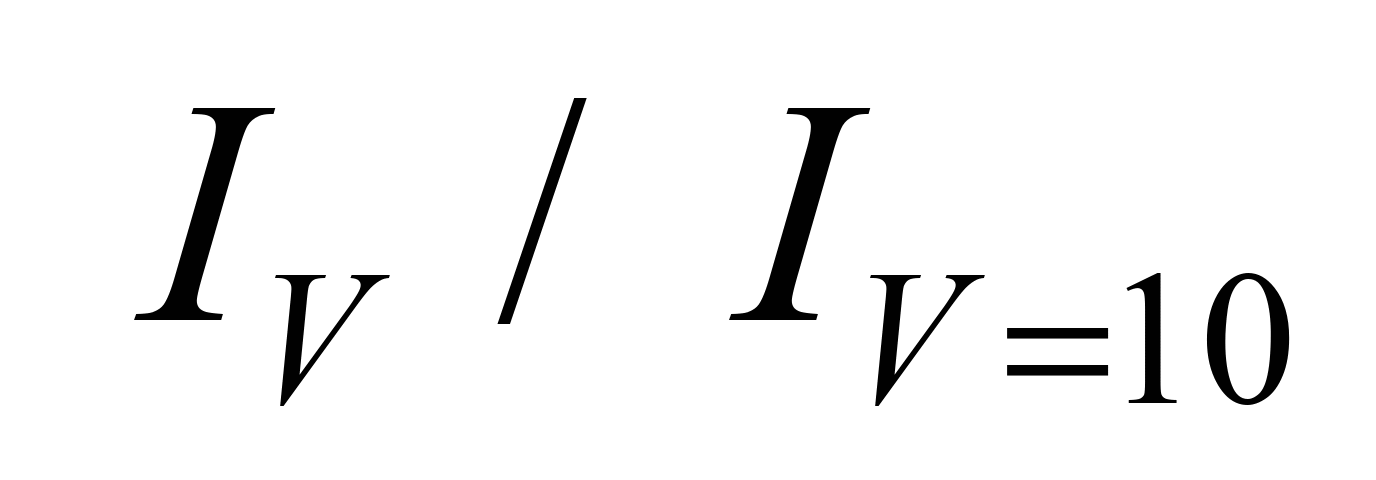
|
|
10
|
1
|
1
|
1
|
1
|
1
|
|
30
|
0.487
|
0.513
|
0.255
|
1.088
|
0.391
|
|
60
|
0.159
|
0.498
|
0.159
|
0.679
|
0.245
|
|
90
|
0.141
|
0.565
|
0.129
|
0.444
|
0.202
|
|
120
|
0.133
|
0.655
|
0.117
|
0.466
|
0.184
|
Following the results of data
analysis given in Table 3, at lower motion speeds, the values of
trackform amplitudes and load impact time exceed the similar values
at higher motion speeds but the load-receiving track length is
shorter. It results in different deformability behavior ratios at
various motion speeds. The evaluation of the trackform and elements
work intensity during the time of load action characterizes the
trackform and elements work intensity at various motion speeds. The
increase in motion speeds results in the intensity of using the rail
pads and railway ballast due to increased load-receiving track
length against the background of total decrease in the intensity of
using the trackform.
Table 4 shows the relationship
between the values of the deformability behavior of the elements and
the trackform at various loads.
Table 4
Deformability behavior ratios
at various loads
|
Object
|
load
|
|
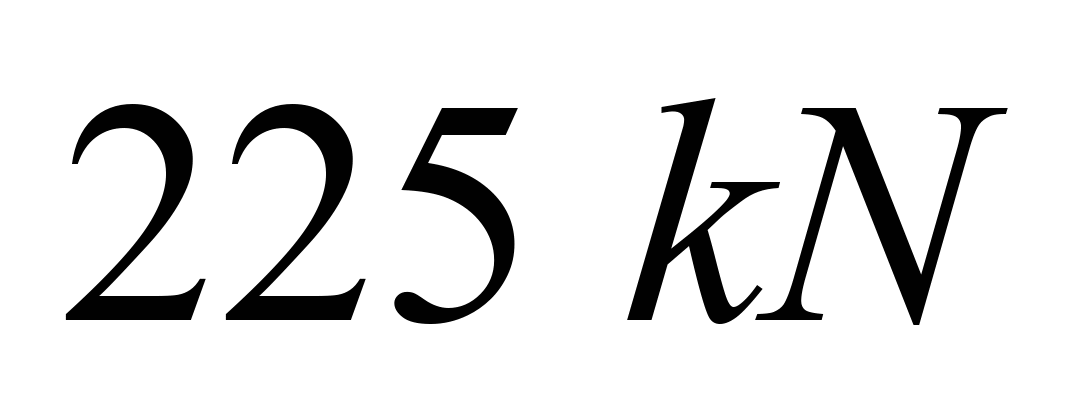
|

|
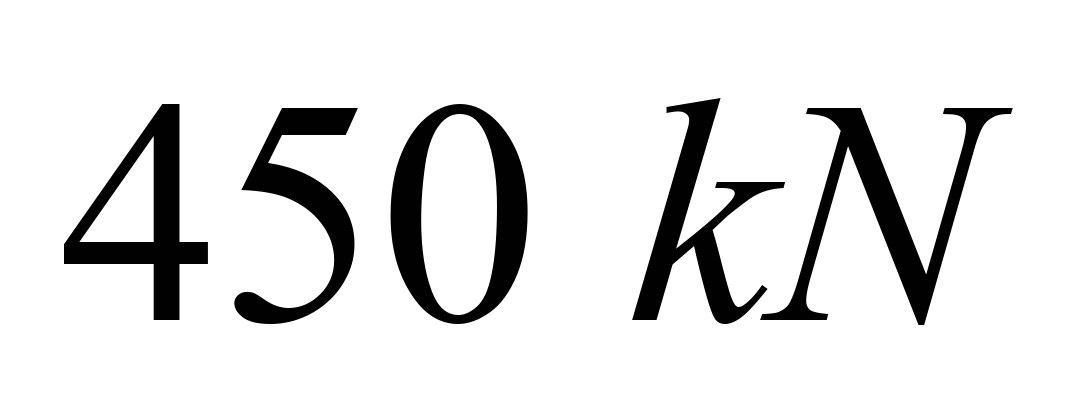
|
|
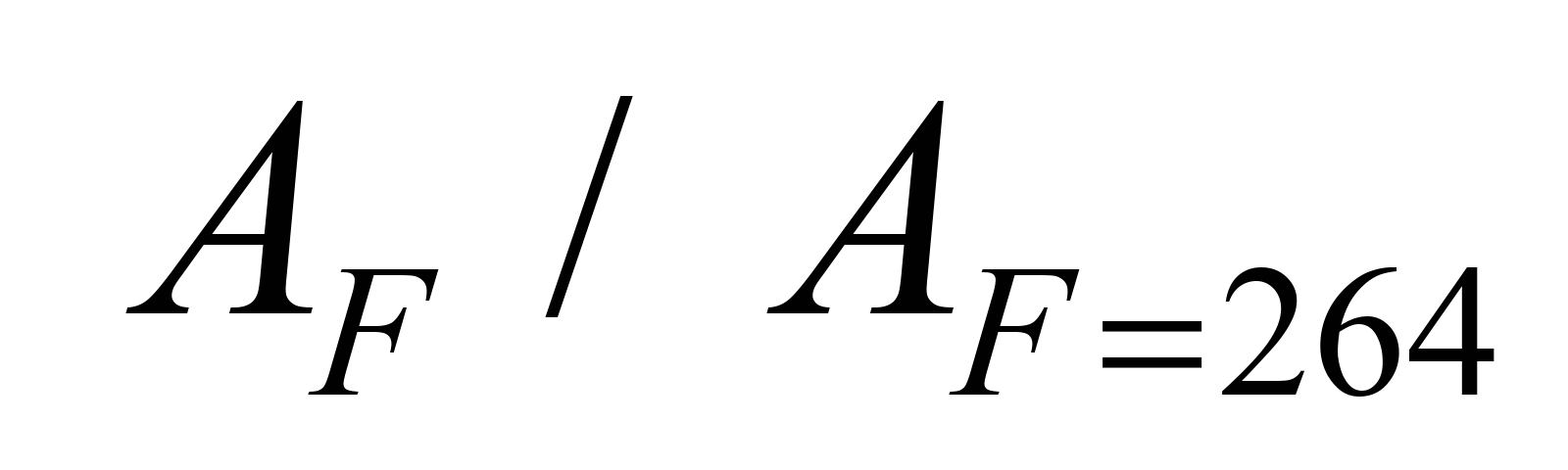
|
|
structures
|
1
|
2.21-2.34
|
7.1-7.63
|
|
pad
|
1
|
2.02-2.25
|
5.67-7.03
|
|
sleeper
|
1
|
2.17-2.19
|
6.25-6.83
|
|
ballast
|
1
|
2.18-2.43
|
6.49-8.13
|
|
ground base
|
1
|
2.18-2.48
|
6.42-6.85
|
|
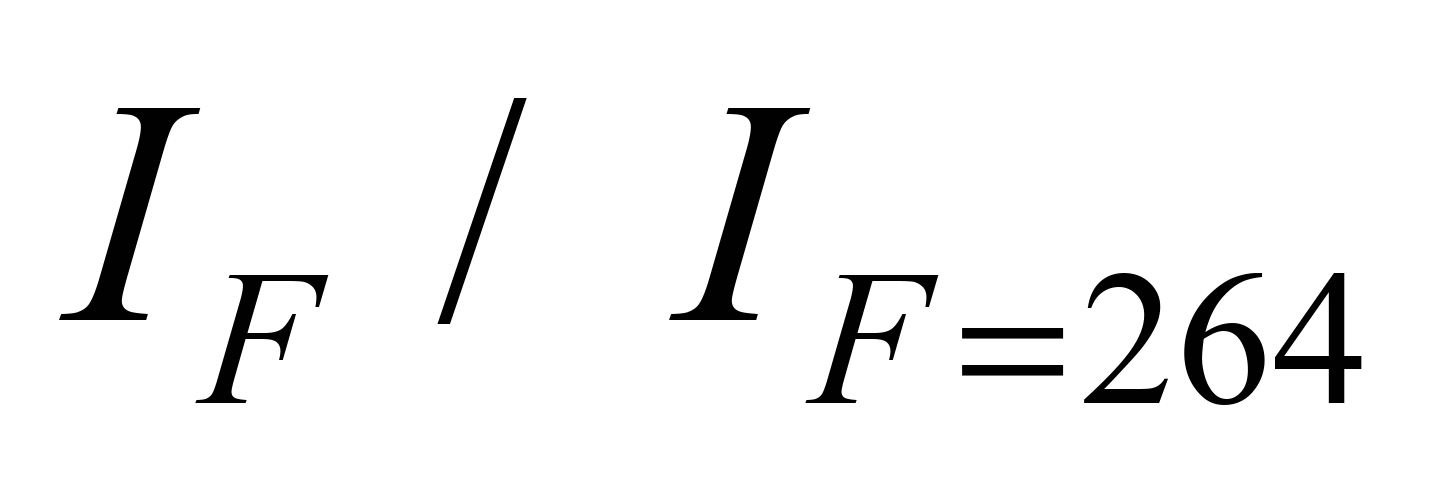
|
|
structures
|
1
|
2.01-2.13
|
5.79-6.02
|
|
pad
|
1
|
1.83-2.06
|
4.47-5.57
|
|
sleeper
|
1
|
1.90-2.00
|
4.94-5.38
|
|
ballast
|
1
|
1.94-2.73
|
5.07-7.7
|
|
ground base
|
1
|
1.98-2.25
|
5.06-6.63
|
Following
the results of data analysis given in Table 4, the railway track,
under the influence of the rolling stock having the load of
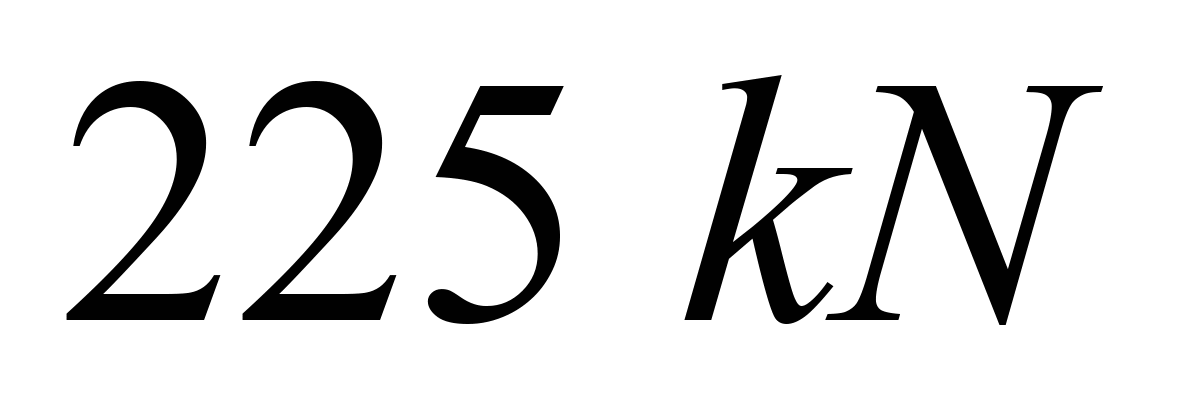 ,
performs less work than the track having the loads of
,
performs less work than the track having the loads of
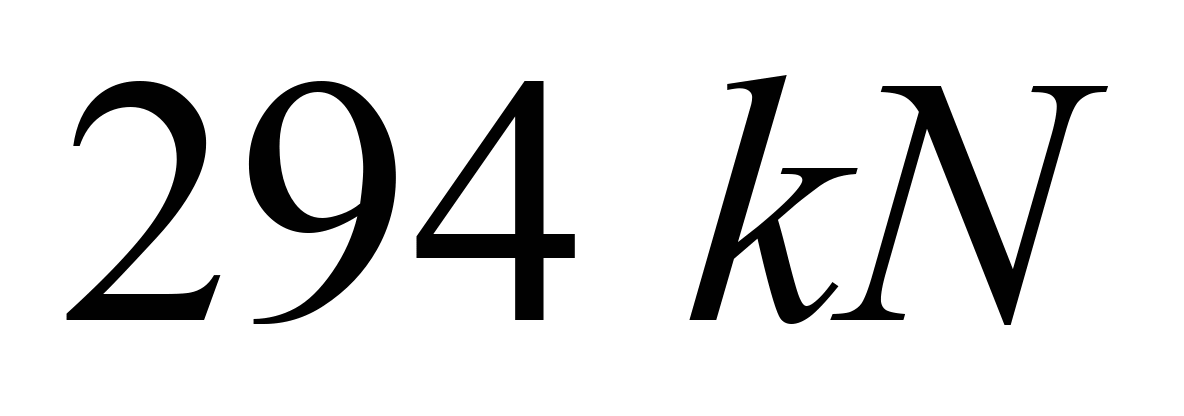 and
and
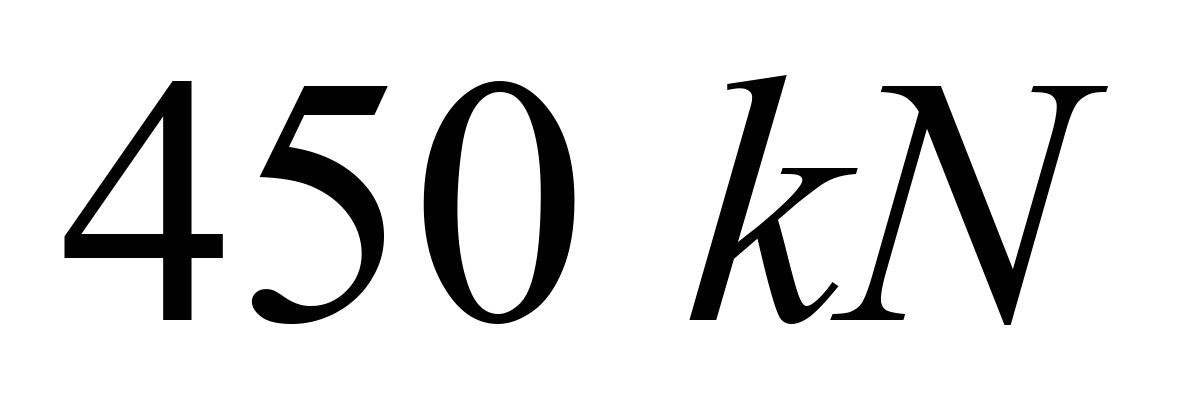 respectively by 2.24 and 6.16 times. The intensity of using the
elements and trackform having the loads of
respectively by 2.24 and 6.16 times. The intensity of using the
elements and trackform having the loads of
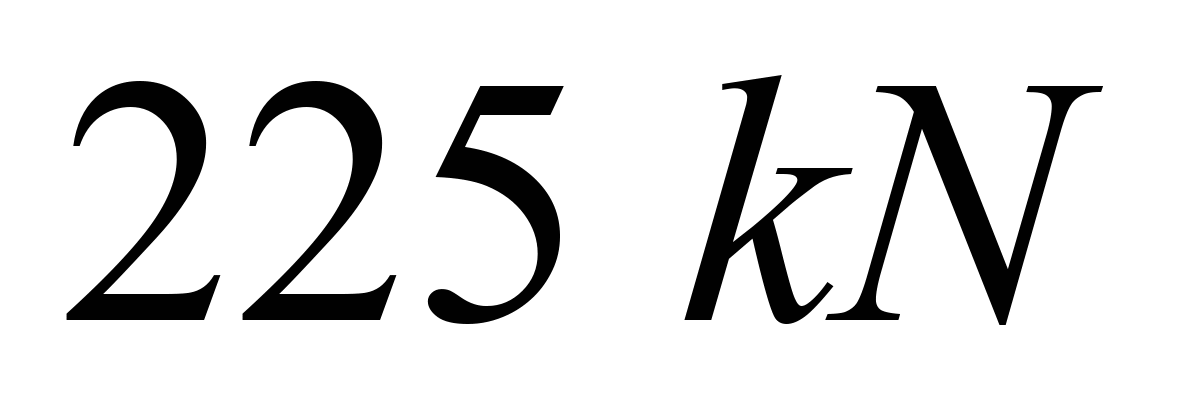 is less than that of the tracks having the load of
is less than that of the tracks having the load of
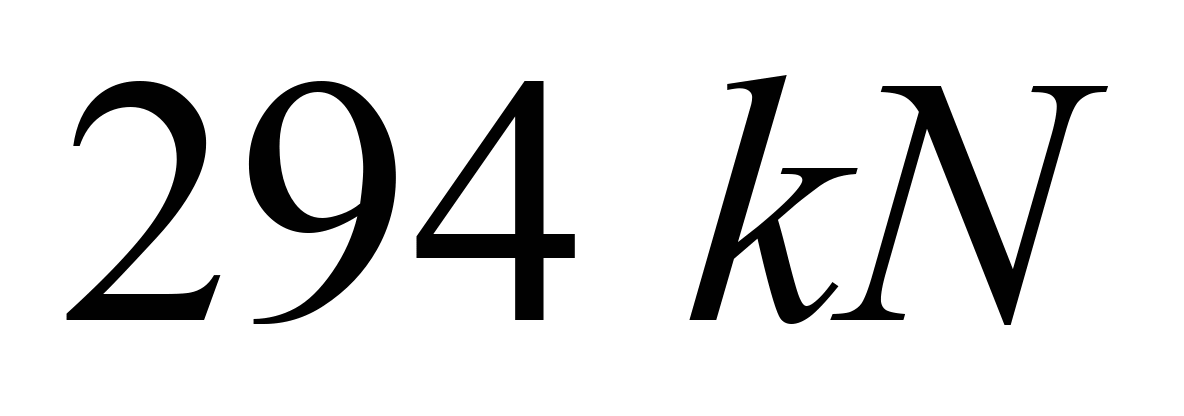 and
and
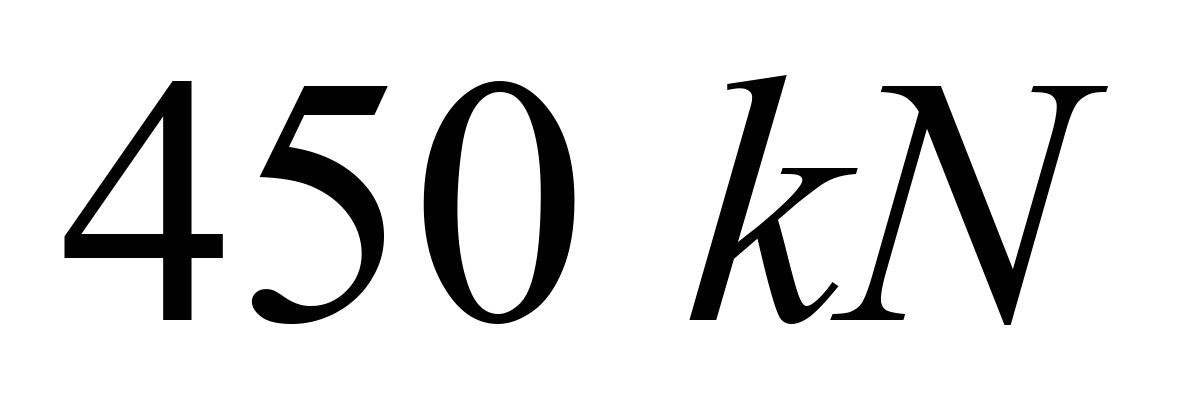 respectively by 2.08 and 5.66 times. It allows establishing the
interdependences of the rolling stock dynamics and the intensity of
using the railway track elements.
respectively by 2.08 and 5.66 times. It allows establishing the
interdependences of the rolling stock dynamics and the intensity of
using the railway track elements.
The use of proposed evaluation
parameters of deformability behavior will further allow us to define
the effect of the aggregate change in the states of elements on the
trackform operation and, in compliance with the current requirements
for the trackform and its maintenance, to form the set of evaluation
data of the track state in terms of acceptable deviations in the
trackform elements.
Originality and
practical value
Trackform and parameters of the
elements of the track superstructure and substructure can be
described using the railway tracks deformability behavior. In the
future this conclusion will help to increase the efficiency of the
dynamic properties of rolling stock when designing and provide
availability of the infrastructure during operation. Changes in the
regulatory framework of rail transport in recent years provide for
the observance of its functional safety, therefore, the question of
the need to assess the impact of rolling stock on the way to be
regarded as a dynamic process which must be taken into account track
deformability resistance. This study provides a basis which takes
into account the stiffness of deformation to allow forming the
regulatory framework for functional safety of railway track in
Ukraine.
Conclusion
The paper presents the
investigation of deformability parameters of the railway track under
various operating conditions as the initial data for forming the
forced oscillations of a rolling stock and the intensity of using
the elements of a trackform. In paper, the theoretical
provisions are proposed, and new analytical dependences of mutual
influence of railway rolling stock and railway track are determined.
It is found that the ratio
between the work intensity value of the elements and the trackform,
as criteria for deformability behavior, may be used as the
evaluation parameters of the functionally safe operation of the
track. The given parameters will serve as the basis for classifying
the critical track states when it is expedient to repair and
maintain the track to ensure the required level of trackform use
efficiency during its service life.
LIST OF REFERENCE LINKS
Бондаренко, І. О.
Моделювання з метою встановлення
оціночних умов функціональної безпеки
залізничної колії / I. O. Бондаренко //
Восточно-Европейский журнал передовых
технологий. – 2016. – Т. 1, № 7 (79). –
С. 4–10. doi:
10.15587/1729-4061.2016.59874
Татарінова, В. А. Теоретичні
дослідження руху одиниці рухомого
складу / В. А. Татарінова, Л. О. Недужа
// Електромагнітна сумісність та безпека
на залізничному транспорті. – 2018. – №
16. –
С. 121–126.
An
analytical
mathematical
method for
calculation of
the dynamic
wheel-rail
impact force
caused by
wheel flat
/ M.
Bogdevicius, R.
Zygiene, G. Bureika, S. Dailydka
// Vehicle
system dynamics. – 2016. – Vol.
54. – Іss.
5. –
Р. 689–705.
doi:
10.1080/00423114.2016.1153114
Bondarenko, I. Development of
algorithm for calculating dynamic processes of railroad track
deformability work / I. Bondarenko // Eastern-European Journal of
Enterprise Technologies. – 2016. – Vol.
6. – Іss.
7 (84). – P.
28–36. doi:
10.15587/1729-4061.2016.85464
Comparison of
simplified and complete contact modeling
approaches in simulations of high
adhesion locomotives
/ M. Spiryagin,
I. Persson, E. A. H. Vollebregt, C. Cole // Dynamics
of Vehicles on Roads and
Tracks : Proceedings of the 25th
International Symposium (14–18
August 2017, Rockhampton, Queensland,
Australia).
–
London, 2017. – Vol. 2. – Р.
613–619.
Derailment risk and
dynamics of railway vehicles in curved
tracks: Analysis
of the effect of failed fasteners
/ S. Morales-Ivorra, J. I. Real, C.
Hernández, L. Montalbán
// Journal of
Modern Transportation.
– 2016. – Vol. 24.
– Іss. 1.
–
P. 38–47.
doi: 10.1007/s40534-015-0093-z
Development of the procedure for
verifying the feasibility of designing an active suspension system
for transport carriages / N. Ershovа,
I. Bondarenko, O. Shibko, N. Velmagina //
Eastern-European Journal of Enterprise Technologies. – 2018. –
Vol. 3. –
Іss. 7 (93). –
P. 53–63.
doi:
10.15587/1729-4061.2018.131534
Dynamic Track Irregularities
Modeling when Studying Rolling Stock Dynamics
/ I. Bondarenko,
R.
Keršys,
O. Lunys,
L. Neduzha //
Proc.
of 23rd Intern.
Sci. Conf.
(October 2–4, 2019)
/ Kaunas University of Technology. – Palanga,
2019.
Facchinetti, A. Rolling Stock
Dynamic Evaluation by Means of Laboratory Tests / A. Facchinetti,
S. Bruni, W. Zhang // Intern. Journal of Railway Technology. –
2013. – Vol. 2. – Iss. 4. – P. 99–123.
doi:
10.4203/ijrt.2.4.6
Heat capacity and heat transfer
coefficient estimation for a dynamic thermal model of rail vehicles
/ R. N.
Hofstädter, T. Zero, C. Dullinger, G. Richter, M. Kozek //
Mathematical and Computer Modelling of Dynamical Systems. – 2017.
– Vol. 23. –
Iss. 5. – P.
439–452.
doi:
10.1080/13873954.2016.1263670
Impact of maintenance conditions of
vehicle components on the vehicle–track
interaction loads
/ N. Kuka, R. Verardi, C. Ariaudo, J.
Pombo // Proceedings
of the Institution of
Mechanical Engineers, Part C: Journal of
Mechanical Engineering Science. – 2018.
– Vol. 232.
– Iss. 15.
–
P. 2626–2641.
doi: 10.1177/0954406217722803
Kalivoda, J. Enhancing
the scientific level of engineering training of railway transport
professionals / J. Kalivoda, L. О.
Neduzha // Наука та прогрес
транспорту.
– 2017. – № 6 (72).
– С. 128–137. doi:
10.15802/stp2017/119050
Kalivoda, J. Simulation of Safety
Against Derailment Tests of an Electric Locomotive / J. Kalivoda,
L. Neduzha // Еngineering Mechanics
2019 : Proc. of 25th Intern. Conf. (Svratka, Czech Republic, 13–16
May 2019). – Svratka, 2019. – P. 177–180. doi:
10.21495/71-0-177
Kraft, S. An
approach for the validation of railway
vehicle models
based on on-track measurements / S.
Kraft, J. Causse, F. Coudert
// Vehicle System Dynamics. –
2015. –
Vol. 232. –
Iss. 10.
–
P. 1480–1499.
doi:
10.1080/00423114.2015.1054406
Kyryl'chuk, O. High
Speed Stability of a Railway Vehicle Equipped with Independently
Rotating Wheels /
O.
Kyryl’chuk, J. Kalivoda, L. Neduzha //
Еngineering Mechanics 2018 : Proc. of 24th
Intern. Conf. (Svratka,
Czech Republic, May 14–17, 2018). – Svratka, 2018.
– P. 473–476. doi: 10.21495/91-8-473
Mathematical
and Computer Models in Estimation of Dynamic Processes of Vehicles
/ A.
Assemkhanuly,
Z.
Niyazova, R.
Ustemirova,
A.
Karpov,
A.
Muratov,
K.
Kaspakbayev
// Journal
of Theoretical and Applied Information Technology. – 2019. –
Vol. 97, No 10. – P. 2803–2820.
Modelling, simulation and
applications of
longitudinal train dynamics / C.
Cole, M. Spiryagin, Q. Wu, Y. Q. Sun
// Vehicle
System Dynamics.
– 2017. – Vol.
55. – Iss. 10.
– P. 1498–1571. doi:
10.1080/00423114.2017.1330484
Research of Lateral Vibrations of a
Passenger Wagon Running Along the Curved Path
/ R. Skvireckas,
A. Keršys,
R. Keršys,
V. Lukoševičius
// Journal of
Vibroengineering. – 2012. – Vol.
14. – Iss. 2. – P. 706–714.
Sichani, M. S.
A fast wheel–rail contact model for
application to
damage analysis in vehicle dynamics
simulation / M.
S. Sichani, R. Enblom, M. Berg // Wear. –
2016. –
Vol. 366-367. –
P. 123–130.
doi: 10.1016/j.wear.2016.06.015
Simplified and advanced modelling
of traction control systems of heavy-haul
locomotives /
M. Spiryagin,
P. Wolfs, F. Szanto, C. Cole // Vehicle System Dynamics. –
2015. – Vol.
53. – Iss. 5. – P. 672–691.
doi: 10.1080/00423114.2015.1008016
Tatarinova, V. A. Research
of Locomotive Mechanics Behavior
/ V. A. Tatarinova, J. Kalivoda, L. О. Neduzha // Наука
та прогрес транспорту.
– 2018.
– № 5 (77).
– С. 104–114. doi:
10.15802/stp2018/148026
Theoretical
foundations for conceptually new rolling stock modules : monograph
/ M. I. Gorbunov, O. V. Fomin, V. V. Kovalenko, R. Y. Domin. –
Sievierodonetsk
: Volodymyr
Dahl East Ukrainian National University, 2018. – 100 p.
Wu, Q.
Longitudinal
train dynamics:
an overview
/ Q. Wu,
M. Spiryagin,
C. Cole
// Vehicle
System
Dynamics. –
2016. –
Vol.
54. – Iss.
12. – P.
1688–1714.
doi: 10.1080/00423114.2016.1228988
І. О. Бондаренко1*,
Л. О. НЕДУЖА2*
1*Каф.
«Колія та колійне господарство»,
Дніпровський національний університет
залізничного транспорту імені академіка
В. Лазаряна, вул. Лазаряна, 2, Дніпро,
Україна, 49010, тел.
+38 (063) 802 21 80,
ел. пошта irina_bondarenko@ua.fm,
ORCID 0000-0003-4717-3032
2*Каф. «Теоретична
та будівельна механіка», Дніпровський
національний
університет залізничного
транспорту імені академіка В. Лазаряна,
вул. Лазаряна, 2, Дніпро, Україна, 49010,
тел. +38 (067) 810 51 65,
ел. пошта nlorhen@i.ua,
ORCID 0000-0002-7038-3006
ДОСЛІДЖЕННЯ ВПЛИВУ ДИНАМІКИ
РУХОМОГО
СКЛАДУ НА ІНТЕНСИВНІСТЬ
ВИКОРИСТАННЯ
ЕЛЕМЕНТІВ
КОНСТРУКЦІЇ КОЛІЇ
Мета.
Основною метою цієї роботи є дослідження
параметрів деформованості залізничної
колії за різних експлуатаційних умов
як вихідних даних для формування
вимушених коливань рухомого складу й
інтенсивності використання елементів
конструкції колії. Методика.
Для дослідження було розроблено
оригінальну модель конструкції колії,
описану з використанням основних понять
теорій пружності й поширення пружних
хвиль. Результати.
Установлено, що
співвідношення між інтенсивністю
роботи елементів і конструкцією колії
як критерієм деформованості можна
використати як оціночний параметр
функціонально безпечної експлуатації
колії. Це може бути основою для
класифікації критичних станів конструкцій
колії за яких необхідно проводити
роботи з відновлення її працездатності,
щоб забезпечити необхідний рівень
ефективності використання конструкції
протягом терміну служби.
Наукова новизна.
Конструкція колії й параметри елементів
ії верхньої та нижньої будов можуть
бути описані з використанням поведінки
деформованості. Надалі цей висновок
допоможе підвищити ефективність
динамічних властивостей рухомого
складу під час проектування й забезпечити
готовність інфраструктури під час
експлуатації. Практична
значимість. Зміни в
нормативно-правовій базі залізничного
транспорту за останні роки передбачають
дотримання його функціональної безпеки.
Тому питання про необхідність оцінки
впливу рухомого складу на колію слід
розглядати як динамічний процес, який
повинен брати до уваги опір деформованості
колії. Це дослідження становить основу
для обліку жорсткості деформації, що
дозволяє сформувати нормативну базу
для гарантування функціональної безпеки
залізничної колії в Україні.
Ключові слова: конструкція
колії; параметри деформованості;
коливання; деформованість залізничної
колії
И. А. Бондаренко1*,
Л. А. НЕДУЖАЯ2*
1*Каф.
«Путь и путевое хозяйство», Днипровский
национальный университет
железнодорожного
транспорта имени академика В. Лазаряна,
ул. Лазаряна, 2, Днипро, Украина, 49010, тел.
+38 (063) 802 21 80,
эл.
почта irina_bondarenko@ua.fm, ORCID
0000-0003-4717-3032
2*Каф.
«Теоретическая и строительная механика»,
Днипровский национальный
университет железнодорожного транспорта
имени академика В. Лазаряна,
ул. Лазаряна,
2, Днипро, Украина, 49010, тел. +38 (067) 810 51 65,
эл. почта nlorhen@i.ua,
ORCID 0000-0002-7038-3006
ИССЛЕДОВАНИЕ ВЛИЯНИЯ ДИНАМИКИ
подвижного состава на
интенсивность
использования
ЭЛЕМЕНТОВ КОНСТРУКЦИИ
ПУТИ
Цель.
Основной целью данной работы является
исследование параметров деформированности
железнодорожного пути при различных
эксплуатационных условиях в качестве
исходных данных для формирования
вынужденных колебаний подвижного
состава и интенсивности использования
элементов конструкции пути. Методика.
Для исследования была разработана
оригинальная модель конструкции пути,
описанная с использованием основных
положений теорий упругости и
распространения упругих волн. Результаты.
Установлено, что соотношения между
интенсивностью работы элементов и
конструкцией пути как критерием
деформированности можно использовать
в качестве оценочного параметра
функционально безопасной эксплуатации
пути. Это может быть основой для
классификации критических состояний
конструкций пути, при которых необходимо
проводить работы по восстановлению
его работоспособности, чтобы обеспечить
необходимый уровень эффективности
использования конструкции в течение
срока службы. Научная
новизна. Конструкция
пути и параметры элементов его верхнего
и нижнего строений могут быть описаны
с использованием поведения
деформированности. В дальнейшем этот
вывод поможет повысить эффективность
динамических свойств подвижного состава
при проектировании и обеспечить
готовность инфраструктуры во время
эксплуатации. Практическая
значимость. Изменения
в нормативно-правовой базе железнодорожного
транспорта в последние годы предусматривают
соблюдение его функциональной
безопасности. Поэтому вопрос о
необходимости оценки влияния подвижного
состава на путь следует рассматривать
как динамический процесс, который
должен принимать во внимание сопротивление
деформированности пути. Это исследование
обеспечивает основу для учета жесткости
деформации, что позволяет сформировать
нормативную базу для обеспечения
функциональной безопасности
железнодорожного пути в Украине.
Ключевые слова: конструкция
пути; параметры деформированности;
колебания; деформированность
железнодорожного пути
REFERENCES
Bondarenko, I. O. (2016). Modeling
for establishment of evaluation conditions of functional safety of
the railway track. Eastern-European
Journal of Enterprise Technologies, 1/7(79),
4-10.
doi: 10.15587/1729-4061.2016.59874
(in Ukrainian)
Tatarinova, V., & Neduzha,
L. (2018). Theoretical Research of the Traction Vehicle Motion.
Electromagnetic compatibility and
safety on railway transport, 16,
121-126. (in
Ukrainian)
Bogdevicius, M., Zygiene, R.,
Bureika, G., & Dailydka, S. (2016). An analytical mathematical
method for calculation of the dynamic wheel–rail
impact force caused by wheel flat. Vehicle
System Dynamics, 54(5), 689-705.
doi:
10.1080/00423114.2016.1153114
(in English)
Bondarenko, I. (2016). Development
of algorithm for calculating dynamic processes of railroad track
deformability work. Eastern-European
Journal of Enterprise Technologies, 6/7(84),
28-36. doi:
10.15587/1729-4061.2016.85464
(in English)
Spiryagin,
M., Persson, I., Vollebregt, E. A. H.,
&
Cole, C. (2017). Comparison of
simplified
and complete contact modelling
approaches
in simulations of high adhesion
locomotives.
Dynamics
of Vehicles on Roads
and
Tracks.
Vol.
2:
Proceedings
of the 25th
International
Symposium, (pp.
613-619).
London.
(in English)
Morales-Ivorra, S., Real, J. I.,
Hernández, C., & Montalbán, L. (2016). Derailment risk and
dynamics of railway vehicles in curved tracks: Analysis of the
effect of failed fasteners. Journal
of Modern Transportation, 24(1),
38-47.
doi: 10.1007/s40534-015-0093-z
(in English)
Ershovа,
N., Bondarenko, I., Shibko, O., & Velmagina, N. (2018).
Development of the procedure for verifying the feasibility of
designing an active suspension system for transport carriages.
Eastern-European Journal of Enterprise Technologies, 3/7(93),
53-63. doi:
10.15587/1729-4061.2018.131534
(in English)
Bondarenko, I.,
Keršys, R.,
Lunys,
O., &
Neduzha, L. (2019).
Dynamic Track Irregularities Modeling when Studying Rolling Stock
Dynamics. Proceedings
of 23rd International Scientific Conference (October
2–4, 2019).
Palanga.
(in English)
Facchinetti,
A., Bruni, S., & Zhang, W. (2013). Rolling Stock Dynamic
Evaluation by Means of Laboratory Tests. International
Journal of Railway Technology, 2(4),
99-123.
doi:
10.4203/ijrt.2.4.6
(in English)
Hofstädter,
R. N., Zero, T., Dullinger, C., Richter, G., & Kozek, M.
(2016). Heat capacity and heat transfer coefficient estimation for
a dynamic thermal model of rail vehicles. Mathematical
and Computer Modeling of Dynamical Systems, 23(5),
439-452.
doi:
10.1080/13873954.2016.1263670
(in
English)
Kuka, N., Verardi, R., Ariaudo, C.,
& Pombo, J. (2018).
Impact of maintenance conditions of vehicle components on the
vehicle–track
interaction loads. Proceedings of the
Institution of Mechanical Engineers, Part C: Journal of Mechanical
Engineering Science, 232(15),
2626-2641.
doi:
10.1177/0954406217722803
(in English)
Kalivoda, J., & Neduzha, L. O.
(2017). Enhancing the Scientific Level of Engineering Training of
Railway Transport Professionals. Science
and Transport Progress, 6(72),
128-137. doi: 10.15802/stp2017/119050
(in English)
Kalivoda, J., & Neduzha, L. O.
(2019). Simulation
of Safety Against Derailment Tests of an Electric Locomotive,
Еngineering Mechanics
2019: Proc. of 25th Intern. Conf., 177-180.
Svratka. doi:
10.21495/71-0-177 (in English)
Kraft, S., Causse, J., &
Coudert, F. (2015). An approach for the validation of railway
vehicle models based on on-track measurements. Vehicle
System Dynamics, 53(10), 1480-1499.
doi:
10.1080/00423114.2015.1054406
(in English)
Kyryl’chuk, О., Kalivoda, J., &
Neduzha, L. (2018). High
speed stability of a railway vehicle equipped with independently
rotating wheels, Engineering Mechanics 2018:
Proc. of 24th
Intern Conf.
Svratka.
doi:
10.21495/91-8-473 (in
English)
Assemkhanuly,
А., Niyazova,
Z., Ustemirova,
R.,
Karpov,
A.,
Muratov,
А., &
Kaspakbayev,
К.
(2019). Mathematical
and Computer Models in Estimation of Dynamic Processes of Vehicles.
Journal of Theoretical and Applied
Information Technology, 97(10),
2803-2820 (in English)
Cole, C.,
Spiryagin, M., Wu, Q., & Sun, Y. Q.
(2017).
Modelling,
simulation and applications
of
longitudinal train dynamics.
Vehicle
System
Dynamics,
55(10),
1498-1571. doi:
10.1080/00423114.2017.1330484 (in
English)
Skvireckas,
R.,
Keršys,
A.,
Keršys,
R.,
& Lukoševičius,
V.
(2012). Research
of Lateral Vibrations of a Passenger Wagon Running Along the Curved
Path.
Journal of Vibroengineering.
14(2), 706-714.
(in English)
Sichani, M. S., Enblom, R., &
Berg, M. (2016).
A fast wheel–rail contact model for
application to
damage analysis in vehicle dynamics
simulation.
Wear, 366-367,
123-130. doi: 10.1016/j.wear.2016.06.015
(in English)
Spiryagin,
M., Wolfs, P., Szanto, F., & Cole,
C. (2015).
Simplified
and advanced modelling
of traction
control systems of heavy-haul
locomotives.
Vehicle
System Dynamics,
53(5),
672-691. doi: 10.1080/00423114.2015.1008016
(in English)
Tatarinova, V. A.,
Kalivoda, J., &
Neduzha, L. O.
(2018). Research
of Locomotive Mechanics Behavior.
Science and Transport Progress,
5(77),
104-114. doi: 10.15802/stp2018/148026
(in English)
Gorbunov,
M. I, Fomin, O. V, Kovalenko, V. V., &
Domin, R. Y. (2018). Theoretical
foundations for conceptually new rolling stock modules: monograph.
Sievierodonetsk:
Volodymyr
Dahl East Ukrainian National University.
(in English)
Wu, Q.,
Spiryagin, M., & Cole, C. (2016). Longitudinal train dynamics:
an overview. Vehicle
System Dynamics,
54(12),
1688-1714. doi: 10.1080/00423114.2016.1228988
(in English)
Received:
March 11, 2019
Accepted:
July 08,
2019
axis – direction of force action. An explanation to the directions
and angles is given in Fig. 1.
.
The main dependences of motion-direction are shown in [1, 4]. As a
result, the shift of structural particles in time has been obtained
(see Fig. 2-5).
.
The experiment was carried out on a single-track section. The
trackform elements: UIC60 rail track, reinforced concrete sleepers
with KPP-5 type fastening,
ballast depth, the roadbed is a sandy loam subgrade. The modulus of
the track elasticity in the vertical plane for calculation by the
existing method was taken from the experimental data and made
.
Table 1 shows the data calculated by the existing method «Track
strength calculation»,
the proposed method and the experiment obtained ones.
:
(1)
– force that affects the element;
– shift caused by the force during the action [
,
].
as the sum of works performed by all the track elements in one
section under the influence of the rolling stock with respect to the
time of perception and reaction of the elements:
(2)
– a number
of elements getting a force impact.
thick, subgrade. They are given in Table 2.