Introduction

Unplanned ammonia release as a result of accident or terror act can cause harm for persons and intensive environment pollution [4, 6, 10, 12]. From the point view of industrial safety, in case of possible unplanned ammonia release we must solve two important problems. The first problem is prediction of possible contamination zones (their dimensions, intensity and development in time). To solve the problem of air contamination in case of unplanned toxic chemicals release Gaussian plume model or some analytical models are widely used [2, 3, 10–13, 15]. These models allow quickly calculating zones of chemical contamination. These models are implemented in some codes, for example, ALOHA, CULLPUFF, etc.

The second problem of unplanned ammonia release is protection environment from pollution and reducing negative influence of toxic chemical after NH₃ emission. In this work we study the problem of neutralizer supply in to ammonia plume to reduce the intensity and dimensions of contamination zones.

Problem statement

We consider the unplanned ammonia release at ammonia pump station which is situated at ammonia pipeline Toliatti-Odessa. To reduce the negative effect on environment in this case we propose to supply neutralizer (NL) to the plume of toxic chemical. As the neutralizer the acid solution can be used. If, for example, we use H₂SO₄ solution, the process of chemical interaction «ammonia + neutralizer» can be described as follows

(1)

Application of neutralizer will cause NH₃ reduction in atmosphere. To supply NL into the plume of toxic chemical the special equipment will be used (Fig. 1).

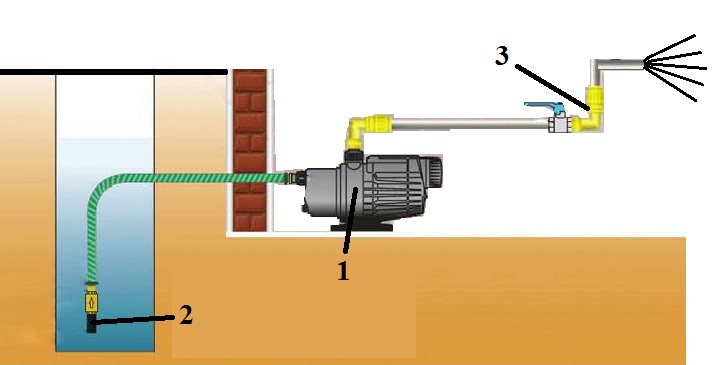
Fig. 1. Equipment for neutralizer supply:
1 – pump; 2 – vessel containing neutralizer;
3 – rotatable nozzle

But in case of NL supply we face some problems: what amount of neutralizer must be supplied, what will be the effect of the neutralization for different meteorological conditions, etc. To answer these questions and develop the effective strategy of neutralization we can’t use physical experiment but only the mathematical one.

Purpose

The purpose of this paper is to develop a mathematical model for quick computing the process of chemical interaction «ammonia + neutralizer» in case of unplanned ammonia release at ammonia pump station.

Mathematical formulation

To simulate the ammonia and acid dispersion in atmosphere 2D transport model is used [5, 7]

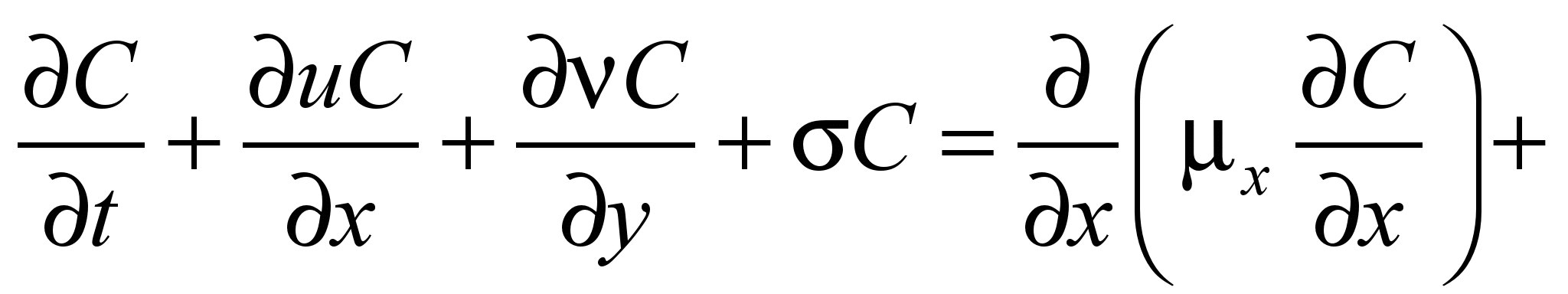
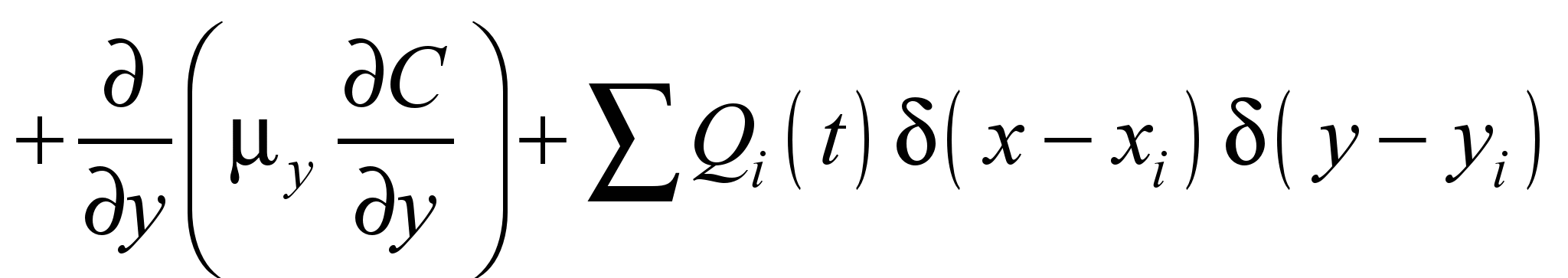, (2)

where С is mean concentration of admixture (ammonia and H₂SO₄); u, v are the wind velocity components;  is the parameter taking into account the process of pollutant washout and gravity fallout [5];

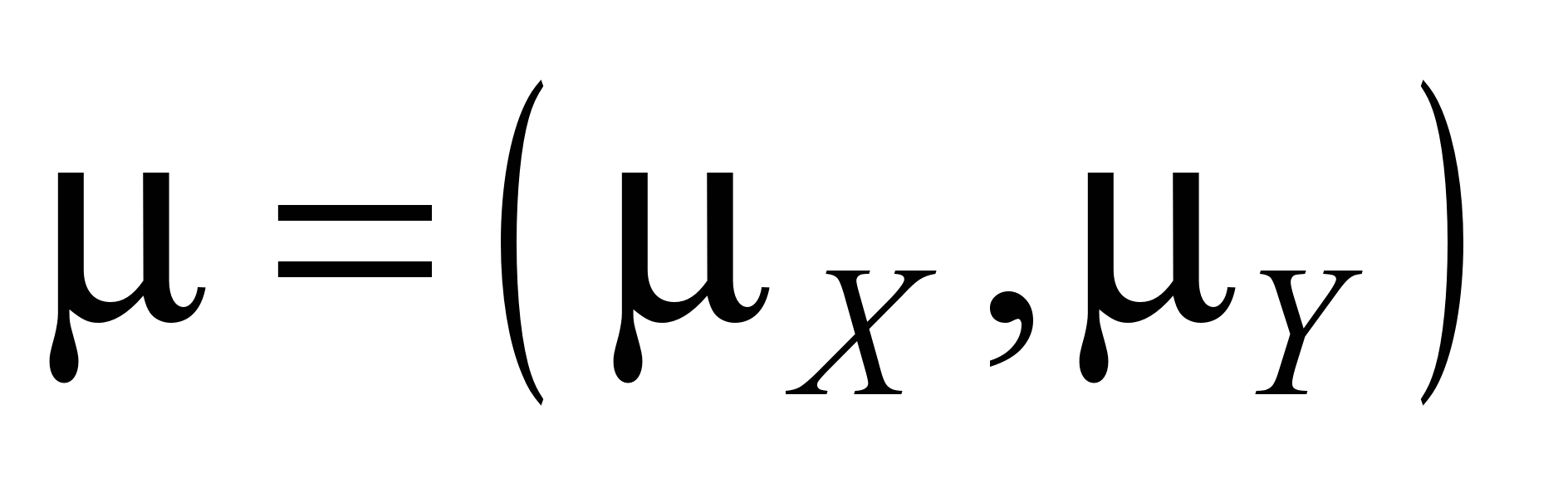are the diffusion coefficients; is intensity of point source emission; 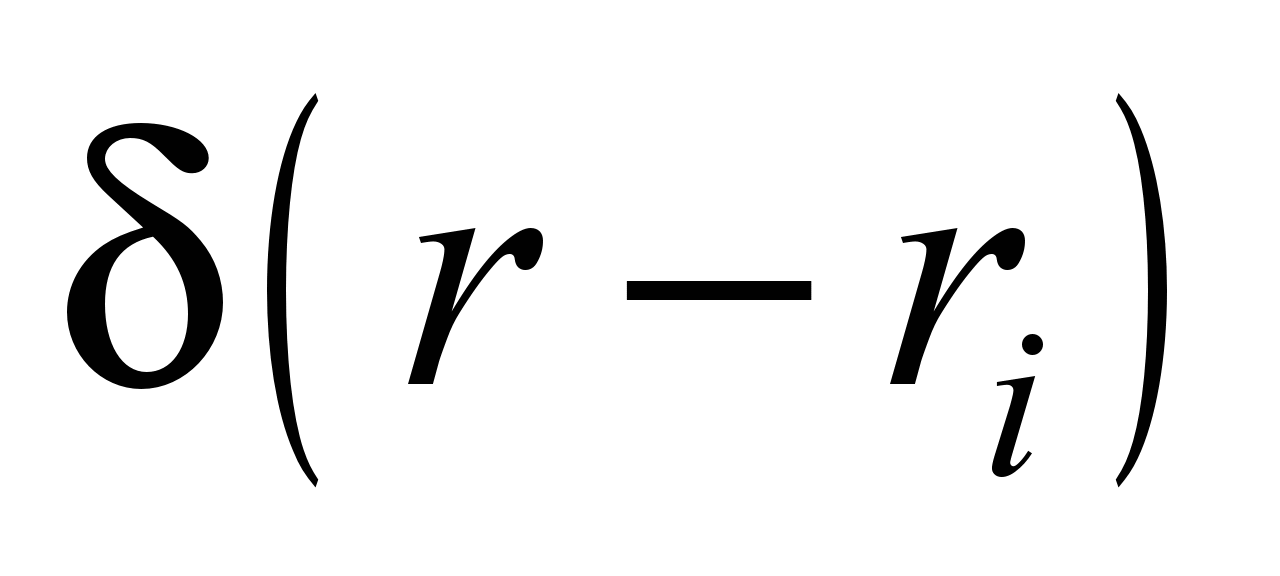are Dirac delta function; 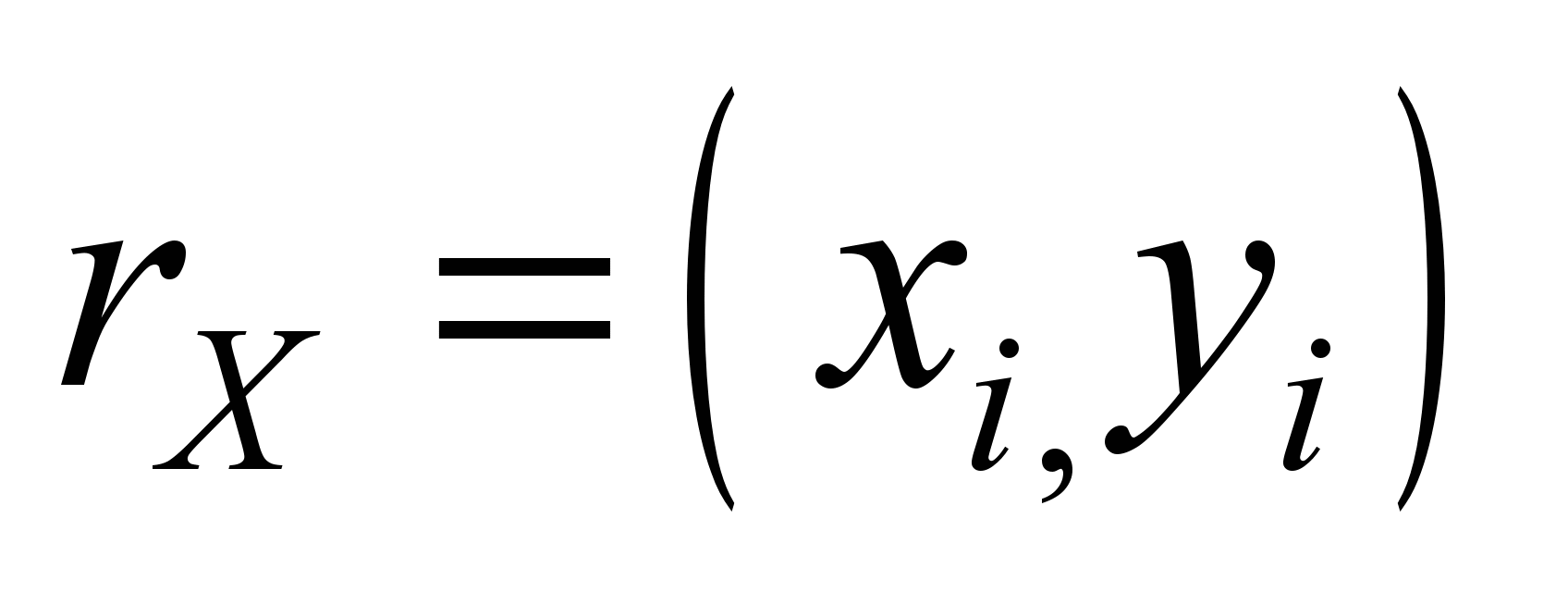are the
coordinates of the point source.

We consider ammonia and NL dispersion among buildings it means that we must take into account influence of these obstacles on process of NH₃ and NL dispersion. To simulate the flow pattern in the case of the buildings at the territory of Pump Station the 2D model of potential flow is used [7]

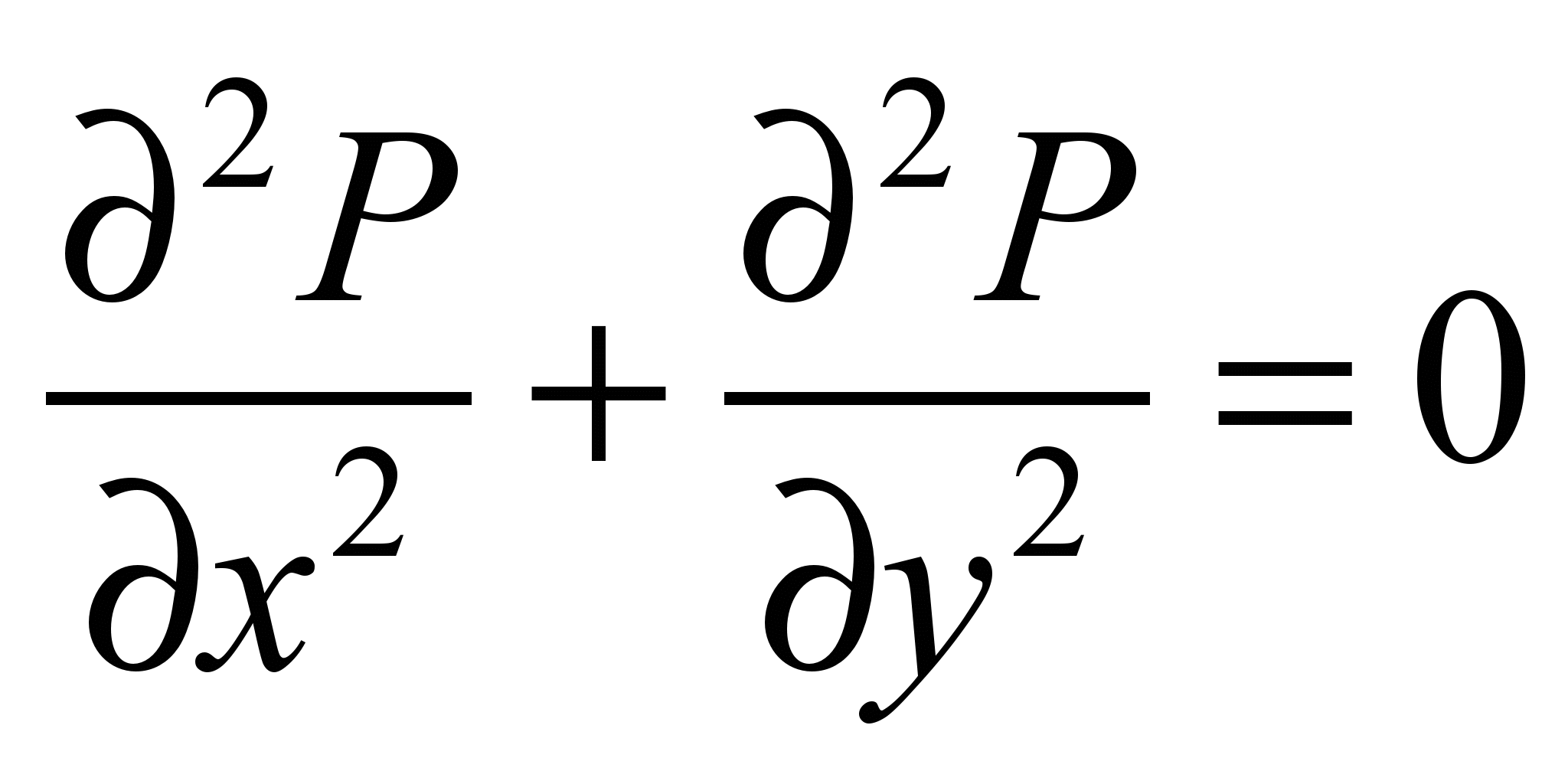, (3)

where 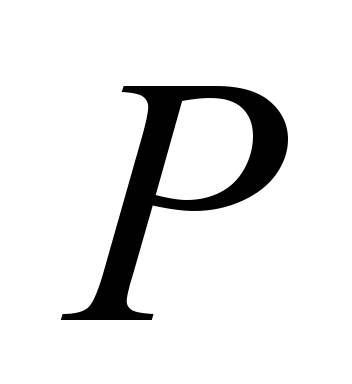is the potential of velocity.

The wind velocity components are calculated as follows:

.

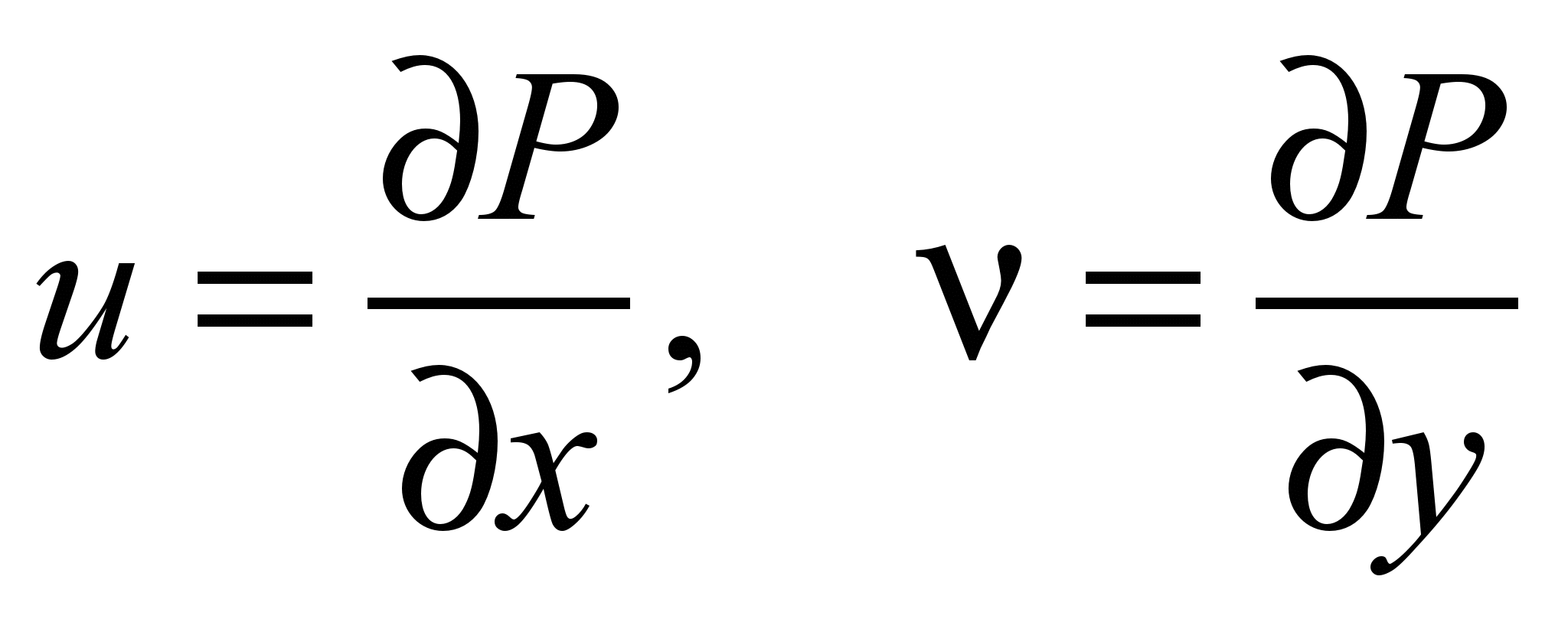Boundary conditions for modeling equations are discussed in [5, 7].

Numerical model

The computation of wind pattern and pollutant dispersion is carried out on rectangular grid. To create the form of buildings we use porosity technique or so called «markers method» [1, 7]. Markers are used to separate the computational cells where flow takes place from the cells which correspond to buildings. Description of the finite difference schemes, used by us, is discussed in [1, 7]. To solve equation (2) we used change–triangle difference scheme, while A. A. Samarskii’s and implicit difference scheme was used for
numerical integration of Eq. (3).

For coding of difference formulae, we used FORTRAN language.

Findings

Computational region is shown in Fig. 2 In this figure one can see position of unplanned ammonia release and position of NL supply equipment. It was supposed that ammonia release takes place at time t=0 and at time t=15 sec neutralizer supply starts.

Fig. 2. Sketch of computational region
(ammonia pump station):
1, 2, 3, 4 – buildings; 5 – position of neutralizer supply;
6 – position of ammonia release at the territory
of pump station

Contaminated zone in case of neutralizer supply absence is shown in Fig. 3. We can see that contaminated zone covers all the territory of ammonia pump station.

Fig. 3. Computed ammonia concentration, t=35 s
(no neutralizer supply)

In Fig. 4 the contaminated zone in the case of neutralizer supply is shown.

As we can see from Fig. 4 neutralizer supply allows quickly reducing dimensions of contamination zone in atmosphere.

Worthy of note that computational time was 6 sec. It allows using the model for development of protection measures at chemically dangerous enterprises.

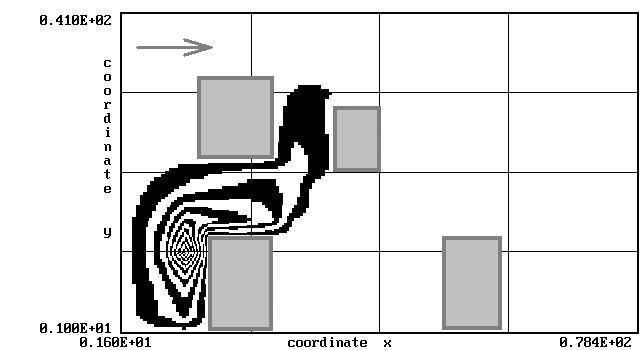
Fig. 4. Computed ammonia concentration, t=28 s
(neutralizer supply)

Originality and practical value

Mathematical model has been developed to compute dispersion and chemical interaction between ammonia and neutralizer which is used to reduce ammonia concentration in atmosphere. The presented mathematical model is based on the application of the fundamental equations of aerodynamics and mass transfer.

The peculiarity of the developed model is the use of standard meteorological information and quick calculation of modeling process in comprehensive computational region.

Conclusions

Numerical model for estimating the efficiency of neutralizer supply to ammonia plume is proposed. Proposed numerical model allows to compute ammonia concentrations and neutralizer concentrations and chemical interaction between substances. The solution of the aerodynamic problem is based on the numerical integration of the equation for the velocity potential. The equation of mass transfer is used to calculate dispersion of ammonia and neutralizer dispersion in atmosphere. The mass transfer equation takes into account the convective and diffusive transport of admixture in atmosphere with account of buildings situated near the source of emission. Emission of ammonia and neutralizer is simulated by a point source, which is modeled using the Dirac delta function.

Further improvement of the model should be carried out in the direction of creating a 3D numerical model that takes into account the formation of vortices in the air flow.

LIST OF REFERENCE LINKS

1. Беляев, Н. Н. Моделирование нестационарных процессов аварийного загрязнения атмосферы: монография / Н. Н. Беляев, А. В. Берлов, П. Б. Машихина. – Днепропетровск : Акцент ПП, 2014. – 127 с.

2. Берлянд, М. Е. Прогноз и регулирование загрязнения атмосферы / М. Е. Берлянд. – Ленинград : Гидрометеоиздат, 1985. – 273 с.

3. Бруяцкий, Е. В. Теория атмосферной диффузии радиоактивных выбросов / Е. В. Бруяцкий. – Киев : Ин-т гидромеханики НАН Украины, 2000. – 443 с.

4. Заказнов, В. Ф. Распространение аммиака при разгерметизации аммиакопровода, емкостей / В. Ф. Заказнов, Л. А. Куршева // Исследования и разработки по созданию магистральных аммиакопроводов и складов жидкого аммиака : тр. ГИАП. – Москва, 1985. – С. 57.

5. Марчук, Г. И. Математическое моделирование в проблеме окружающей среды / Г. И. Марчук.– Москва : Наука, 1982. – 320 с.

6. Цыкало, А. Л. Испарение и рассеивание аммиака при его разливах и утечках. Серия: Азотная промышленность / А. Л. Цыкало, И. И. Стрижевский, А. Д. Баглет. – Москва : НИИТЭХИМ, 1982. – 48 с.

7. Численное моделирование распространения загрязнения в окружающей среде / М. З. Згуровский,
   В. В. Скопецкий, В. К. Хрущ, Н. Н. Беляев. – Київ : Наук. думка, 1997. – 368 с.

8. Biliaiev, M. M. Numeric Simulation of Air Pollution in Case of Unplanned Ammonia Release / M. M. Biliaiev, L. V. Amelina // Science and Transport Progress. – 2017. – Vol. 3 (96). – P. 7–14.
   doi: 10.15802/stp2017/104142.

9. Biliaiev, M. M. Numerical simulation of the atmosphere pollution after accident at the “Tolliaty-Odessa” ammonia pipe / M. M. Biliaiev, L. V. Amelina, M. M. Kharytonov // NATO Science for Peace and Security. Series C: Environmental security. – 2013. – P. 391–395. doi: 10.1007/978-94-007-5577-2_66.

10. Daly, A. Accident reconstruction and plume modeling of an unplanned ammonia release / A. Daly, P. Zanetti, M. Jennings // Air Pollution XX. WIT Transactions on Ecology and The Environment. – 2013. – Vol. 174. – P. 3–13. doi: 10.2495/AIR130011.

11. Dispersion Modeling of Hydrogen Sulfide at Cimarex Rands Butte Project Using ALOHA / Bureau of Land Management Pinedale Field Office, SWCA Environmental Consultants. – Wyoming, 2010. – 26 p.

12. Janos, T. Atmostheric spreading model for ammonia released from the poultry house [Electronic resource] / T. Janos. E. Gorliczay, J. Borbely // Ecotoxicologie, Zootehnie si Tehnologii de Industrie Alimentara. – 2016. – Vol. XV/B. – P. 331–337. – Available at: http://protmed.uoradea.ro/facultate/publicatii/ecotox_zooteh_ind_alim/2016B/ipa/17%20Tamas_Janos.pdf – Title from the screen. – Accessed : 30.05.2017.

13. Mellsen, S. B. A Fortran program for calculating chemical hazards using the NATO stanag 2103/ATP-45 algorithm [Electronic resource] / S. B. Mellsen // Suffield memorandum No. 1275. – Alberta : Defence Research Establishment Suffield, 1989. – 34 p. – Available at: http://oai.dtic.mil/oai/oai?verb=getRecord&metadataPrefix=html&identifier=ADA214763. – Title from the screen. – Accessed : 30.05.2017.

14. The analysis of the use of mathematical modeling for emergency planning purposes [Electronic resource] / O. Zavila, P. Dobeš, J. Dlabka, J. Bitta // Bezpecnostni vyzkum. The science for population protection. – 2015. – No. 2. – P. 1–9. – Available at: http://www.population-protection.eu/prilohy/casopis/30/213.pdf. – Title from the screen. – Accessed : 30.05.2017.

15. The Pentagon Shield field program: Toward critical infrastructure protection / T. Warner, P. Benda, S. Swerdlin [et al.] // Bulletin of the American Meteorological Society. – 2007. – Vol. 88. – Iss. 2. – P. 167–176. doi: 10.1175/BAMS-88-2-167.

Л. В. АМЕЛІНА¹, М. М. БІЛЯЄВ², П. Б. МАШИХІНА³

^1*Каф. «Гідравліка та водопостачання», Дніпропетровський національний університет залізничного
транспорту імені академіка В. Лазаряна, вул. Лазаряна, 2, Дніпро, Україна, 49010, тел. +38 (056) 273 15 09,
ел. пошта water.supply.treatment@gmail.com, ORCID 0000-0002-8525-7096
^2*Каф. «Гідравліка та водопостачання», Дніпропетровський національний університет залізничного
транспорту імені академіка В. Лазаряна, вул. Лазаряна, 2, Дніпро, Україна, 49010, тел. +38 (056) 273 15 09,
ел. пошта water.supply.treatment@gmail.com, ORCID 0000-0002-1531-7882
^3*Каф. «Гідравліка та водопостачання», Дніпропетровський національний університет залізничного
транспорту імені академіка В. Лазаряна, вул. Лазаряна, 2, Дніпро, Україна, 49010, тел. +38 (056) 273 15 09,
ел. пошта water.supply.treatment@gmail.com, ORCID 0000-0003-3057-9204

ЗНИЖЕННЯ КОНЦЕНТРАЦІЇ АМІАКУ В АТМОСФЕРІ

ПРИ ЙОГО РАПТОВІЙ ЕМІСІЇ

Мета. Метою даної роботи є побудова чисельної моделі, що дозволяє розраховувати ефективність застосування нейтралізатора для зниження концентрацій аміаку в атмосфері в разі його раптового викиду на території аміачної насосної станції. Властивостями розробленої моделі повинні бути можливість швидкого розрахунку, врахування метеорологічних параметрів та будівель, що розташовані поблизу джерела викиду аміаку та нейтралізатора. Методика. Розроблена модель базується на рівнянні для потенційного потоку та рівнянні дисперсії домішки в атмосфері. Для моделювання хімічної взаємодії аміаку з нейтралізатором використовується рівняння стехіометрії. Рівняння потенційного потоку застосовується для розрахунку швидкості вітру між будівлями. Для вирішення рівняння потенційної течії використовується неявна різницева схема Самарського, а для чисельного рішення рівняння масопереносу – поперемінно-трикутна різницева схема. Чисельне інтегрування здійснюється з використанням прямокутної різницевої сітки. Метод маркування («метод маркерів») вживається для створення вигляду розрахункової області. Емісія аміаку моделюється з використанням дельта функції Дірака для точкового джерела. Результати. Розроблена чисельна модель відноситься до класу «діагностичні моделі». Ця модель враховує основні фізичні фактори, що впливають на процес розсіювання аміаку та нейтралізатора, а також вплив будівель на дисперсію домішки. На основі розроблених чисельних моделей було проведено обчислювальний експеримент із оцінки ефективності застосування нейтралізатора для зниження концентрацій аміаку в атмосфері в разі його раптового викиду на аміачні насосні станції. Наукова новизна. Розроблена чисельна модель дозволяє розрахувати структуру потоку серед будівель та оцінити ефективність подачі нейтралізатора для зменшення забруднення повітря у разі незапланованого вивільнення аміаку. Практична значимість. Модель дозволяє швидко розраховувати ефективність подачі нейтралізатора для зниження негативного впливу емісії аміаку на людей та навколишнє середовище.

Ключові слова: забруднення атмосфери; викид аміаку; нейтралізація; чисельне моделювання

Л. В. АМЕЛИНА¹, Н. Н. беляев², П. Б. МАШИХИНА³

^1*Каф. «Гидравлика и водоснабжение», Днепропетровский национальный университет железнодорожного
транспорта имени академика В. Лазаряна, ул. Лазаряна, 2, Днипро, Украина, 49010, тел. +38 (056) 273 15 09,
эл. почта water.supply.treatment@gmail.com, ORCID 0000-0002-8525-7096
^2*Каф. «Гидравлика и водоснабжение», Днепропетровский национальный университет железнодорожного
транспорта имени академика В. Лазаряна, ул. Лазаряна, 2, Днипро, Украина, 49010, тел. +38 (056) 273 15 09,
эл. почта water.supply.treatment@gmail.com, ORCID 0000-0002-1531-7882
^3*Каф. «Гидравлика и водоснабжение», Днепропетровский национальный университет железнодорожного
транспорта имени академика В. Лазаряна, ул. Лазаряна, 2, Днипро, Украина, 49010, тел. +38 (056) 273 15 09,
эл. почта water.supply.treatment@gmail.com, ORCID 0000-0003-3057-9204

СНИЖЕНИЕ КОНЦЕНТРАЦИИ АММИАКА В АТМОСФЕРЕ

ПРИ ЕГО ВНЕЗАПНОЙ ЭМИССИИ

Цель. Целью данной работы является построение численной модели, которая позволяет рассчитывать эффективность применения нейтрализатора для снижения концентраций аммиака в атмосфере в случае его внезапного выброса на территории аммиачной насосной станции. Качествами построенной модели должны быть быстрый расчет, учет метеорологических параметров и зданий, что расположены вблизи источника выброса аммиака и нейтрализатора. Методика. Разработанная модель основана на уравнении для потенциального потока и уравнении дисперсии примеси в атмосфере. Для моделирования химического взаимодействия между аммиаком и нейтрализатором используется уравнение стехиометрии. Уравнение потенциального потока употребляется для расчета скорости ветра между зданиями. Для решения уравнения потенциального течения применяется неявная разностная схема Самарского. Для численного решения уравнения массопереноса используется попеременно-треугольная разностная схема, а для численного интегрирования – прямоугольная разностная сетка. Метод маркирования («метод маркеров») применяется для создания вида расчетной области. Эмиссия аммиака моделируется с использованием дельта функции Дирака для точечного источника. Результаты. Разработанная численная модель относится к классу «диагностические модели». Эта модель учитывает основные физические факторы, влияющие на процесс рассеивания аммиака и нейтрализатора, а также влияние зданий на дисперсию примеси. На основе разработанных численных моделей был проведен вычислительный эксперимент по оценке эффективности применения нейтрализатора для снижения концентраций аммиака в атмосфере в случае его внезапного выброса на аммиачной насосной станции. Научная новизна. Разработанная численная модель позволяет рассчитать структуру потока между зданиями и оценить эффективность подачи нейтрализатора для снижения загрязнения воздуха в случае незапланированного выделения аммиака. Практическая значимость. Модель позволяет быстро рассчитывать эффективность подачи нейтрализатора для снижения негативного влияния эмиссии аммиака на людей и окружающую среду.

Ключевые слова: загрязнение атмосферы; выброс аммиака; нейтрализация; численное моделирование

REFERENCES

1. Biliaiev, M. M., Berlov, A. V., & Mashikhina, P. B. (2014). Modelirovaniye nestatsionarnykh protsessov avariynogo zagryazneniya atmosfery [Monograph]. Dnipropetrovsk: Aktsent PP.

2. Berlyand, M. Y. (1985). Prognoz i regulirovaniye zagryazneniya atmosfery. Leningrad: Gidrometeoizdat.

3. Bruyatskiy, Y. V. (2000). Teoriya atmosfernoy diffuzii radioaktivnykh vybrosov. Kyiv: Institut gidromekhaniki NAN Ukrainy.

4. Zakaznov, V. F., & Kursheva, L. A. (1985). Rasprostraneniye ammiaka pri razgermetizatsii ammiakoprovoda, emkostey. In Issledovaniya i razrabotki po sozdaniyu magistralnykh ammiakoprovodov i skladov zhidkogo ammiaka. Moscow: The State Research and Design Institute of the Nitric Industry and Organic Synthesis Products.

5. Marchuk, G. I. (1982). Matematicheskoye modelirovaniye v probleme okruzhayushchey sredy. Moscow: Nauka.

6. Tsykalo, A. L., Strizhevskiy, I. I., & Baglet, A. D. (1982). Azotnaya promyshlennost: Ispareniye i rasseivaniye ammiaka pri ego razlivakh i utechkakh. Moscow: NIITEKHIM.

7. Zgurovskiy, M. Z., Skopetskiy, V. V., Khrushch, V. K., & Biliaiev M. M. (1997). Chislennoye modelirovaniye rasprostraneniya zagryazneniya v okruzhayushchey srede. Kyiv: Naukova dumka.

8. Biliaiev, M. M. & Amelina, L.V. (2017). Numeric Simulation of Air Pollution in Case of Unplanned Ammonia Release. Science and Transport Progress, 3(96), 7-14. doi:10.15802/stp2017/104142

9. Biliaiev, M. M., Amelina, L.V., & Kharitonov, M. M. (2013). Numerical simulation of the atmosphere pollution after accident at the “Tolliaty-Odessa” ammonia pipe. NATO Science for Peace and Security Series C: Environmental Security, 391-395. doi:10.1007/978-94-007-5577-2_66

10. Daly, A., Zanetti, P., & Jennings, M. (2013). Accident reconstruction and plume modeling of an unplanned ammonia release. Air Pollution XXI. WIT Transactions on Ecology and the Environment, 174, 3-13.
    doi:10.2495/AIR130011

11. SWCA Environmental Consultants. (2010). Dispersion Modeling of Hydrogen Sulfide at Cimarex Rands Butte Project Using ALOHA. Wyoming.

12. Janos, T., Gorliczay, E., & Borbely, J. (2016). Atmostheric spreading model for ammonia released from the poultry house. Ecotoxicologie, Zootehnie si Tehnologii de Industrie Alimentara, XV/B, 331-337. Retrieved from http://protmed.uoradea.ro/facultate/publicatii/ecotox_zooteh_ind_alim/2016B/ipa/17%20Tamas_Janos.pdf

13. Mellsen, S. B. (1989). A Fortran Program for Calculating Chemical Hazards Using the NATO Stanag 2103/ATP-45 Algorithm: Suffield memorandum 1275. Alberta: Defence Research Establishment Suffield.
    Retrieved from http://oai.dtic.mil/oai/oai?verb=getRecord&metadataPrefix=html&identifier=ADA214763

14. Zavila, O., Dobeš, P., Dlabka, J., & Bitta, J. (2015). The analysis of the use of mathematical modeling for emergency planning purposes. Bezpecnostni vyzkum, 2. Retrieved from http://www.population-protection.eu/prilohy/casopis/30/213.pdf

15. Warner, T., Benda, P., Swerdlin, S., Knievel, J., Copeland, J., Crook, A., …, & Weil, J. (2007). The Pentagon Shield Field Program: Toward Critical Infrastructure Protection. Bulletin of the American Meteorological Society, 88(2), 167-176. doi:10.1175/BAMS-88-2-167

Prof. S. A. Pichugov, Dr. Sc. in Phys.-and-Math. (Ukraine); Prof. S. Z. Polishchuk, D. Sc. (Tech.), (Ukraine) recommended this article to be published

Received: March 31, 2017

Accessed: July 05, 2017



 L. V. Amelina, N. N. Biliaiev, P. B. Mashichina, 2017